Low-tech ways of visualizing multivariable and vector calculus

Question

One way, which is the most obvious, is do sketches of 3d shapes that tend to be the ones that we can all draw (like rectangle, cone, cylinder, sphere, etc.)

Another way is by analogy so even if we can't really sketch the graph to any kind of precision or accuracy, there is enough analogy to "fill in the blanks" of what the sketch is lacking. Like how a skier might who wants to do down the hill fastest will go along the gradient.

Akin to that above one is real-life pictures or diagram like contour maps for showing elevations, or heat maps. These could show 3d functions on a 2d surface.

There might be a way of 2d-3d analogy in how, say, finding the area underneath is curve via rectangles is like finding the volume under a surface via rectangular prisms.

---

What are ways you show "visuals" without having to type up equations that will be graphed by a computer program?

Answer 1

I don't know if this is what you're looking for exactly, but I've run forms of this activity a few times when introducing partial/directional derivatives.

Supply the class with materials: square grids printed on paper, scissors, compass (with attached pencil), ruler and protractor [Sometimes I forego the compass and just provide appropriately-sized circles printed on paper.]

Give them the following directions:

1. Measure the length of three squares from the grid, and multiply this by $\sqrt{2}$. Call your result $r$.

2. Use your compass to draw a circle with radius $r$, and cut it out with scissors.

3. Mark a small point on the perimeter of the circle, labeling it #1.

4. With a protractor, find and label another point #2 on the perimeter which is $105.5^{\circ}$ away from point #1 (with the center of the circle as the vertex of that angle).

5. Cut a straight line from mark #1 to the center of the circle.

6. Curve the paper so mark #1 coincides with mark #2.

7. Tape your surface together (inside and out), being sure not to crease or otherwise deform the surface.

8. Draw an x-axis and a y-axis on the grid paper provided, so the origin $(0,0)$ is in the center of the grid.

9. Place your surface on the grid so the point is upward, directly above the point $(0,0)$ on the plane.

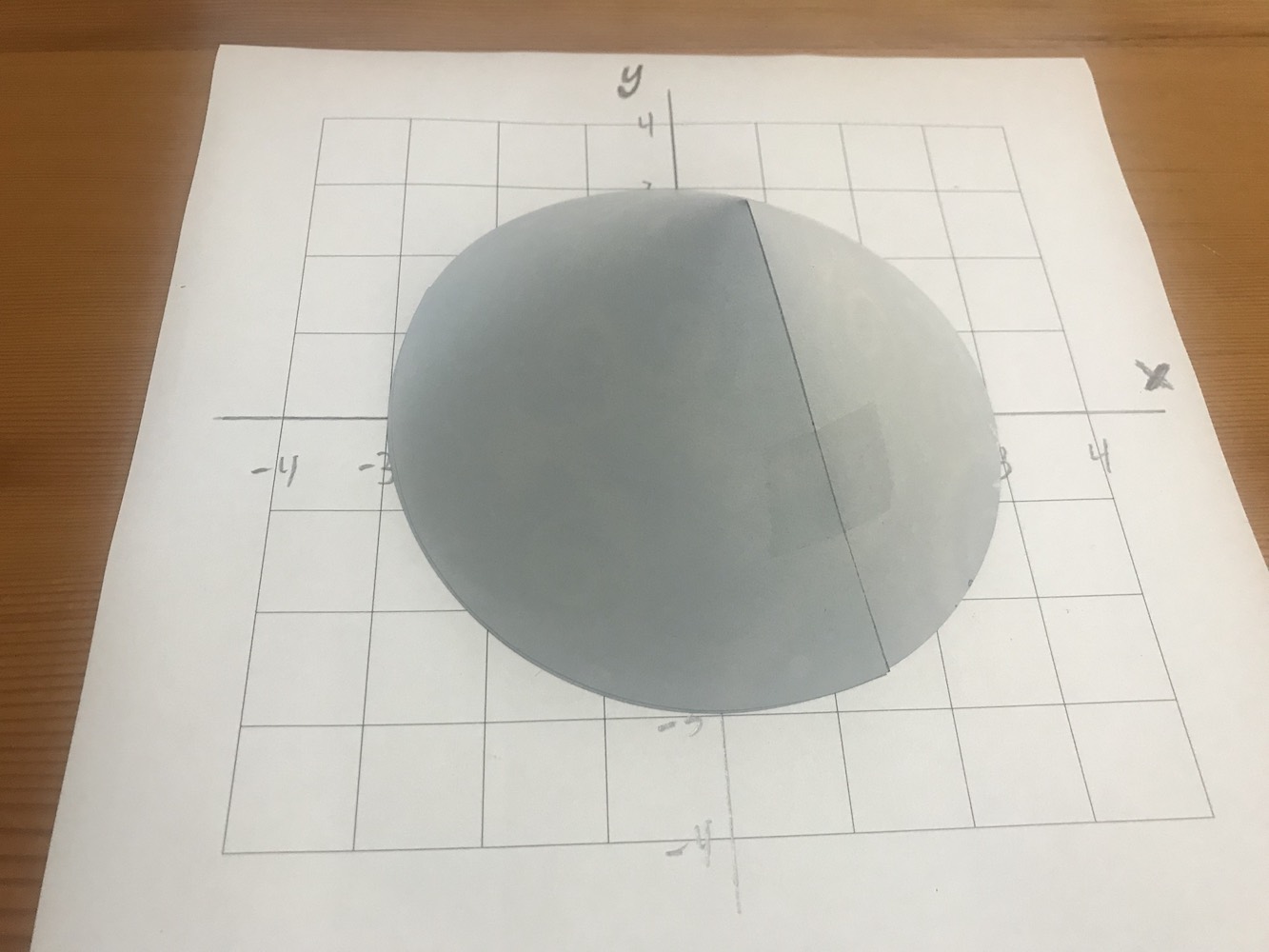
Congratulations! You have just made the surface $z=f(x,y)=3-\sqrt{x^2+y^2}$ which is part of (the bottom half of) a right, circular cone.

Then a series of questions, such as:

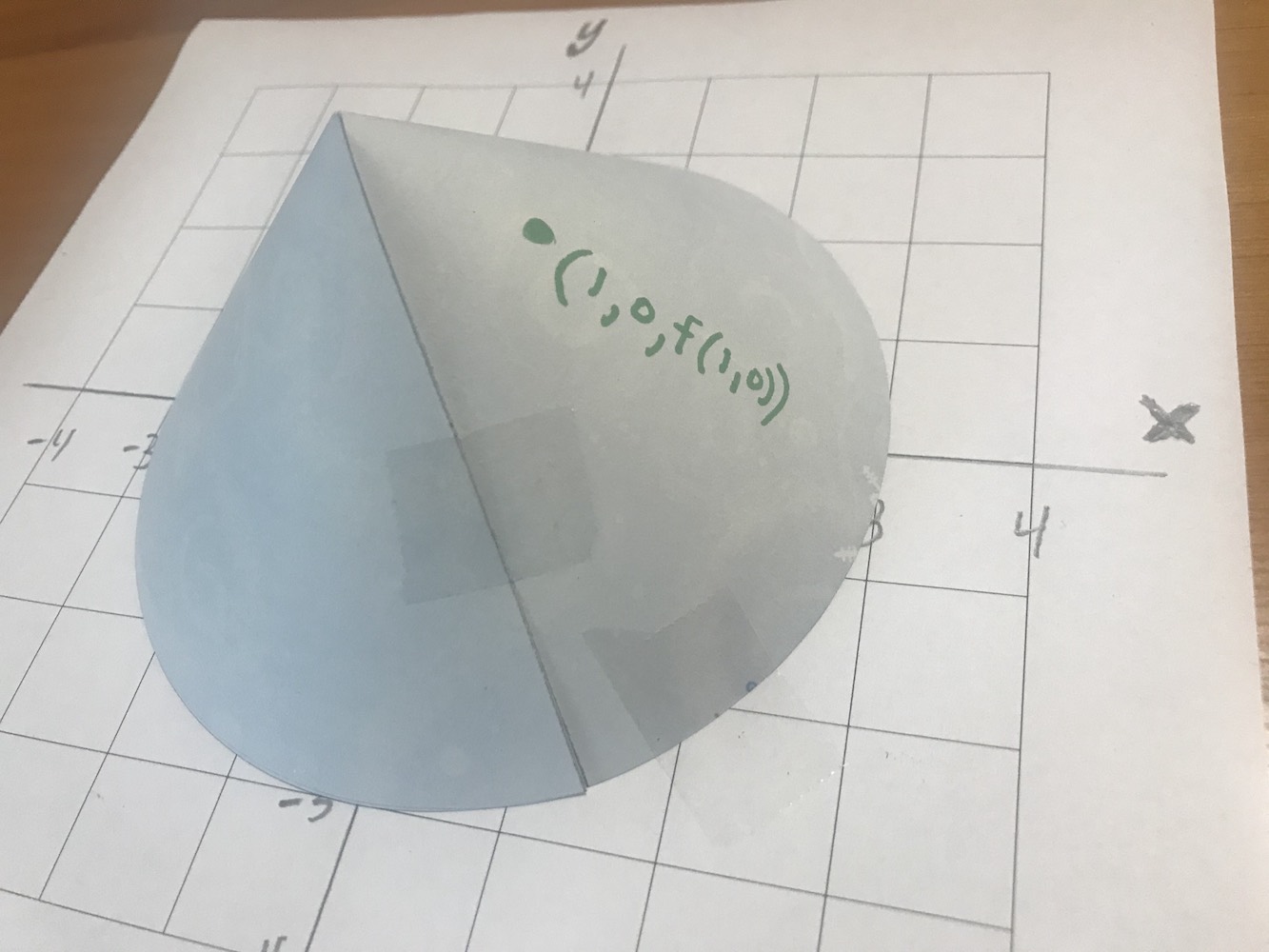
(a) What are the coordinates of the point of the cone?

(b) If you walked straight up the side of the cone, what would be the slope?

(c) What is $f(1,0)$?

(d) Plot the point $(1,0,f(1,0))$ on your surface.

(e) From that point, if you kept your x-value fixed but moved slightly in the y-direction, what would you experience as you moved along the surface of the cone? Approximate your slope at that point.

Repeat parts (c)-(e) with the point $(1,1)$ and $(1,2)$.

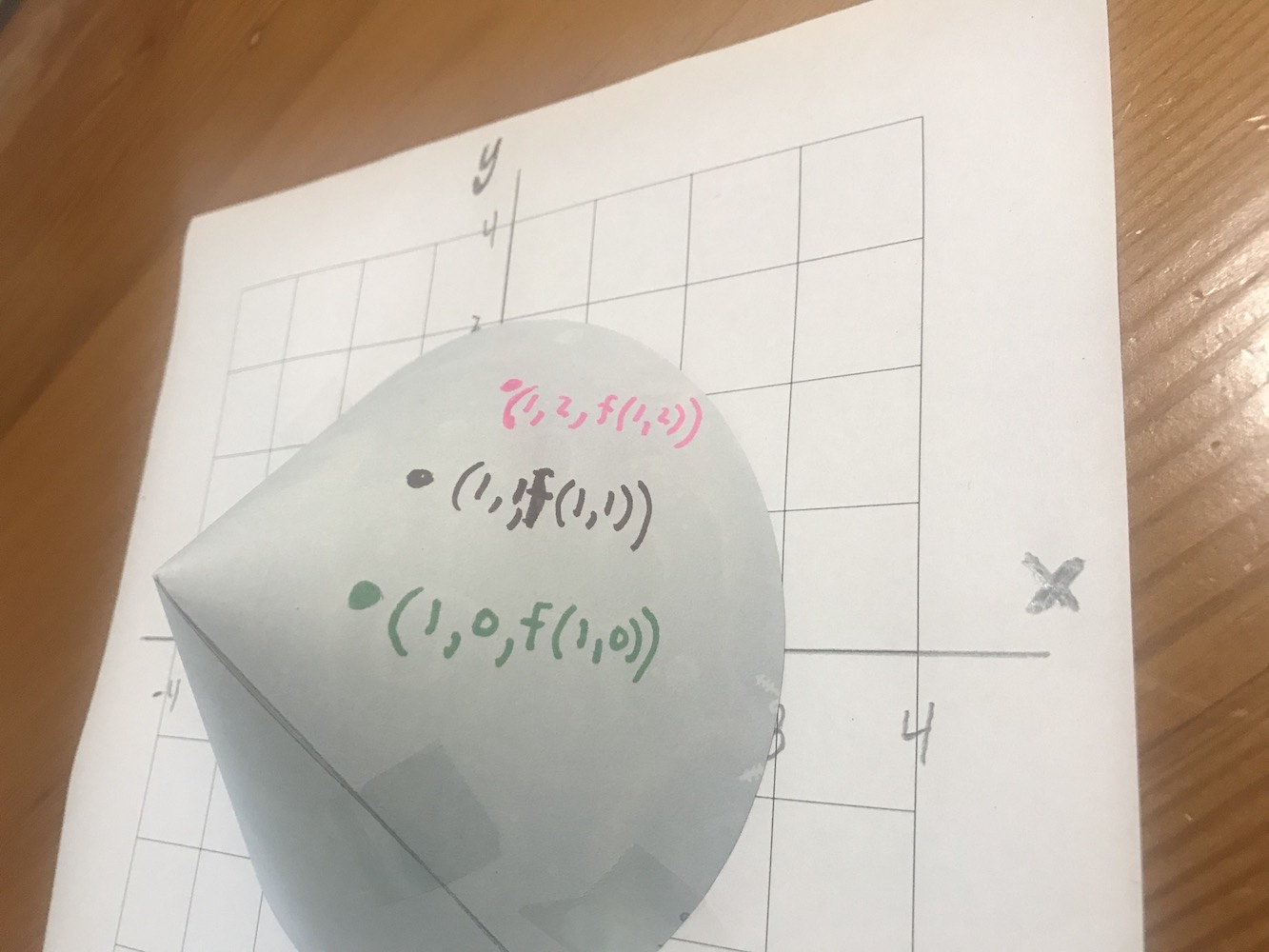
Compare your approximations of the slope at each of those points.

Now, Let's try this out algebraically. Since we're leaving the x value fixed, take the derivative of the function $z=f(x,y)=3-\sqrt{x^2+y^2}$ by imagining that $x$ is a constant. [That is, just differentiate with respect to $y$.]

Etc. [Connect the dots to make a slice. What is this curve? How can you see the "slopes" on this curve? Repeat questions where we move in x and not in y.]

---

It just happens to be the simplest surface I can imagine students building with any level of precision for measurement or approximation of slopes. It might be the first time in years that they have used a compass or a protractor, and it gets them handling a surface they built.

I'm sure there are other better examples of this kind of thing, but it has served me well for introductory purposes. There's probably a book of these types of activities, but this one just occurred to me to try once when I felt my students were too disconnected from the physicality of the topic.