4
$\begingroup$

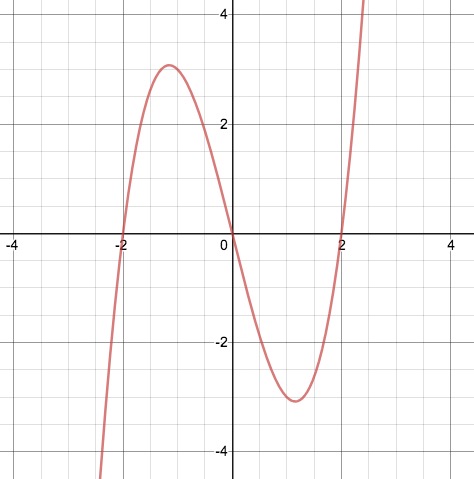
Let me use this example,

Solve $x^3-4x>0$

After factorization, we have $$(x+2)x(x-2)>0$$, in order to have product of several numbers positive, even(0,2,4,...) of them have to be negative numbers. In this case, none of them is negative or two of them are negative, we can conclude $\displaystyle x\in(-2,0)\cup (2,\infty)$.

However I don't find the above approach too popular, more often than not, the following approach is what I see people doing,

Start by solving equality, $x^3-4x=0$, same factorization gives us $x=-2,0,2$, therefore we make partition $\mathbb{R} \backslash \{-2,0,2\}=(-\infty,-2)\cup(-2,0)\cup (0,2)\cup (2,\infty)$. In order to see whether $f(x)=x^3-4x$ is positive or negative on each interval, plug in a number in each interval. For example $f(-10)=-960<0$ so $f$ is negative on $(-\infty,-2)$, $f(-1)=3>0$ so $f$ is positive on $(-2,0)$,etc. In the end you get the same conclusion $\displaystyle x\in(-2,0)\cup (2,\infty)$.

Although perfectly valid, there are two main reasons I don't like the second approach,

  1. In order to solve equality, we still need to factorize the left hand side. Once we have the factorization, it's much easier to check positive/negative than to compute values of function.

  2. In order to conclude $f(x)>0,\forall x\in I$(where $f(x)\neq 0$ on interval $I$) from $\exists c\in I, f(c)>0$, $f$ needs to satisfy intermediate value property(all continuous functions have it by IVT). I don't see why we need any result from calculus to solve any precalculus problem.

Therefore my question is, despite the reasons I list, why is the second approach more popular, not only among students but also instructors and TAs (of calculus/precalculus class)? There should be some advantages of this method that I don't know about. Any insight is welcome.

Improve this question
$\endgroup$
5
  • 4
    $\begingroup$ I really don't expect it to be for any reason other than the IVT. $\endgroup$
    – Git Gud
    Commented May 26, 2016 at 10:50
  • 4
    $\begingroup$ It would seem that this is an extension of how linear inequalities are taught in high school. Students prefer to continue a method that they know rather than learn a new method. $\endgroup$
    – Amy B
    Commented May 26, 2016 at 13:04
  • 5
    $\begingroup$ Students are often poor at reasoning. Plugging in a value may feel easier to them than analyzing how many factors are negative. $\endgroup$
    – Sue VanHattum ♦
    Commented May 26, 2016 at 13:47
  • 1
    $\begingroup$ FYI, in high school we used your first method, and I believe this method was the only one described in our textbook -- Dolciani's Modern Algebra and Trigonometry. I don't know where I learned about the other method, but it was after high school, and when I learned the other method we didn't plug numbers into the intervals but rather determined the sign by algebraic considerations (e.g. factor(s) corresponding to the interval) or by graphical considerations (e.g. $(e^x - 2)\ln x$ is negative just to the right of $0$ and positive for large $x).$ $\endgroup$
    – Dave L Renfro
    Commented May 27, 2016 at 14:49
  • 4
    $\begingroup$ Also, an obvious drawback to the first method is that the possibilities needed to examine grow at an exponential rate as a function of the number $n$ of factors. For example, with $6$ factors there are $2^6 = 64$ sign permutations, $32$ of which lead to a positive product and $32$ of which lead to a negative product. For the second method, the number of possibilities needed to examine only grows linearly (in fact, not something like $200n$ or $37n,$ but just $n).$ $\endgroup$
    – Dave L Renfro
    Commented May 27, 2016 at 14:53

2 Answers 2

Reset to default
11
$\begingroup$

I, personally, wouldn't solve this specific inequality in either fashion; I would instead decide the sign on one of the four intervals — e.g. by plugging in $x = +\infty$ — and recognize that each of the roots is a simple crossing so that the sign alternates between adjacent intervals.

In the precalculus courses at my university, yet another method was taught: rather than having to reason out what possible combinations of signs there are and fill in the detail you glossed over with "we can conclude", they construct a table $$\begin{matrix} & (-\infty, -2) & (-2, 0) & (0, 2) & (2, \infty) \\ x-2 & - & - & - & + \\ x & - & - & + & + \\ x+2 & - & + & + & + \end{matrix} $$ so they can just tally up the signs.

Ultimately, the important thing is that students have a method that works, and furthermore can be executed in a systematic and straightforward fashion on problems complicated enough that they can't just see the answer directly.

Despite your assertion, you do not need to factor to do sign analysis. Obtaining roots can be faster, and factorization could well be rather complicated. For example, solving $x^8 - 1 > 0$.

Your second point looks like a non sequitur; I'm going to assume your objection is more about using methods whose underpinnings use results you haven't proven... but when the point is to have a method that students can use and understand, the theoretical underpinnings aren't really relevant unless they get in the way of students' ability to use and understand.

If you're really interested in full rigor, there are worse things to worry about, such as the existence of square roots, or the definitions and properties of transcendental functions.

TL;DR:

  • there are numerous approaches
  • none seem dramatically superior to the others
  • an advantage of the second versus first approaches of your post is that the algorithm is explicit: it tells students how to conclude
Improve this answer
$\endgroup$
2
  • 4
    $\begingroup$ "Ultimately, the important thing is that students have a method that works" — I disagree. The important thing is that students have a method they understand, which includes understanding why each step works and why it's a reasonable thing to do. $\endgroup$
    – Daniel Hast
    Commented May 27, 2016 at 12:44
  • 4
    $\begingroup$ I used to teach this method, and I agree you don't have to factor as much as identify all the zeros and other relevant points. I posted several detailed examples in this 29 October 2006 sci.math post. $\endgroup$
    – Dave L Renfro
    Commented May 27, 2016 at 14:34
0
$\begingroup$

As Dave notes, the zeroes are key. Factoring is one method to arrive at that. I deal with students that benefit from visual examples where appropriate. To that end, I encourage these students to sketch a quick graph.

enter image description here

Presumably, when they are given this particular question, the zeroes are enough for them to sketch the graph in a way that makes the inequality solution clear. By this, I mean that they won't use these X-Axis intercepts but produce a flipped graph. And 'sketch' means that the local maxima/minima don't really matter, as far as accuracy is concerned. If the graph shows (-1,2) as the local max, no harm. No impact to the question as asked.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.