20
$\begingroup$

The slope-intercept form of the equation of a line is often presented in textbooks (in the US) as

$$y = mx + b\,,$$

where $m$ is the slope of the line and $b$ is the $y$-intercept. How did $m$ and $b$ become the standard variables used for the slope and $y$-intercept? What should we tell students $m$ and $b$ represent to help them make sense of the roles of $m$ and $b$ in that equation?

Improve this question
$\endgroup$
9
  • 12
    $\begingroup$ In the old newsgroup sci.math, we would get this question like clockwork every fall. The guess that $m$ is for the French monter appears to be without basis. See mathforum.org/library/drmath/view/52477.html and funtrivia.com/askft/question11912.html and madmath.com/2015/12/why-m-for-slope.html $\endgroup$
    – Gerald Edgar
    Commented Aug 29, 2017 at 21:56
  • 5
    $\begingroup$ Somewhere I heard: $b$ for $b$egin and $m$ for $m$ove. $\endgroup$
    – Joseph O'Rourke
    Commented Aug 29, 2017 at 23:09
  • 3
    $\begingroup$ The symbol $a$ is often used for the $x$-intercept and other values related to $x$, while $b$ is for the $y$-intercept and other values related to $y$. Those letters and their order makes sense. Examples are the intercept-intercept form of the line $\dfrac xa+\dfrac yb=1$ and the axis-aligned ellipse equation $\dfrac{(x-h)^2}{a^2}+\dfrac{(y-k)^2}{b^2}=1$. This explains $b$ but does not explain $m$. $\endgroup$
    – Rory Daulton
    Commented Aug 30, 2017 at 0:17
  • 3
    $\begingroup$ "m" for "marginal" might be helpful for students who have been exposed to business ideas. Linear cost functions look like $C(x) = mx+b$ with $m$ the marginal cost (and "baseline" for "b" works well for the other parameter) $\endgroup$
    – John Coleman
    Commented Sep 1, 2017 at 15:05
  • 3
    $\begingroup$ I must admit that I'm not sure what students gain mathematically from the answer to the question. The letters that we use are completely irrelevant to the math. It might be an interesting historical question that is worth asking, but I'm concerned that if we attach artificial meaning to the letters, students will focus on that instead of the underlying mathematics. $\endgroup$
    – rnrstopstraffic
    Commented Sep 9, 2017 at 23:15

3 Answers 3

Reset to default
27
$\begingroup$

This is also borderline not-an-answer, but it might be a nice broadening of your students' worldview to know that the "$m$" and the "$b$" are not universally accepted. Showing them this map (even though I do not know its original source, so it may not be accurate in its details) could help their thinking out a bit:

Map of linear equation letters

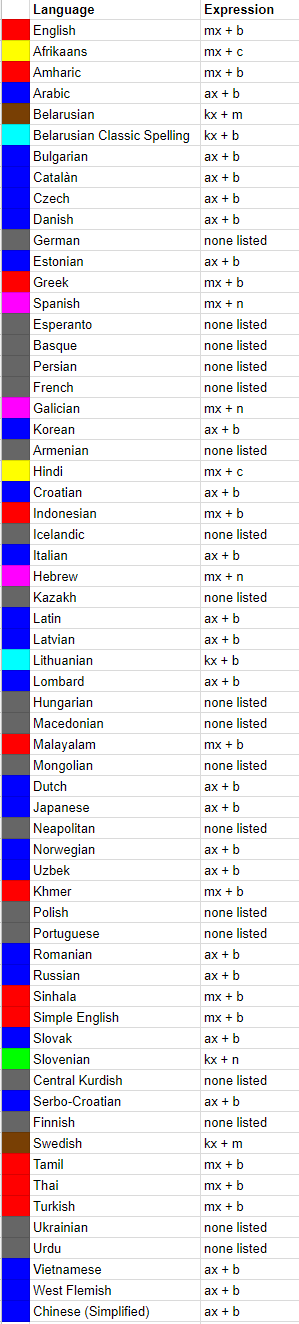
Substantial edit: I now no longer believe that the above map has very many if any of its details correct, although the general sentiment ("$mx + b$ is not a worldwide convention") is still correct. I went through all the languages of Wikipedia that had an article for Linear_equation and found the first mention of "[variable]x + [variable]" in the article. I kept the same absurd color-coding as the map, even though most of my results disagree with the map, even in Europe where languages map more precisely to countries.

First "$mx+b$-type expression" in each language's Wikipedia page for Linear_equation

Linear Equation by Language in Wikipedia

So anyway, one way or another, even though the map is probably-wrong, there definitely isn't much worldwide agreement on which variables to use in which slot.

Improve this answer
$\endgroup$
6
  • 1
    $\begingroup$ Yeah, finding a good source for this map is tough. I found this thread on Reddit, and this footnote on mathisfun.com, but neither provide a reference (and many of the comments on the Reddit thread seem to disagree). $\endgroup$
    – Mike Pierce
    Commented Aug 30, 2017 at 15:17
  • 4
    $\begingroup$ Even in the United States it isn't universal above a certain level. For example, $m$ is seldom used in stats books when they discuss simple linear regression. $\endgroup$
    – John Coleman
    Commented Aug 30, 2017 at 15:20
  • $\begingroup$ @MikePierce Thanks for the link to the reddit thread; I went ahead and just did the Wikipedia research myself since those links are indeed the best sources for the map and neither of them are any good. The map is probably bogus and I've tried to update my answer accordingly. $\endgroup$
    – Chris Cunningham
    Commented Aug 31, 2017 at 23:30
  • $\begingroup$ In Italy, I'd say that $mx+q$ (we use $q$ instead of $b$) is more widespread than $ax+b$. $\endgroup$
    – Massimo Ortolano
    Commented Sep 2, 2017 at 15:41
  • 3
    $\begingroup$ Ya, I'd say that I have been unable to find a good way to make any good conclusions about any of the details; the only thing constant I was able to find was that it is definitely not $mx + b$ worldwide. $\endgroup$
    – Chris Cunningham
    Commented Sep 3, 2017 at 1:09
11
$\begingroup$

I taught low level algebra for a bit and those students really struggled with knowing what variables were and that they actually stood for numbers. They would see $y=mx+b$ and just not have any idea what it meant or stood for. I've heard the "monter" explanation before, but the students didn't find it helpful (they didn't speak french after all...). What I ended up doing, is replacing a lot of the variables in the class with blanks. So I would write $y=\_\_\,x + \_\_$ and then ask what went in each blank and they would respond with "slope" and "intercept" so I would write $$y=(\text{slope})\,x+(y\text{-intercept})$$ and they seemed to follow that a bit better since they themselves came up with the words to use and didn't have to memorize "m for slope" and "b for y-intercept" since those seem more disconnected.

The "b" for "begin" and "m" for "move" explanation seems like it would have worked for this level of student, I wish I could have tried that.

Improve this answer
$\endgroup$
7
$\begingroup$

I would suggest not focusing on the notation "m" and "b" and just explain why they are different in terms of their relationships to x and y.

What's in a name? That which we call a rose by any other name would smell as sweet.

The slope has to interact with `x' because it is describing the relationship between how much y changes when x changes. If x changes by a lot, the slope passes this information on to y.

The intercept sits out there by itself because it is an absolute location on the line. If x changes by a lot, the y-intercept is still just the y-intercept. It doesn't care how x is changing.

Improve this answer
$\endgroup$
0

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.