Different ways to multiply decimals

Question

I am an undergraduate secondary math education major. In $2$ weeks I have to give a Number Talk in my math ed class on the problem "$3.9$ times $7.5$". I need to come up with as many different solution methods as possible.

Here is what I have come up with so far:

1. The most common way: multiply the two numbers "vertically", ignoring the decimal, to get $2925$: \begin{array} {}\hfill {}^6{}^439\\ \hfill \times\ 75 \\\hline \hfill {}^1 195 \\ \hfill +\ 273\phantom{0} \\\hline \hfill 2925 \end{array} Since there are two numbers that are to the right of the decimal, place the decimal after the $9$ to get the answer $29.25$.

2. Write both numbers as improper fractions: $$3.9= \dfrac{39}{10}$$ and $$7.5=\dfrac{75}{10}$$Then multiply $$\dfrac{39}{10}\cdot\dfrac{75}{10}$$ to get $\dfrac{2925}{100}$ which simplifies to 29.25.

3. Use lattice multiplication. This is a very uncommon method that I doubt the students will use, and I need to review it myself before I consider it.

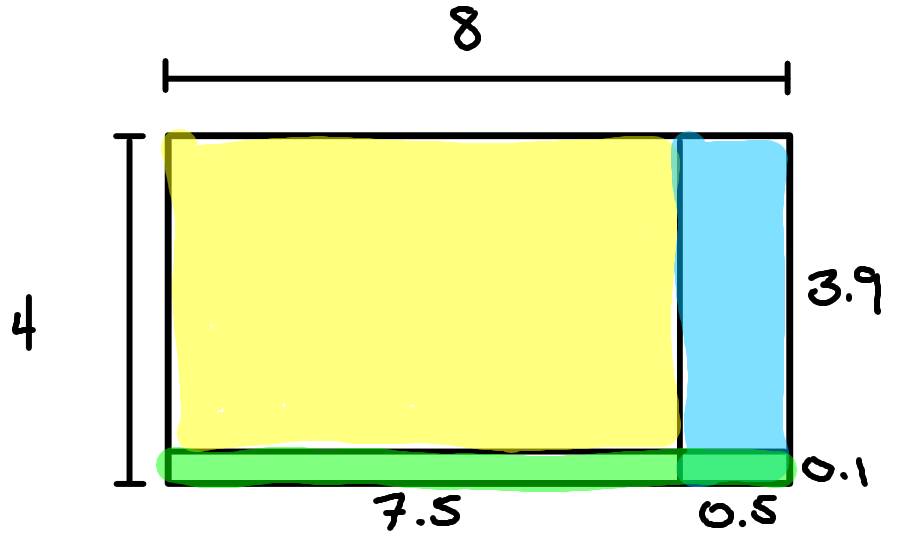
4. Since $3.9$ is very close to $4$, we could instead do $4\cdot7.5=30$ and then subtract $0.1\cdot7.5=0.75$ to get $30 - 0.75=29.25$

5. Similarly, since $7.5$ rounds up to $8$, we can do $3.9\cdot 8=31.2$ and then subtract .$5\cdot 3.9=1.95$ to get $31.2-1.95=29.25$

Are there any other possible methods the students might use? (Note: they are junior college math ed students.) Thanks!

Answer 1

There's a fun method which I've seen referred to as Russian peasant multiplication or ancient Egyptian multiplication. (I don't know if these names have a historical basis.)

If you think about how the algorithm works behind the scenes, it's essentially multiplying the two numbers in base 2, so it could have more than one pedagogical use.

This technique is for integers; you'll still need to handle the placement of the decimal point separately, as usual. (You could actually keep the decimal points in column 2, but you'd still have to adjust the placement of the decimal point in the product, so it's probably easier just to work with integers throughout.)

Here's the method:

(a) Put each number at the top of a column.

(b) In column 1, divide by $2$ repeatedly, ignoring any remainders, until you reach $1.$

(c) In column 2, multiply by $2$ repeatedly. Stop when column 2 is the same height as column 1.

(d) Cross off every row where the number in column 1 is even.

(e) Add the numbers in column 2 that you haven't crossed off. That's the product.

---

Here's your example of $39\times75,$ worked out using this method:

39     75
19    150
 9    300
 4    600
 2   1200
 1   2400
     ----
     2925

Answer 2

Combining your last two methods: $3.9*7.5 = 4*8 - 0.1*8 - 4 * 0.5 + 0.1*0.5$, which can be thought of as computing the area of the big rectangle below, cutting off the two extra strips along the edge, then adding back in a copy of the corner piece since you have removed it twice, instead of once.

Answer 3

Estimate the result. Multiply the numbers without paying attention to the decimal point. Place the decimal point so that the result is close to the estimate.

Answer 4

Write out the numbers using the "expanded form", then use the distributive property of multiplication over addition to evaluate the product. Consistently using powers of $10$ throughout, this is \begin{align} 3.9 \cdot 7.5 &= \left(\color{red}{3}\cdot 10^\color{red}{0} + \color{blue}{9}\cdot 10^\color{blue}{-1} \right) \left( \color{orange}{7}\cdot 10^\color{orange}{0} + \color{green}{5}\cdot 10^\color{green}{-1} \right) \\ &= \left(\color{red}{3}\cdot \color{orange}{7} \cdot 10^{\color{red}{0}+\color{orange}{0}} \right) + \left( \color{red}{3}\cdot \color{green}{5}\cdot 10^{\color{red}{0}+(\color{green}{-1})} \right) \\ &\qquad\qquad+ \left( \color{blue}{9}\cdot \color{orange}{7}\cdot 10^{\color{blue}{-1}+\color{orange}{0}} \right) + \left( \color{blue}{9}\cdot \color{green}{5}\cdot 10^{\color{blue}{-1}+(\color{green}{-1})} \right) \\ &= \left( 21\cdot 10^{0} \right) + \left( 15\cdot 10 ^{-1}\right) + \left( 63 \cdot 10^{-1} \right) + \left( 45 \cdot 10^{-2} \right) \tag{$\ast$} \\ &= \left( 21\cdot 10^{0} \right) + \left( 1.5\cdot 10 ^{0}\right) + \left( 6.3 \cdot 10^{0} \right) + \left( 0.45 \cdot 10^{0} \right) \tag{$\ast$} \\ &= 29.25 \cdot 10^{0} \\ &= 29.25. \end{align}

There are some advantages to this approach

1. It emphasizes the place-value notation—when we write a number, each digit represents some power of ten. This would be an appropriate thing to emphasize in late elementary school in the US (it hits on a Grade 5 Common Core Standard (CCS); there is also an important connection to scientific notation here).

2. It emphasizes the distributive property of multiplication over addition. This is part of the Grade 6 CCS, and is an excellent way to practice skills that are important later in algebra.

3. We don't have to track the decimal point. Of course, this comes at a cost, as we have to keep track of powers of ten. TANSTAAFL.

However, there is a lot of extra notation running around, particularly at the two marked lines. An alternative, though similar, approach would be to skip the exponential notation and work with the decimals:

\begin{align} 3.9\cdot 7.5 &= (\color{red}{3} + \color{blue}{0.9}) ( \color{orange}{7} + \color{green}{0.5} ) \\ &= \left( \color{red}{3} \cdot \color{orange}{7} \right) + \left( \color{red}{3} \cdot \color{green}{0.5} \right) + \left( \color{blue}{0.9} \cdot \color{orange}{7} \right) + \left( \color{blue}{0.9} \cdot \color{green}{0.5} \right) \\ &= 21 + 1.5 + 6.3 + 0.45 \\ &= 29.25. \end{align}

Answer 5

Trachtenberg methods: this depends on calculating the answer one digit at a time, starting at the units. Maybe this is what you call "lattice". This was in vogue around 1960 -- maybe it gets rediscovered every generation.

Units can only come from 9 x 5. Write down 5, carry 4.

Tens can come from 3 x 5 and 9 x 7, i.e. 78, plus carry. Write down 2, carry 8.

Hundreds come from 3 x 7 plus carry, so 29. Write 9, carry 2.

Thousands leaves only the carry. Write down 2.

On paper, it is customary to put a dot over each digit of the top number as it is used, until you get proficient.

There are specific methods for particular multipliers, but they are special cases of this method.

Answer 6

This slide rule is set to show the same multiplication problem, $3.9\times7.5=29.25.$ (Click on the image for a larger view showing enough detail to see what's going on.)

This is equivalent to computing the product by adding logarithms, which is yet another way to do it:

$$3.9\times7.5=e^{\ln 3.9 + \ln 7.5}$$

Answer 7

Pull out your slide rule. Align the second "1" on the C scale with the "3.9" on the D scale. Move the slide to the "7.5" on the C scale and read "2.92" off the D scale. Observe that there are two decimal places in the problem, and write down the answer as approximately 29.2.

(If you want more precision, get a bigger slide rule. A standard 10-inch rule is only good for about three and a half digits.)

Answer 8

You can do questions like this in your head pretty easily which is a great skill to teach students. Since $3.9$ is close to $4$, and $7.5$ is easy to add to itself. So $3.9\times 7.5 = 4\times 7.5 - 0.1\times7.5$. $4\times 7.5$ is just doubling it twice. $7.5\times 2=15$, and $15\times 2=30$. Then subtract $0.1\times 7.5=0.75$. So that's $29.25$. A similar process can be used to do most questions like this in your head.

Answer 9

I would do $$3.9\times7.5=\frac{3.9\times30}4=\frac{39\times3}4=\frac{(40-1)\times3}4=\frac{40\times 3}4-\frac34=30-\frac34.$$

Answer 10

Consider the following solution. \begin{align*}7.5\times3.9&=(0.1\times75)\times(0.1\times39)\\\\&=0.01\times75\times39\\\\&=75\%\,\,\text{of}\,\,39\quad(\text{as}\,\,\%\,\,\text{means}\,\,1/100=0.01)\tag{1}\\\\&=\frac34\times39\quad\small{\left[=\frac{4-1}4\times39=\left(\frac44-\frac14\right)\times39\right]}\tag2\\\\&=\left(1-\frac14\right)\times39\quad\small{\left[=1\times39-\frac14\times39\right]}\\\\&=39-\frac{39}4\\\\&=39-\frac{36+3}4\quad\small{\left[=39-\left(\frac{36}4+\frac34\right)\right]}\\\\&=39-\left(9+\frac34\right)\\\\&=39-9-\frac34\\\\&=30-0.75\\\\&=29.25\end{align*} $(1)$ As an aside, it may be of interest to mention that $75\%\times39=39\%\times75$.

$(2)$ Comparing the length of this solution to Martin's, it shows the importance of choosing whether to express $3=4-1$ (as in this answer), or $39=40-1$ which yields a quicker solution.

Answer 11

Do it in slices.

We teach young kids that multiplication is just repeated addition. What's 5x4? It's just 5, added 4 times.

So do the same with the decimal numbers. 3.9 x 7.5. First, let's use 3.9 added 7 times. You can't add it an eighth time, because that's over 7.5 - we only need to add it 0.5 times now. So how would you add it 0.5 times? Divide it by 10, and add it five times. So you'd get:

3.9 + 3.9 + 3.9 + 3.9 + 3.9 + 3.9 + 3.9 + 0.39 + 0.39 + 0.39 + 0.39 + 0.39

Express Each Term As A Rational Fraction

Every non-infinite decimal value can be expressed as a rational fraction. 0.153258 is just 153258/1000000. And you can multiply two rational fractions without worrying about decimal places. Then, finally at the end, you can reduce it back into decimal form.

3.9 is 39/10. 7.5 is 15/2 (or 75/100, if you want.) Multiply those fractions together, and you get (39x15)/(10x2) - or reduced down 117/4.

Jokingly ask them to convert it to binary and let them discover the joy of floating point errors.

... hey, you asked for as many ways as possible, right?

Answer 12

$$\left\lfloor \frac{\left(x+y\right)^2}{4} \right\rfloor - \left\lfloor \frac{\left(x-y\right)^2}{4} \right\rfloor = xy.$$

This answer uses the floor function (which essentially means to get rid of the decimal). You can then quickly consult a table to find 0.25*x^2.

X, 0.25*x^2

1, 0.25
2, 1
3, (9/4)
4, 4
5, (25/4)
6, 9
7, (49/4)
8, 16
9, (81/4)
10, 25
11, (121/4)
12, 36
13, (169/4)
14, 49
15, (225/4)

Source: https://en.wikipedia.org/wiki/Multiplication_algorithm#Quarter_square_multiplication

Answer 13

This is similar to Xander Henderson's approach, but the calculations have been made slightly easier by using two FOIL multiplications instead of just one:

$$3.9 \times (3.8 + 3.7)$$ $$=3.9 \times 3.8 + 3.9 \cdot 3.7$$ $$=(4 - 0.1)(4-0.2)+(4-0.1)(4-0.3)$$ $$=16-0.8-0.4+0.02+16-1.2-0.4+0.03$$ $$=16+16-0.8-1.2-0.4-0.4+0.02+0.03$$ $$=(16+16)-(0.8+1.2)-(0.4+0.4)+(0.02-0.03)$$ $$=32-2-0.8+0.05$$ $$=29.2+0.05$$ $$=29.25$$