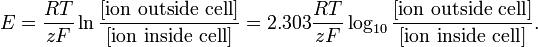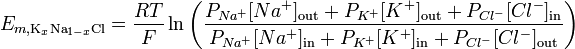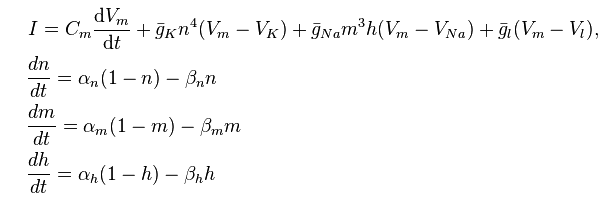I happen to have revised our calculus syllabus for first year biology majors about one year ago (in a French university, for that matter). I benefited a lot from my wife's experience as a math-friendly biologist.
The main point of the course is to get students able to deal with quantitative models. For example, my wife studied the movement of cells under various circumstances.
A common model postulates that the average distance $d$ between two
positions of a cell at times $t_0$ and $t_0+T$ is given by
$$d = \alpha T^\beta$$ where $\alpha>0$ is a speed parameter and
$\beta\in[\frac12,1]$ is a parameter that measures how the movement
fits between a Brownian motion ($\beta=\frac12$)
and a purely ballistic motion ($\beta=1$).
This simple model is a great example to show how calculus can be relevant to biology.
My first point might be specific to recent French students: first-year students are often not even proficient enough with basic algebraic manipulations to be able to do anything relevant with such a model. For example, even asking to compute how $d$ changes when $T$ is multiplied by a constant needs to now how to deal with exponents. In fact, we even had serious issues with the mere use of percentages.
One of the main point of our new calculus course is to be able to estimate uncertainties: in particular, given that $T=T_0\pm \delta T$, $\alpha=\alpha_0\pm\delta\alpha$ and $\beta=\beta_0\pm\delta\beta$, we ask them to estimate $d$ up to order one (i.e. using first-order Taylor series). This already involves derivatives of multivariable functions, and is an important computation when you want to draw conclusions from experiments.
Another important point of the course is the use of logarithms and exponentials, in particular to interpret log or log-log graphs. For example, in the above model, it takes a (very) little habit to see that taking logs is a good thing to do: $\log d = \beta\log T+\log \alpha$ so that plotting your data in log-log chart should give you a line (if the models accurately represent your experiments).
This then interacts with statistics: one can find the linear regression in log-log charts to find estimates for $\alpha$ and $\beta$. But then one really gets an estimate of $\beta$ and... $\log\alpha$, so one should have a sense of how badly this uncertainty propagates to $\alpha$ (one variable first-order Taylor series: easy peasy).
The other main goal of the course is to get them able to deal with some (ordinary) differential equations. The motivating example I chose was offered to me by the chemist of our syllabus meeting.
A common model for the kinetics of a chemical reaction
$$A + B \to C$$
is the second-order model: one assumes that the speed of the reaction is proportional to the product of the concentrations of the species A and B. This leads to a not-so-easy differential equation of the form
$$ y'(t) = (a-y(t))(b-y(t)).$$
This is a first-order ODE with separable variables. One can solve it explicitly (a luxury!) by dividing by the second member, integrate in $t$, do a change of variable $u=y(t)$ in the left-hand-side, resolve into partial fractions the rational fraction that comes out, and remember how log is an antiderivative of the inverse function (and how to adjust for the various constants the appeared in the process). Then, you need some algebraic manipulations to transform the resulting equation into the form $y(t) = \dots$. Unfortunately and of course, we are far from being able to properly cover all this material, but we try to get the student able to follow this road later on, with their chemistry teachers.
In fact, I would love to be able to do more quantitative analysis of differential equations, but it is difficult to teach since it quickly goes beyond a few recipes. For example, I would like them to become able to tell in a glimpse the variations of solutions to
$$y'(t)=a\cdot y(t)-b \sqrt{y(t)}$$
(a model of population growth for colonies of small living entities organized in circles, where death occur mostly on the edge - note how basic geometry makes an appearance here to explain the model) in terms of the initial value. Or to be able to realize that solutions to
$$y'(t)=\sqrt{y(t)}$$
must be sub-exponential (and what that even means...). For this kind of goals, one must first aim to basic proficiency in calculus.
To sum up, dealing with any quantitative model needs a fair bit of calculus, in order to have a sense of what the model says, to use it with actual data, to analyze experimental data, to interpret it, etc.
To finish with a controversial point, it seems to me that, at least in my environment, biologists tend to underestimate the usefulness of calculus (and statistics, and more generally mathematics) and that improving the basic understanding of mathematics among biologists-to-be can only be beneficial.