19
$\begingroup$

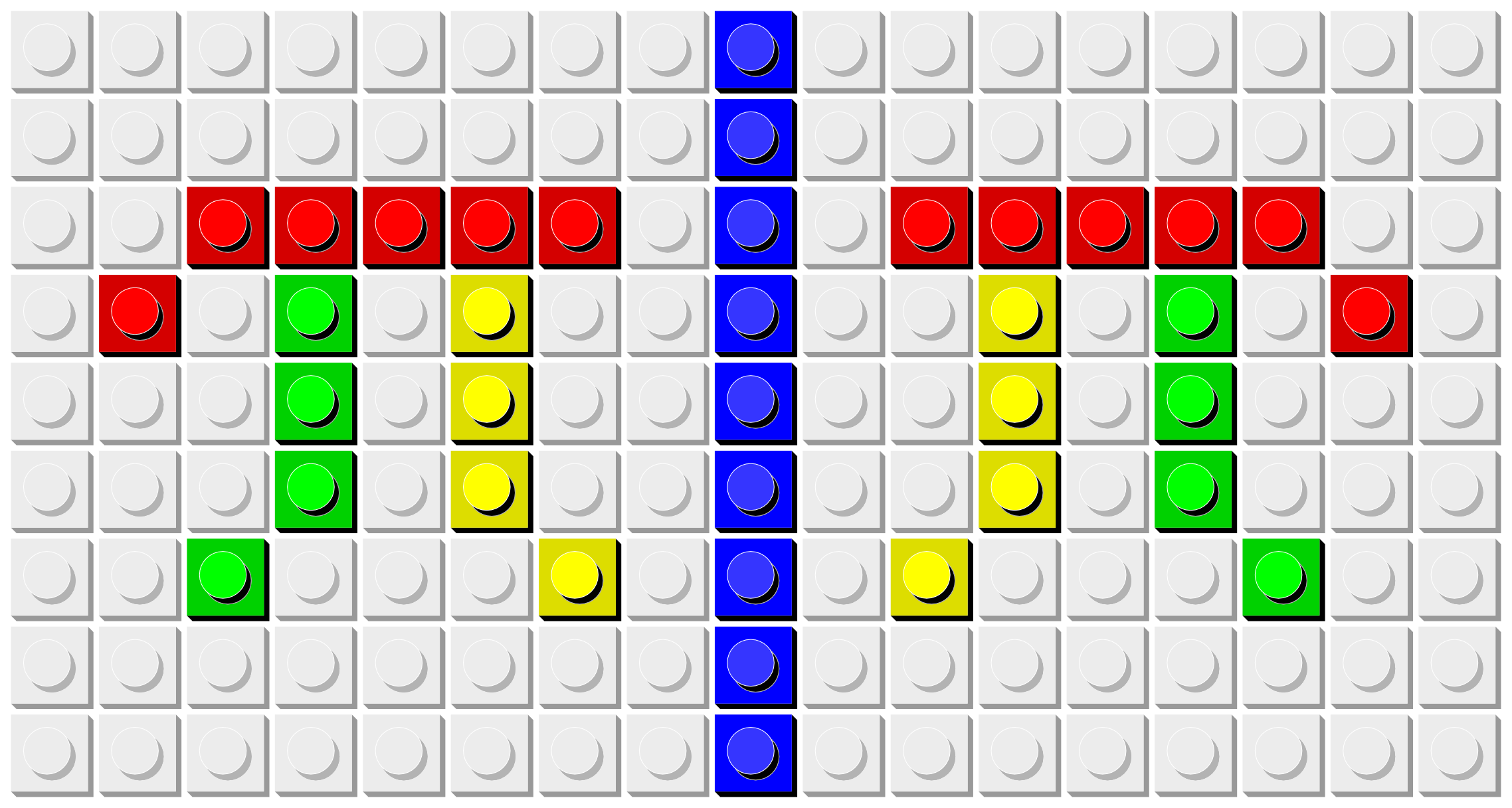
What are some mathematical problems that are feasible for preschool children to stimulate their intellectual development?

There are multiple natural laws that are not apparent to them, for example:

  • the conservation of number/quantity,
  • sets are not disjoint classes (i.e. might overlap),
  • fallacy of circular arguments.

I posted the examples as answers, so they aren't privileged. Also, it would be great if we could have one problem per answer, so the votes would represent our opinions more precisely.

Finally, the ideas for the examples I posted come from a great book "Math from Three to Seven" by A. Zvonkin, which I strongly recommend to anyone interested in this topic.

Edit: First, I would not ask a child a bare question. Some posts suggests games and games are great. In fact, all of examples I posted are easily transformable into games (the symmetry-related actually is a game already). In this light, the question could be understood:

If I had to ask a question or set a mathematical problem (in whatever form, game or not, that would be appropriate for the child), which questions and problems would be most suitable for a preschooler?

I'm looking for concrete examples. Posts like "use another approach", or "use questions that arise because of natural situations", or "just let it play some Lego" are not valid answers. In other words, I do acknowledge Lego as a great educational toy/tool, but "Lego" by itself does not answer the question. If you insist on games, how and which mathematical ideas would you incorporate into your play?

Improve this question
$\endgroup$
2
  • $\begingroup$ I thought that these types of natural laws were not apparent simply because the child has not reached that developmental point yet. Is there any research to show that children in the 3-5 years age range can understand and answer these questions correctly? Or is this just a practice in getting children to start thinking in a more critical way? $\endgroup$
    – David G
    Commented Mar 24, 2014 at 21:05
  • $\begingroup$ @DavidG Both. It seems that some ideas are not accessible before certain age, but you can make the child think. Also, it's not necessary for the child to "know" about it, even if it had reached appropriate age (i.e. the concept should be accessible). There is research on this, in particular "Piaget's phenomena" are directly related, and from what I have read, they had some limited success. $\endgroup$
    – dtldarek
    Commented Mar 24, 2014 at 21:20

12 Answers 12

Reset to default
10
$\begingroup$

For preschool children I suggest games instead of questions. Children come across some mathematical questions when they are playing games. They will learn how to solve these problems in practice (instead of abstract situations) and without any need to continuous supervision of an adult trainer (which is necessary for the case of asking mathematical questions). As a consequence they will learn by playing again and again. After a while you can see that they are inventing new games based on their new point of view.

Lego could be one of the best examples of a game with mathematical aspects. It will introduce the geometric notions of area and volume to children in an easy and effective way.

enter image description here

Improve this answer
$\endgroup$
5
  • 1
    $\begingroup$ Well, I would not ask a child bare questions in the form they were posted. Games are important, and all of my examples are easily transformable into games (the symmetry-related actually is a game already). The book I linked describes how the author would lead such games and ask questions during them (e.g. now build a tower as tall as this one, but start on the table). Did it work? The children were eager to play his games and looked forward to his sessions, sometimes asking for more after the time passed (of course, there were also failures). $\endgroup$
    – dtldarek
    Commented Mar 25, 2014 at 9:35
  • 2
    $\begingroup$ In this light, your answer doesn't really answer my question. Could you supplement your post with ideas how would you incorporate mathematical ideas into your play? $\endgroup$
    – dtldarek
    Commented Mar 25, 2014 at 9:39
  • 1
    $\begingroup$ I upvoted this because I honestly think I myself learned so much playing with legos. There was area and volume calculation - somewhat limited geometry as you are e.g. restricted to straight angles - but most importantly becoming familiar with having a 3D-image of what you want to build in your head. Mind you, I'm referring to ages about 7-10, so not exactly preschoolers. $\endgroup$
    – Jyrki Lahtonen
    Commented Mar 25, 2014 at 18:09
  • 2
    $\begingroup$ I like your answer, but as I stated before, it does not answer the question. See the edits for additional info. $\endgroup$
    – dtldarek
    Commented Apr 4, 2014 at 12:05
  • $\begingroup$ Maybe worth saying that the potential for games for learning mathematics extends well beyond the early years. See this post by Terry Tao on possibly "gamifying" algebra: terrytao.wordpress.com/2012/04/15/gamifying-algebra $\endgroup$
    – Todd Trimble
    Commented Apr 5, 2014 at 2:09
10
$\begingroup$

To supplement the "games not problems" answer above (I love that answer): Certain games provide interesting opportunities for discovery...even if you don't know anything about the rules!

An example is Tantrix.

enter image description here

The tiles feel good in one's hands, and most preschoolers will spontaneously start tiling if given some of these. If the tiling continues, automatically paths and loops form. It is possible that the child will even try to form monochromatic paths and loops. My three-year-old was alternately tiling with these the other day, and pretending that they were cookies. As the child gets older, there are other great activities to do with these tiles.

Although it is controversial to say, certain video games are good practice for mathematical rule-following for preschoolers. A simple example is this maze game. The other games on the mathisfun.com site are quite good for engaging little ones.

As a rule, I try to be on the lookout for things that encourage "combinatorial play". These are things that have multiplicity and can be combined and recombined and promise discovery by doing so. Classic lego blocks are the example everybody knows, but found items with combinatorial richness are also good.

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ These are nice, concrete examples, thank you! $\endgroup$
    – dtldarek
    Commented Mar 26, 2014 at 14:05
  • $\begingroup$ You are welcome! $\endgroup$
    – Jon Bannon
    Commented Mar 26, 2014 at 14:37
  • $\begingroup$ I just found out about this: nsf.gov/news/now_showing/tv/cyberchase.jsp $\endgroup$
    – Jon Bannon
    Commented Jul 21, 2014 at 11:54
9
$\begingroup$

Addition/subtraction by measurement

I'm not sure whether this is at all what you had in mind, but here comes anyway. When I was something like 2-3 years old my Dad taught me to add and subtract single digit numbers by sawing (and sanding for safety) pieces of wood of dimensions about half an inch x $2$ inches x $2n$ inches, where $n$ is the integer to be presented. He also drew the digit in the middle in red ink. The idea was then to add, say $2+3$, by juxtaposing the sticks representing $2$ and $3$ and then compare the total length to that of longer sticks and find the one that matches. He also defined $a-b$ as a solution of the equation $b+x=a$ that can similarly be solved by finding the stick that fits.

A generation later I repeated the exercise. It worked to my satisfaction and to my son's amusement. When my son started school (in Finland first graders are aged 7) his teacher asked parents for ideas for an "evening at school". So I did this number again. The kids picked it up immediately, and while they were excited for the allotted 5 minutes or so, it was apparent that it was too easy for most of them (some kids remained silent, so I cannot give any stats). The teacher was observing me with a group of kids, and he suggested afterwards that an average 7-year-old's cognitive ability has, indeed, developed enough to deal with the quantity concept more abstractly, and this was thus easy. He did think that this model is beneficial for pre-schoolers. If somebody knows enough about the cognitive development of children, I would appreciate a confirmation/reference/link.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Well, that's exactly I was asking about :-) The problem is great, perhaps it could be also performed using clay and kitchen knife. $\endgroup$
    – dtldarek
    Commented Apr 5, 2014 at 13:06
  • 1
    $\begingroup$ You can also buy these ready made. Cuisenaire Rods are made for this purpose and there are educational materials that accompany them. There are many videos on you tube for teaching with Cuisenaire Rods, so you could make them and then search for how to use them. $\endgroup$
    – Amy B
    Commented Mar 27, 2018 at 15:35
8
$\begingroup$

A good math curriculum to check out for Pre-K to K is Big Math for Little Kids.

You can find some information about it in this interview with co-developer Herb Ginsburg.

Ginsburg is also working on software that will include problems for the range you have specified. In particular, he and others (including doctoral students at Teachers College Columbia University) are working on a set of computer activities called MathemAntics.

Some of his students have also gone on to create their own apps. Two worth mentioning are Teachley and Tiggly. Although there are many apps and instances of software put out for the teaching of Pre-K students, it is very rare to find cases in which the development of these programs is based on actual research about early childhood mathematics. In this respect, the links above are exceptions to the norm.

Finally, if you are a bigger fan of book-learning, I must recommend the classic Mirror Puzzle Book by Marion Walter. (This is the same Walter who co-wrote, with Stephen Brown, "The Art of Problem Posing.")

In response to the OP's edit, here is a concrete example. The source of this is:

Ginsburg, H. P. (2009). Early mathematics education and how to do it. Handbook of child development and early education: Research to practice, 423-428.

enter image description here

The description continues:

enter image description here

Final note: It is important to realize that just allowing children to engage in free-play is not the best way to promote mathematical thinking. There should be some amount of structure and scaffolding by the teachers, which requires a real knack for observation and interaction. With regard to the latter, those who teach mathematics to Pre-K students may wish to familiarize themselves with the clinical interview technique. This approach is in keeping with the general thrust of Piaget's work (another good source for problems to give to students of a young age). For more on the clinical interview, see:

Ginsburg, H. (1997). Entering the child's mind: The clinical interview in psychological research and practice. Cambridge University Press.

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ Thanks for the tiles, I will check them out for sure! $\endgroup$
    – dtldarek
    Commented Mar 26, 2014 at 14:06
  • $\begingroup$ @dtldarek Great; I would be interested to hear (read) your feedback on any of the suggestions. $\endgroup$
    – Benjamin Dickman
    Commented Mar 26, 2014 at 20:22
6
$\begingroup$

Symmetry

Suppose the blue line is a mirror, how the figure would look like in it (i.e. put the blocks at the right side to recreate the left side)?

symmetry

The purpose of this problem is to train pattern recognition. There are multiple follow-up challenges (point-symmetry, scaling, etc.).

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ This is good. Less dependent upon convention, and to the extent it does so, explains the convention clearly. $\endgroup$
    – paul garrett
    Commented Mar 25, 2014 at 0:46
  • $\begingroup$ I like this, too. A just-in-case warning: can you do this with real blocks, or may be computer aided? Your target group may include kids motorically challenged to do this with pencils/crayons/whatever. $\endgroup$
    – Jyrki Lahtonen
    Commented Apr 13, 2014 at 8:26
  • $\begingroup$ @JyrkiLahtonen I think that real blocks are even better than paper-based version (computer or tablet should work as well). In fact, the picture I made does represent blocks on purpose :-) $\endgroup$
    – dtldarek
    Commented Apr 13, 2014 at 10:15
6
$\begingroup$

Odd one out

There is a bird, a plane, a square with a hole and a cloud, which one does not fit? bird,plane,square,cloud

The purpose of this problem is to highlight that explanations are as important as answers. In fact, any object can be singled out:

  • the bird, because it's the only animal;
  • the plane, because it's the only mechanical object;
  • the square, because it's abstract, or because it does not fly;
  • the cloud, because it's gas/liquid (immaterial, but not abstract).
Improve this answer
$\endgroup$
4
  • $\begingroup$ I remember thinking, as a child, that the pictures in such "tests" were themselves abstractions, since they were cartoonish depictions of the things... and felt that I could not reliably duplicate the state-of-mind of the grown-up exam-makers. E.g., were they oblivious to cartoonishness? To "depictions" rather than the things themselves? To cartoons versus photos? Seemed too complicated to decrypt, unlike the "puzzle" of many grown-ups' "tests/challenges" to kids. $\endgroup$
    – paul garrett
    Commented Mar 25, 2014 at 0:30
  • $\begingroup$ @paulgarrett Was it enough if someone said "suppose that those are real objects, not their depictions"? It reminds me of René Magritte and his "This is not a pipe". $\endgroup$
    – dtldarek
    Commented Mar 25, 2014 at 9:26
  • $\begingroup$ Could the downvoter explain? $\endgroup$
    – dtldarek
    Commented Mar 25, 2014 at 18:56
  • $\begingroup$ My first two answers: 1) The geometrical figure. It is the only one that does not go in the sky. 2) The cloud. It is the only one that does not have any red or orange. $\endgroup$
    – Jasper
    Commented Mar 22, 2015 at 3:57
4
$\begingroup$

Have a collection of objects and ask the child to put them in order based on some attribute of them.

For example, my wife Catherine (a preschool teacher) and I came up with the following activity which we did with a group of children: We got empty cardboard milk containers and filled them with different amounts of sand and then glued them shut. Then we asked the children to put them in order of how heavy they were.

We've also done it with pictures of sunflowers and asked them to order them by height, and shakers which we asked to put in order of loudness. Each of these activities were part of group sessions where a story was told or read that had something to do with what the children were ordering. For example, with the sunflowers, they read Titch by Pat Hutchins.

The idea is that before children can learn to measure things, they have to be able to compare things, and they need to realise that there are multiple aspects of objects that can be compared.

Improve this answer
$\endgroup$
3
$\begingroup$

Counting

In the pictures below, are there more cats or dogs?

6cats 7dogs

The purpose of this problem is to teach that moving or rearranging objects does not change the count. If the child does not see this, we remove some cats (spreading them far enough so they take "more space" than dogs) until arrive at an absurd situation (two cats versus bunch of dogs).

Improve this answer
$\endgroup$
3
  • $\begingroup$ This does broach another genuine issue: the "bulk" of the dogs appears greater than the "number". "More" does not clearly distinguish. $\endgroup$
    – paul garrett
    Commented Mar 25, 2014 at 0:47
  • $\begingroup$ @paulgarrett Would resizing the picture be enough or would you need something else? The experimenters usually did this with things like matches and coins, and then "the spread" was enough to ensure which of the two groups was bigger. In other experiment they used clay and then when the child claimed some part was bigger, they would remove some material and transform the rest so to match the previous length. $\endgroup$
    – dtldarek
    Commented Mar 25, 2014 at 9:19
  • $\begingroup$ Perhaps it really does depend on what one is aiming to "test"... $\endgroup$
    – paul garrett
    Commented Mar 25, 2014 at 13:06
3
$\begingroup$

Which tower is taller?

Which tower is taller (measuring from the base of the tower)?

2towers

The purpose of this problem is to teach that height (and perhaps other measurements) are translation-invariant.

Improve this answer
$\endgroup$
3
  • $\begingroup$ "Higher" is ambiguous... despite the parenthetical comment. "Taller"? Some kids are sensitive to such... $\endgroup$
    – paul garrett
    Commented Mar 25, 2014 at 0:31
  • $\begingroup$ I agree, "higher" is not clear. Both my kids have in the past stood on chairs and then announced “I'm taller than you, Daddy!” “No,” I replied, “you are higher than me.” $\endgroup$
    – Mark Dominus
    Commented Mar 25, 2014 at 1:34
  • $\begingroup$ @paulgarrett Thanks, fixed. $\endgroup$
    – dtldarek
    Commented Mar 25, 2014 at 9:09
3
$\begingroup$

A toy my kids have enjoyed lately (most popular with my 5 year old) is an interesting set of plastic turtles which interlock in numerous ways. These turtles illustrate many different symmetry patterns. enter image description here

They are a lot of fun. Beyond that, there are tons of pattern recognition games that can encourage children to begin thinking about abstraction. When I was little, my brother and I used to look for triangles, we found them everywhere. The manipulatives to illustrate 1, 10, 100 and 1000 are also a big hit. Although, I mostly see them being used to build towers, I'm not sure how much math is there just yet. Honestly, the most important thing is just to nurture a love of learning in general. Math will follow when the time is ripe.

Improve this answer
$\endgroup$
2
$\begingroup$

Sets and subsets

What is there more of in the whole world: cats and dogs, or animals?

cats_and_dogs animals

The purpose of this problem to teach the children that "the whole is bigger than its part" and to stimulate them to grow out of the disjoint-classes thinking.

Improve this answer
$\endgroup$
5
  • $\begingroup$ Still a bit of a problem in interpreting cartoons... and, seriously, I very vividly remember trying to guess adults' intentions. $\endgroup$
    – paul garrett
    Commented Mar 25, 2014 at 0:45
  • $\begingroup$ @paulgarrett I don't think that would be an issue here, the answer does not depend on whether you count real animals or cartoon pictures – there are still more cartoon animals than cartoon cats and dogs! $\endgroup$
    – dtldarek
    Commented Mar 25, 2014 at 9:21
  • $\begingroup$ But I recall wasting time being confused "in advance" because I wanted to know the adults' intentions before proceeding. Maybe it was just me... :) $\endgroup$
    – paul garrett
    Commented Mar 25, 2014 at 13:05
  • $\begingroup$ @dtldarek how did you make all of these graphics? Or did you get them from somewhere? They are very cute. $\endgroup$
    – Steven Gubkin
    Commented Nov 5, 2014 at 15:58
  • 1
    $\begingroup$ @StevenGubkin I've never been good with animals, so I was trying to draw something similar to pictures found online. For example the sleeping cat was based on this tutorial, while the terrier was based on a mix of photos, drawings, etc. (although it came out very close to one particular). Maybe one day I will be able to make a tutorial like that myself $\ddot\smile$ (The tool I'm using is inkscape.) $\endgroup$
    – dtldarek
    Commented Nov 5, 2014 at 17:44
2
$\begingroup$

One should start very easy!!! A long time ago, I where on a beach with my (then) four year old daughter. I asked "How many grains of sand do you think it is on the beach?". She thought a little and then said "seventeen?" Then we started to count, and it was soon obvios that guess was far off!

So I guess my answer is: just take advantage of natural situations!

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ Your post does not answer the question. See the edits for additional info. $\endgroup$
    – dtldarek
    Commented Apr 4, 2014 at 12:06
  • $\begingroup$ And if she really wanted to know, she'd someday be old enough to learn it from the best. :) lix.polytechnique.fr/Labo/Ilan.Vardi/sand_reckoner.ps $\endgroup$
    – Vectornaut
    Commented May 10, 2015 at 4:54

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.