10
$\begingroup$

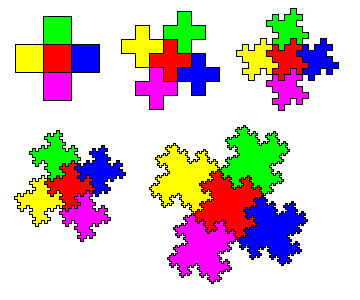
In roughly two weeks, my secondary geometry class will have reached the part of the year where we discuss geometric translations: translation, rotation, and reflection. Dilation i am going to use as a segue to similarity so I am just talking within the context of these first three transformations for the remainder of this question. These transformations will be discussed both on and off the coordinate plane. There will be some basic algebra to manipulate coordinates etc. but nothing too intense, some of my students have not taken the second algebra course yet.

I would like to frame these transformations within the context of fractals because 1) they are so visually appealing and intriguing! 2) the serve as a good motivator to investigate these different transformations and 3) ideally the final project for the unit will be to create your own fractal, either by hand or on the computer, using the transformations that we learned, which i think will be really fun for the kids and give me some awesome things to hang on my wall :)

However, I do not know a ton about fractals and am not really sure how to go about tying these two subjects without getting too in depth. I have a book called Indra's Pearls, but it gets into imaginary numbers and some pretty heavy programming which my students have limited exposure to. How can I marry these two topics at a level suitable to a secondary geometry class? Any good resources that give the "quick and dirty" on fractals?

Improve this question
$\endgroup$
2
  • $\begingroup$ Maybe this would be a good place to start; hosted by yale.edu, the link comes from notes on fractal geometry co-authored by Mandelbrot. Also: Check out 3 beginning on p. 78 of this book, which was also co-authored by Mandelbrot. $\endgroup$
    – Benjamin Dickman
    Commented Mar 26, 2015 at 6:05
  • 2
    $\begingroup$ It's an interesting idea, but since the most common definition of fractals included similarity, you might need to include dilations at some point. If all you can use is size-preserving transformations, you could consider teasels toons instead? $\endgroup$
    – DavidButlerUofA
    Commented Mar 26, 2015 at 6:13

2 Answers 2

Reset to default
10
$\begingroup$

If you start directly with fractals, you'll get a hard time because dilation and similarity are an inherent feature of fractals. You should start with tesselations and friezes. They are equally visually stunning, can be used to problem-oriented and theory-oriented investigations and there are easy computer and tablet apps to generate and play with them. And they only need the rigid motions.

From tesselations and friezes on you can get to fractals by adding dilation.

Improve this answer
$\endgroup$
6
$\begingroup$

An illustration for @Toscho's nice idea, from Steven Dutch's Rep-Tiles webpage:

  reptiles
"Four squares can be arranged around a central square to form a Greek Cross. Greek Crosses make a lovely plane tesselation, but they are not rep-tiles. We can try modifying a Greek Cross tesselation by replacing each cross with the compound of five crosses. It's closer, but still not an exact rep-tile. We can repeat the process, each time getting figures that are more and more crinkled and closer to a true rep-tiling. But at every step, we see that the perimeter of the tiling always has twice as many crinkles as each tile."

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.