I found it difficult to explain the difference between the fraction a / b and the ratio a : b. This subject is for pupils of grade 5. So is there a real difference between them and how to explain the difference in simple way ?!
$\begingroup$
$\endgroup$
13
asked Jan 24, 2015 at 5:54
-
25$\begingroup$ Could you explain the difference to me (Student of Mathematics)? $\endgroup$– JasperCommented Jan 24, 2015 at 12:19
-
4$\begingroup$ But is the ratio a:b is a number ? $\endgroup$– Abdallah AbusharekhCommented Jan 24, 2015 at 18:24
-
7$\begingroup$ Suppose there really is a difference between these two alleged concepts. Does having a clear distinction between these concepts make it easier to solve any real-world problems? Especially if you are careful to use units? $\endgroup$– JasperCommented Jan 25, 2015 at 21:20
-
2$\begingroup$ @AbdallahAbushrekh It can be. I found the wikipedia page to be quite useful: en.wikipedia.org/wiki/Ratio $\endgroup$– Chris CCommented Jan 25, 2015 at 21:28
-
7$\begingroup$ Note: Proportions $a : b : c$ also make sense, but are certainly not equal to $a/b/c$. Also note: ratios $1:0$ and $0:1$ are both allowed, but fraction $1/0$ is not allowed. $\endgroup$– Gerald EdgarCommented Nov 15, 2020 at 11:17
|
Show 8 more comments
16 Answers
$\begingroup$
$\endgroup$
2
This paragraph from Adding It Up is a good overview of the context of this question. Rational numbers are complex because there are multiple interpretations (meanings) as well as multiple forms for expressing a rational number.
As we said at the beginning of the chapter, rational numbers can be expressed in various forms (e.g., common fractions, decimal fractions, percents), and each form has many common uses in daily life (e.g., a part of a region, a part of a set, a quotient, a rate, a ratio). One way of describing this complexity is to observe that, from the student’s point of view, a rational number is not a single entity but has multiple personalities. The scheme that has guided research on rational number over the past two decades identifies the following interpretations for any rational number, say 3/4: (a) a part-whole relation (3 out of 4 equal-sized shares); (b) a quotient (3 divided by 4); (c) a measure (3/4 of the way from the beginning of the unit to the end); (d) a ratio (3 red cars for every 4 green cars); and (e) an operation that enlarges or reduces the size of something (3/4 of 12). The task for students is to recognize these distinctions and, at the same time, to construct relations among them that generate a coherent concept of rational number. Clearly, this process is lengthy and multifaceted.
I think it is important for students to understand the connection as well as the distinction between the fraction and the ratio. They can both be written as 3/4. The point to make to students is that the expression 3/4 can be interpreted in different ways. It has different meanings that are mathematically equivalent. So the students should realize that the ratio 3:4 has the same meaning as the ratio 3/4. A second point to make is that there are different forms for writing rational numbers. Using the colon and the bar are just different ways of writing the ratio. As a ratio it is a correspondence of 3 things to 4 things. The students should also realize that the expression 3/4 can be interpreted as a fraction, 3 out of 4 equal portions of some whole. The fraction is a part-to-whole ratio. There are also part-to-part ratios, like one blue dot to 2 yellow dots. Since there are three dots in total, I can also compare the blue dots to the total, giving 1 to 3 as a ratio, or as a fraction 1/3.
Give lots of examples to make it concrete. Answer their questions to clarify the connection and the difference. Then ask them questions and let them explain their thinking. For example, “There are 12 boys and 14 girls in this class. What fractions and ratios can you make from these numbers?”
-
8$\begingroup$ I don't understand this answer, so it's hard for me to imagine how children could understand this approach. $\endgroup$– user507Commented Apr 30, 2016 at 3:09
-
9$\begingroup$ This is not correct. A 3 to 4 ratio, as in cooking, means for every 7 cups of stuff used, 4 are of one type, and 3, the other. Each ingredient is then 4/7 or 3/7 of the total. The G/B ratio is 12/14 or 6/7 but there are 12/26 or 6/13 students are girls. The example doesn't make this clear. $\endgroup$ Commented May 7, 2016 at 10:20
$\begingroup$
$\endgroup$
4
I find this diagram helpful when relating the two:
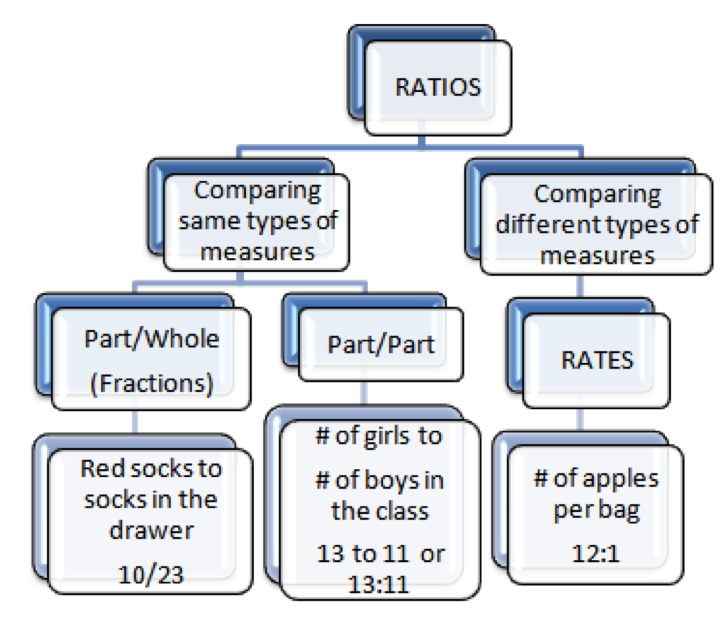
It comes from a model curriculum unit on Rates and Ratios for 6th graders (you can see them all here after registering), and I have found this particular graphic very helpful with math content professional development with 6th grade math teachers.
-
2$\begingroup$ thank you Tamisha for the diagrame .. you have just supported my undrestandind for the whole matter $\endgroup$ Commented Feb 1, 2015 at 18:06
-
-
$\begingroup$ Counterexamples: a literacy rate compares two same measures; 10:23 can be the ratio of the number of red socks to the total number of socks. That said, I find this chart a useful insight into the junior-school perspective. $\endgroup$– ryangCommented May 17 at 4:48
-
$\begingroup$ NB: we can allow a fraction to have "part" > "whole", we call such fractions improper fractions $\endgroup$ Commented Oct 13 at 11:39
$\begingroup$
$\endgroup$
1
There are several possible fractions one could associate with a given ratio.
Say that a recipe for lemonade calls for $2$ cups of lemon juice and $5$ cups of water. This could be expressed with the ratio $2:5$. Here are some different associated fractions:
- $2/7$ of the lemonade is lemon juice.
- $5/7$ of the lemonade is water.
- $2/5$ of a cup of lemon juice is the amount which needs to be added to 1 cup of water.
- $5/2$ of a cup of water is the amount which needs to be added to 1 cup of lemon juice.
I don't think any of these fractions has a right to be called "the fraction" associated with the ratio.
Some additional evidence that ratio and fraction are distinct concepts:
The ratios 0:5 and 5:0 both make sense. For instance, you could have 0 parts lemon juice to 5 parts water.
One can have ratios between more than 2 quantities. The ratio 1:2:5, as in 1 part sugar, 2 parts lemon juice, and 5 parts water makes perfect sense. This ratio would be equivalent to 2:4:10 (since the proportions are the same), but there is no single associated fraction.
For a mathematical definition one could use the following:
An ratio of $n$ quantities is an equivalence class of elements of $\mathbb{R}^n-\{\mathbf{0}\}$ under the equivalence relation $\mathbf{v} \sim \mathbf{w}$ iff there exists a $c \in \mathbb{R}-\{0\}$ such that $\mathbf{v} = c \mathbf{w}$.
In other words, ratios are just elements of real projective spaces!
Please don't tell this to your 5th graders.
answered Jul 25, 2019 at 12:27
-
2$\begingroup$ I'll tell it to all my fifth grade students. You can't stop me. $\endgroup$ Commented Apr 21, 2022 at 18:46
$\begingroup$
$\endgroup$
4
I always get students to colour in dots. 1 red and 2 blue. Here the ratio is 1:2 red to blue. I ask, "What fraction are red?" Hopefully someone says $\frac{1}{2}$ and we can discuss the misconception. In terms of how to relate $\frac{1}{2}$ to the ratio 1:2 I do it after establishing the equivalence of ratio by scaling up both sides. I want to make green from blue and yellow in the ratio 1:2. I have 2 tins of blue how many yellow? Students often say that's easy just double the tins of yellow because you have doubled the blue. Now I ask them if I had 1000 yellow or something like they say 500 but this time because they recognise there are $\frac{1}{2}$ the number of yellow. A subtle change but one that recognise the proportion of blue and yellow is the same for equivalent ratios. Hope this helps.
-
7$\begingroup$ Same thing comes up with odds and probability. If the odds are 1 to 2 against you, then the probability of winning is 1/3. $\endgroup$ Commented Jan 24, 2015 at 17:12
-
$\begingroup$ Thank you. But if we have 1 red and 2 blue the fraction will equal 1/3 not 1/2 ! $\endgroup$ Commented Jan 24, 2015 at 18:27
-
2$\begingroup$ hence the "misconception" in saying it. $\endgroup$ Commented Jan 24, 2015 at 18:34
-
$\begingroup$ @GeraldEdgar No, if the odds are 1:2 against you, then you have a 2/3 probability of winning. $\endgroup$ Commented Apr 24, 2022 at 18:33
$\begingroup$
$\endgroup$
I want to build a factory and I go to a bank for a loan, to finance part of the investment cost.
Bankers tend to think in $a:b$: "How many euros we will lend for every euro the company will invest". And they tend to have rules of thumb on the matter, say a $3:1$ rule. From the point of view of the company, this could be written $1:3$ and here, confusion may arise more easily, because "$1/3$" should be interpreted as "the company will invest one third of what the bank will lend" and not as "one third of the total cost of the factory".
To arrive at this last magnitude the relation is always $a:b \rightarrow \frac {a}{a+b}$
More generally, I think a fraction $a/b$ (which then can be written also as a number, a decimal, etc), is meaningful only when $a$ and $b$ measure same entities in nature (in my example, money in the same currency). But the concept represented usually by $a:b$ can bring together items that are not alike (say, "$a$ car-accident deaths per $b$ kilometers of highways), in which case there is no meaningful interpretation of $a+b$.
answered May 5, 2016 at 13:52
$\begingroup$
$\endgroup$
2
The ratio $a:b$ and the fraction $a/b$ are generally not synonymous and should not be treated as such, at least without making clear their interpretations. In many contexts, $a:b$ corresponds to the fraction $a/(a+b)$ or $b/(a+b)$.
For example, if one cuts a pizza into $6$ slices, one of which has anchovies, the fraction of slices with anchovies is $1/6$, while the ratio of slices with anchovies to slices without anchovies is $1:5$.
One context, unfortunately familiar to many students, where $a/b$ and $a:b$ are not synonyms is when speaking of odds in the context of bookmaking. That the odds be given by the ratio $3:2$ means roughly that the bookmaker expects the bet upon side to win $2/5$ times and the payout in the event of a win will be $3/5$ of the sum of the payout and money staked. That is when speaking of odds, the ratio $a:b$ corresponds to the fraction $a/(a+b)$ or $b/(a+b)$ (which form is relevant depends on interpretation/use). So $9:1$ odds against means that the horse is not expected to win, so the payout is big if it does, $900$ would be paid on a $100$ bet, while $9:1$ odds in favor (usually quoted as $1:9$) means that the horse is expected to win, and the payout is small if it does, approximately $11 \simeq 100/9$ would be paid on a $100$ bet.
-
1$\begingroup$ "In many contexts, 𝑎:𝑏 corresponds to the fraction 𝑎/(𝑎+𝑏) or 𝑏/(𝑎+𝑏)" — just don't call it ratio then. "the ratio of slices with anchovies to slices without anchovies is 1:5" — no, slices with anchovies correspond to slices without anchovies in proportion 1 to 5. People just use "ratio" because it is simpler to say than a "proportional relationship". Likewise, people use "rate" instead of "speed", then they wonder why kids don't understand what rate is. $\endgroup$ Commented Aug 30, 2019 at 18:58
-
$\begingroup$ @RustyCore You seem to have a nonstandard definition of "ratio". $\endgroup$ Commented Apr 24, 2022 at 18:36
$\begingroup$
$\endgroup$
2
After thinking a while about this question, here comes my first answer on matheducators.SE:
First of all, I would leave out ratios entirely, if possible. They aren't as expressive as fractions are, and fractions are more widely used.
Why are ratios less expressive?
With (binary) ratios, you can compare only two things: $a:b$ (scores in a game, male-to-female distribution in the class, ...), while with fractions, you can have $a/x$, $b/x$, $c/x$, the fraction of several things from a larger group $x$ (color of hair, election results, ...).
Of course you could also do $a:b:c:...$, but I think that's too complicated?
How to explain the difference?
You could emphasize the difference in operations on both: adding ratios is simple, adding fractions is not. Multiplying fractions, or a fraction with a number makes sense, multiplying ratios doesn't. Another key is proper use of language to make clear what is being compared: The ratio of the number of one thing to another number of things, versus the fraction of a number of things of a total number of things.
-
4$\begingroup$ I don't think that $a:b:c$ is too complicated. It nicely points out the fact that ratios written this way aren't technically numbers. $\endgroup$ Commented Jan 26, 2015 at 0:06
-
2$\begingroup$ You can't leave out ratios altogether. For one thing, fractions are ratios. Rational numbers are numbers that can be expressed as ratios. The ratio a:b can be written as a/b. $\endgroup$ Commented Jan 26, 2015 at 20:10
$\begingroup$
$\endgroup$
1
By itself, the word ratio is indeed rather opaque and should probably be avoided. Here is what "is under the hood and usually goes without saying".
Natural (aka counting) numbers are used to measure a "quantity" (= counting number) of discrete objects such as in: the "quantity" of the set {egg, egg, egg} is 3. What do we do when we want to measure a "quantity" (= real number) of continuous stuff such as length?
In terms of proportion, the ancient Greeks would have said that the "quantity" (length) of a footstick is to the "quantity" (length) of a yardstick the same as the "quantity" (count) of the set {egg}, i.e. 1, is to the "quantity" (count) of {egg, egg, egg}, i.e. 3. In terms of ratios, they would have said that the ratio of footstick to yardstick is equal to the ratio of {egg} to {egg, egg, egg}. When we say that the "quantity" of a footstick equals $\frac{1}{3}$ the "quantity" of a yardstick, we are just writing the proportion another way.
In other words, the idea of proportion was meant to reduce the measure of "quantity" of stuff (which they couldn't do) to the measure of "quantity" of sets (which they could do).
From my (by now very remote) experience with 5 graders, I think that the above distinction, measuring sets versus measuring continuous stuff (which, by the way, requires the introduction of units). is quite within their reach.
Warning: what follows may be a bit off-topic but is tightly related to the question.
The trouble comes when we want to look at a fraction as indicative of a measure the same way as when we look at a natural number as indicative of a measure. For instance, when we look at 3084385 and 47975 we immediately see that the first is larger than the second. Not so immediately with fractions.
So, of course, we look at $\frac{1}{3}$ as code for "divide 3 into 1" but the question now is "where to stop the division?" and that of course depends on the real-world situation. That we have rules for dealing with the code, e.g. $\frac{a}{b}\times\frac{c}{d}=\frac{ac}{bd}$ is nice and fortunate but not really necessary: as engineers are wont to put it, "The real real numbers are the decimal numbers".
See Gowers Mathematics, A Very Short Introduction. While spending a whole chapter (7) on infinite decimals, Gowers never even mentions real numbers. And, appropriately recast, the content of Gowers' Chapter 7 should be accessible to 5 grader.
-
3$\begingroup$ Why are the "real real" numbers the decimal numbers and not the base 3 numbers? The faction 1/3 is much easier to express in base 3, and is in no sense less mysterious than expressing 1/10 in base 10. $\endgroup$ Commented Nov 15, 2020 at 18:13
$\begingroup$
$\endgroup$
2
Cooking examples work great here.
Rice is made with 1 part rice 2 parts water. The ratio is 1:2 rice:water. Or we can say 1/2 the water is the volume of rice to use. Or, as is often the case, you have an odd amount of rice, use twice that amount of water. I once saw my sister fill a 1 cup measure with rice, throw away the rest, and then add 2 cups of water. I asked why she threw out what looked like another 1/2 cup of rice. It wasn't enough for a "recipe". I don't know if she was a bad cook or bad at math, but she could have just measured 1-1/2 cups rice and double to 3 cups water to use.
We use 2:1 for Margarita's as well. 2 parts Mix to 1 part Tequila. Mix to Tequila is 2:1, and the final drink has 1/3 Tequila, 2/3 Margarita Mix.
It's key to understand that (ratio) 2:1 results in 3 parts of stuff, made up of (fraction) 1/3 one ingredient, 2/3 the other.
answered May 7, 2016 at 16:50
JTP - Apologise to MonicaJTP - Apologise to Monica
9,8133 gold badges44 silver badges71 bronze badges
-
1$\begingroup$ I loved your example using cooking examples! Great job! Going to use it in my lesson. $\endgroup$– user7088Commented Aug 28, 2016 at 13:41
-
$\begingroup$ 1 fresh squeeze lime juice : 1 orange liqueur (I prefer Cointreau, but Triple Sec is more traditional) : 2 tequila. On the rocks. Mix is teh debil (yes, "teh"; not "the"). :P $\endgroup$– Xander Henderson ♦Commented Jul 25, 2019 at 21:33
$\begingroup$
$\endgroup$
Mathematically there really is no difference. The words "fraction" and "ratio" simply express the concept of dividing one number by another, and are used pretty much interchangeably in mathematics. They need not refer to comparing a part to a whole. The words "fraction" and "ratio" are also used with reference to division of complex, and hypercomplex numbers, where the concept of a part to a whole is completely meaningless.
One difference in how the words are used is that the word "fraction" is often used to represent a specific representation of a ratio. For example 3/4 and 6/8 might be considered different fractions which represent the same ratio. You might also run across phrases like "when dividing two complex numbers, first write the ratio as a fraction with a real denominator."
$\begingroup$
$\endgroup$
4
Ratio is just a single fraction. Whereas proportion is a ratio between 2 fractions.
Example - 5 boys and 3 girls in a class. Ratio of boys is 5/8 Ratio of girls is 3/8 But proportion of boys to girls is 5:3
The basic difference is Ratio is always with respect to some large quantities or superior quantities, whereas proportion is between same kind of quantities.
-
1$\begingroup$ I have never seen this distinction made. Out of curiosity, can you link to a source that does this? $\endgroup$ Commented Apr 28, 2016 at 14:01
-
1$\begingroup$ A proportion is an equation stating that there are two equal ratios 3:5=6:8. If you google "define proportion mathematically", you will find this definition and the definition on many math sites you used. $\endgroup$– Amy BCommented Apr 28, 2016 at 14:21
-
1$\begingroup$ @AmyB "A proportion is an equation stating that there are two equal ratios 3:5=6:8" — Indeed. The problem is, these two particular ratios are not equal ;-) $\endgroup$ Commented Jul 23, 2019 at 23:39
-
2$\begingroup$ @RustyCore It shouldn't have taken 2 years for someone to notice that! I tried to edit but wasn't able to so I will rephrase: A proportion is an equation stating that there are 2 equal ratios 3:5=6:10. If you google "define proportion mathematically" you will find this definition on many math sites. $\endgroup$– Amy BCommented Jul 25, 2019 at 5:33
$\begingroup$
$\endgroup$
Relation between division and fraction have to be understood to understand the relationship between fraction and ratio: 1. Division: finding how many times of divisor in the dividend, or the value of a part 1/5 means 5 is 0.20 times in 1; value of one part is 0.20. 2. Fraction: It is how many times the denominator in numerator or how many parts of denominator in numerator. It means multiplying the value of one part with numerator: 2/5 = 1/5 X 2 = 0.40 3 Ratio: Ratio is a division finding how many times one entity in to other. And it is a fraction for one in numerator. 4. All are written in fraction form with same value
Division and Fractions have applications in daily life where as ratio being an another form of division and fraction does not find place in daily life applications. Therefore ratio is not an independent entity but a name to refer how many times in a division in context. Probably it is good idea not to teach ratio as an independent entity. Therefore ratio is an application of fractions like percentage, rebate, loss and profit. They all use the properties of equivalent fractions. Proportion too is an equivalent fraction.
$\begingroup$
$\endgroup$
Focusing on the difference between the abstract concept of a ratio and a fraction is likely to confuse students who are just learning these concepts.
The goal should be that they eventually understand and become skilled with the abstraction a fraction. The concept being abstract means, that it can be applied in a wide variety situations and can be used as a model for many concrete examples. The key to understanding is eventually seeing what is common between seemingly different things.
In grade 5, it suffices to say, that the difference is in the notation:
a/bis meant to denote thatais the part,bis the whole,a:bis meant to denote, thataandbare two complementary parts of the same whole.
The notation a/b is useful and is a good basis to build further abstractions and calculations later on. The notation a:b is less useful, and is mostly only used in noting down concrete problems, and is not developed further for more abstractions that students will use later as often as with the fraction a/b.
That is why it is not so useful to go into abstract explanations of what is the difference between the "definition" of a fraction and a ratio. Just explain it in the minimalistic sense of what the notation means, and then focus on developing their understanding of the concept of a fraction.
$\begingroup$
$\endgroup$
I haven't taught this yet, I teach Munchkins but for me it looks like if we have:
Fraction = a/b and Ratio = x:y then
b = x+y
Also x:y is ALWAYS about one Unit of anything BUT a/b can be a Part of a Unit, a Unit or more than a Unit of anything.
$\begingroup$
$\endgroup$
3
Above while representing ratio in a flow diagram above one branch is represented by part/part. There is no such concept as part/part in fraction form. The denominator is always a 'whole'. While comparing two items one of them should be taken as whole and other as part of the whole.
The diagram in the present form violates the fundamental principle of fraction.
-
1$\begingroup$ I don't understand why fractions cannot be used to describe part/part relations. For example, in the flow chart, 13 boys and 11 girls is represented by the ratio $13 : 11$. As a fraction, you may regard this as either $$\frac{13}{11} \qquad\text{or}\qquad \frac{13/T}{11/T} = \frac{13/24}{11/24}, $$ where $T$ is the total number of students (which is $24$, hence the second expression). $\endgroup$– Xander Henderson ♦Commented Jul 25, 2019 at 21:37
-
$\begingroup$ @XanderHenderson I do not see the meaning of the fraction $13/11$ in this context. It could mean something like ``For every 1 girl, there is $13/11$ of a boy'', but that is awkward. $\endgroup$ Commented Aug 27, 2019 at 12:18
-
1$\begingroup$ Or... for every 13 boys, there are 11 girls. This seems to be a question of dimensional analysis, which was taught in my high school chemistry and physics classes. For example: there are approximately 3.3 feet per meter, so we can use the conversion factor $$ \frac{33 \ \text{feet}}{10\ \text{meters}} $$ to convert from meters to feet. We could also use $3.3\ \text{feet}/\text{meter}$, but working with whole numbers is sometimes easier. $\endgroup$– Xander Henderson ♦Commented Aug 27, 2019 at 13:25
$\begingroup$
$\endgroup$
I'm not sure how you would translate the following into the language of 10-year-olds, but the teacher should first understand this much:
The variables x and y are respectively in the ratio a:b means only that x/y = a/b (for non-zero values of a, b, x and y).
Similarly, the variables x, y and z are respectively in the ratio of a:b:c means only that x/y = a/b and y/z =b/c (for non-zero values of a, b, c, x, y and z).
