I don't think this is a nationalistic difference (I'm in the US), but I also don't think your example is optimal. As an example where the correct technique is more well defined, let's say we have a physics problem like this:
A bug starts from rest and accelerates with constant acceleration for 0.53 s, traveling 1.37 m. Find the bug's acceleration.
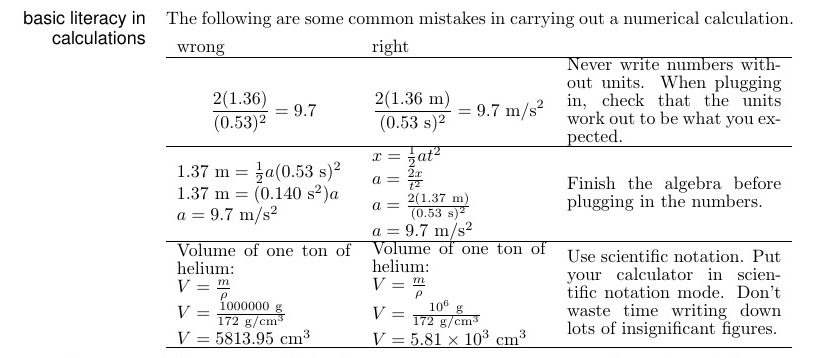
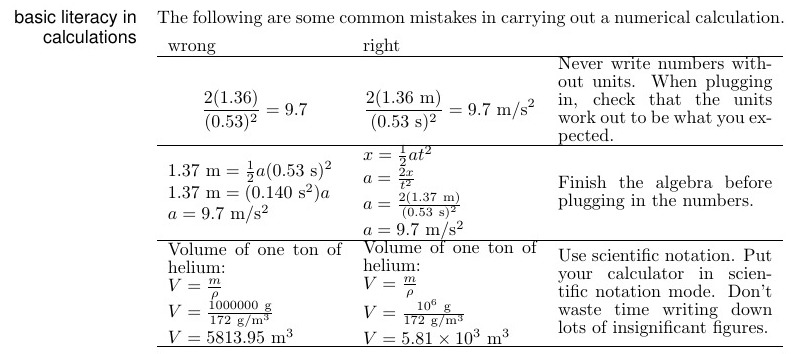
I would consider it wrong wrong wrong to solve this by first writing down the equation $x=(1/2)at^2$, then plugging in numbers, then solving for $a$. As you say, a competent person will do the algebra first and then plug the numbers in at the last step. Below is a section from my syllabus for a physics course where I present this kind of thing, including this example.
The unfortunate reality today is that many physics instructors use computerized grading of homework, and they implement this in such a way that they never actually see their students' written work. Therefore their students never get feedback on this kind of thing.