Here are a number of examples of Calculus problems that ChatGPT was not able to solve satisfactorily (at least now, but it may learn!)
Example 1) (Use large numbers) Explain how you would integrate $\int\sin^{679}(x) dx$, but don't find the answer.
The successful solution by a student is to separate one $\sin(x)$, then write the remaining $\sin^{678}(x)$ as $(\sin^2(x))^{339}$, which is equal to $(1-\cos^2(x))^{339}$ and then say use the substitution $u=\cos(x)$, although the answer to that integral will be very complicated and long.
Here is ChatGPT's response to the same question:

Example 2) Suppose the series $\sum_{n=1}^\infty a_n^2$ is convergent. What can we say about the convergence or divergence of the series $\sum_{n=1}^\infty \frac{a_n^2}{n}$?
The successful solution by a student will use the Comparison Test, as $0\leq \frac{a_n^2}{n}\leq a_n^2$ and conclude that the series $\sum_{n=1}^\infty\frac{a_n^2}{n}$ is convergent.
Here is ChatGPT's response to the same question (note that the conclusion is correct but the reasoning doesn't make sense):

Example 3) (Include extraneous information) Suppose $f$ is a continuous function and $g(x)=\int_1^x f(t)dt$. If $f(4)=0$ and $f(5)=1$ can we find a critical number of $g(x)$?
The successful solution by a student is to say since $f$ is continuous, $g$ is differentiable and $g^\prime(x)=f(x)$. Since $g^\prime(4)=f(4)=0$, $x=4$ is a critical number of $g$. That $f(5)=1$ is irrelevant.
Here is ChatGPT's response to the same question, which does not make sense:

Example 4) suppose $f(x) = x+3$ when $x\leq-2$, and $f(x) = -x/2$ when $-2<x<2$ and $f(x) = x-3$ when $x\geq2$. Suppose $g(x)=\int_{-4}^x f(t)dt$. Can we find the extreme values of $g$ on the interval $[-4,6]$?
The successful solution by a student would use the Closed Interval Method. As $f$ is continuous, $g^\prime(x)=f(x)$ and $g$ has critical numbers at $x=-3,0,3$. To find the extreme values of $g$ on the interval $[-4,6]$ it suffices to find and compare the values of $g(-4), g(-3), g(0), g(3)$ and $g(6)$.
Here is ChatGPT's response to the same question, which doesn't make sense (it actually got stuck for more than 5 minutes there):

Since ChatGPT got stuck in the middle of solving this problem, I stopped it and asked it to regenerate its response. In its new response, somewhere it said that $-3$ is not in the interval $[-4,6]$! So I challenged it and here is the conversation that followed:
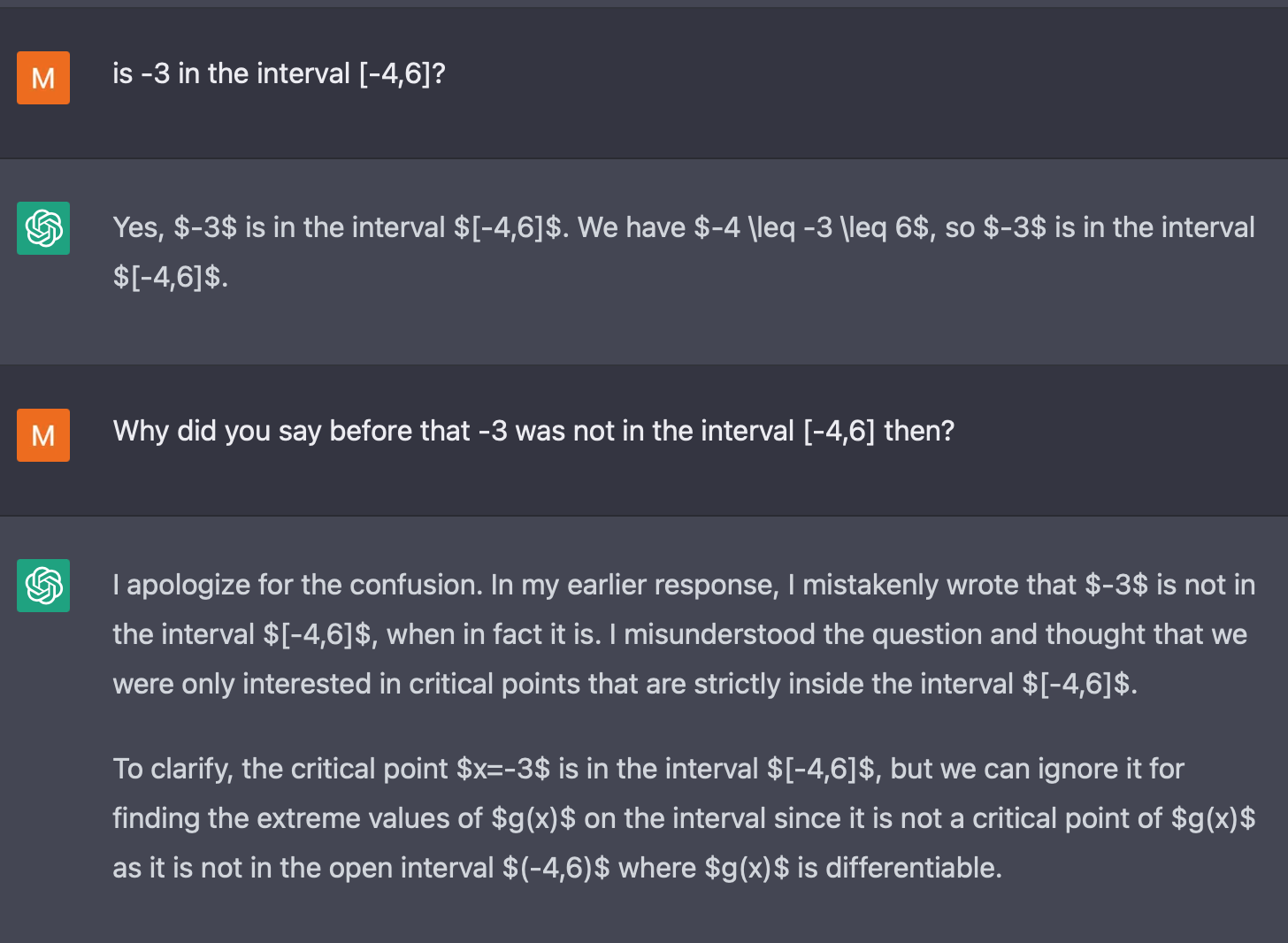
Note that it is still saying $-3$ is not in the open interval $(-4,6)$!
Example 5) Is it possible to integrate $\frac{x}{\sqrt{9-x^2}}$ using integration by parts?
ChatGPT got stuck again in the middle of solving the problem:


Example 6) Here is an elementary question: Can a rectangle and a circle have the same area?
Here is ChatGPT's response:








