Is there a way to extend the analogy that fractions means "x out of y" to show that fractions are also dividing?

Question

When explaining fractions to my kids, I've used the analogy that $\frac{a}{b}$ means "you want $a$ out of every group of $b$ (of the thing you're finding a fraction of)."

E.g. $\frac{3}{4}$ of a pizza means "you want $3$ out of every group of $4$ (pizza slices) of a pizza (that's been sliced up to let you make groups of 4)". So if you sliced up the pizza into $8$ slices, you'd have $2$ groups of $4$, so if you took $3$ from each group of $4$, you'd have $6$ slices, which is your $\frac{3}{4}$ of the original pizza that was cut up into 8 slices.

This analogy works pretty well for fractions that are "$<=$ $1.0$," like $\frac{3}{4}$, $\frac{5}{7}$, $\frac{11}{11}$, etc.
But it seems to break down (or at least I can't find a way to extend it) when considering fractions that are $>$ $1.0$, like $\frac{8}{4}$.
Like: the extended analogy for $\frac{8}{4}$ would be "you want $8$ out of every group of $4$ pizza slices (of a pizza that's been sliced up to let you make groups of 4)."
I can't wrap my head around whether that makes grammatical sense, let alone mathematical sense.

I do realize that $\frac{8}{4}$ can be thought of as "$\frac{1}{4}$ of $8$", in which case we can go back to our nice analogy that seemingly only works for fractions that are $<=$ $1.0$.
But here, I struggle for a way to explain (to 8-year-olds) why $\frac{8}{4}$ can be thought of as "$\frac{1}{4}$ of $8$" in the context of the original analogy that $\frac{a}{b}$ means "you want $a$ out of every group of $b$".

So I think my question comes down to: is there a way to extend the analogy that $\frac{a}{b}$ means "you want $a$ out of every group of $b$" to account for when $a > b$ (i.e. that it effectively means ${a}\div{b}$)?

Answer 1

I think the heuristic

\begin{align*} \frac{8}{4} \quad \longleftrightarrow \quad 8 \textrm{ out of every group of } 4 \end{align*}

still makes sense if you emphasize that you want $8$ slices of pizza out of every group of $4,$ even if it's not possible to take that many slices.

If you want more slices than there are available in the group, then what you're really saying is you want more than a full pizza.

This naturally leads to the follow-up question "Then how many full pizzas do you want?" and the answer to that question is to divide $8 \div 4.$

Here's how I imagine this actually being explained to a kid. (I'll refer to "pancakes" instead of "pizzas" since it's easier to imagine oneself hungry for multiple pancakes than for multiple pizzas.)

Kid: How can you take $8$ slices out of every $4$ slices?? That doesn't make sense. There are only $4$ slices. You can't take more than $4$ of those slices.

You: You're right. You can't actually take $8$ of those $4$ slices, because there are only $4.$ But you can want $8$ slices that are the same size as those $4$ slices.

Imagine that we're having pancakes for breakfast. Normally, you don't even want a full pancake. So I cut up a single pancake into $4$ pieces and ask you how many of those pieces you want.

But today you're really hungry and you want to eat not only the full pancake (all $4$ pieces), but also ANOTHER full pancake! You want to eat two full pancakes instead of just one.

But I don't know this, so I bring out a pancake that's cut into $4$ pieces and ask you how many of those pieces you want. You have to answer my question with a number. What number can you tell me, that indicates you want more than I'm offering you?

Well, you just tell me how many pieces of those size you want. You want the entire pancake that I'm offering you ($4$ pieces), and another full pancake (another $4$ pieces of the same size). So you should tell me that you want $4+4=8$ of those pieces.

Answer 2

Justin Skycak wrote a great answer that you can use with your kids, but I'd like to explain how what you're telling them relates to what they might be seeing right now and later on in school. I'll be referring to the U.S. Common Core State Standards (CCSS), but many curricula in the U.S. and in other countries follow similar approaches, though timing may differ.

In the CCSS, fractions are introduced in grade 3. Here's an excerpt from the standards.

Students develop an understanding of fractions, beginning with unit fractions. Students view fractions in general as being built out of unit fractions, and they use fractions along with visual fraction models to represent parts of a whole... Students are able to use fractions to represent numbers equal to, less than, and greater than one.

This means that we want third graders to view $\frac{8}{4}$ as "8 parts when each whole is divided into 4 parts." To use pizza as an example, we would say "One pizza is a whole. Divide each whole into 4 parts. 8 of these parts makes $\frac{8}{4}$." Of course, this means that $\frac{8}{4}$ is more than 1 whole!

Here's what a grade 3 workbook exercise from a popular publisher looks like.

Each shape is 1 whole. Write a fraction greater than 1 for the parts that are shaded.

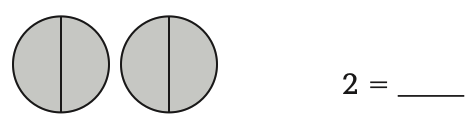
A third grader might reason it out like this.

Each circle is 1 whole, and each whole is divided into 2 equal parts. This means each part is $\frac{1}{2}$. There are four parts shaded, so $\frac{4}{2}$.

This is pretty much all we expect from third graders. In particular, we do not expect 8-year-olds to reason about fractions using multiplication. The remainder of this answer is devoted to considering what students will learn in later grades.

---

In grade 4, students learn how to add and subtract fractions with the same denominator, and the operation "$n$ times $\frac{a}{b}$" is introduced. Students at this point can interpret $\frac{8}{4}$ as "$8$ times $\frac{1}{4}$." To go back to the pizza example, "I ate a quarter of a whole pizza eight times."

Then in grade 5, the interpretation of fractions as division, $\frac{a}{b} = a \div b$, and the operation "$\frac{a}{b}$ of $q$" are introduced. As you've said, it's not simple at all to explain why $\frac{8}{4}$ should be the same as $8 \div 4$ and $\frac{1}{4}$ of 8, and this is a major focus of 5th grade mathematics in the CCSS.

Understanding this idea requires children to be able to, within a single problem, change units/re-identify a whole. This is why it's critically important to have third graders go through the process of identifying a whole, aka "unitizing." To illustrate what I mean, here's another exercise from the same workbook.

Write a fraction to name the shaded part of the group.

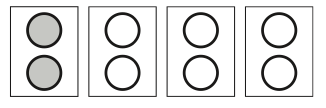
The students are being asked to treat the entire group as a whole. They might interpret the whole as being divided into 8 equal parts, in which case the shaded parts are $\frac{2}{8}$ (of a whole), or they might interpret the whole as being divided into 4 equal parts, and the shaded part is $\frac{1}{4}$ (of a whole). This is $\frac{1}{4}$ of our whole pizza order. On the other hand, if we consider each dot to be a whole, then each dot is divided into one part, and the shaded parts are $\frac{2}{1}$ (of a whole), or 2 wholes. This is 2 whole pizzas.

After the notion of "$\frac{a}{b}$ of $q$" is introduced in grade 5, students learn to say that this is also $\frac{1}{4}$ of 8 wholes. To do this, they have to be able to quickly switch between viewing the entire group as a whole and viewing each dot as a whole. Once they're able to, they can determine that $\frac{1}{4}$ of 8 whole pizzas is 2 whole pizzas. They also know that 2 is the result of dividing 8 by 4. And by dividing each whole pizza into 4 equal slices, they can see that 2 whole pizzas is also $\frac{8}{4}$ whole pizzas. $\frac{8}{4}= 8 \times \frac{1}{4} = 8 \div 4 = \frac{1}{4} \times 8$. It seems innocuous, but this idea is one of the capstones of elementary school mathematics.

Answer 3

Justin H has a good answer emphasizing how fractions are a complex topic and we learn more and more about them over the years. To your specific question, I would emphasize the need to spend a significant amount of time with the less than one fractions before moving to greater than one. Often in questuons here there is a tacit or explicit desire for earlier and more comprehensive explanations. But this is usually a mistake in terms of human pedagogy.

On the specific question, when ready, I would just think of passing out slices of pizza to kids. So five thirds is each kid getting a slice of pizza that is one third of the pie. And yes you need a second pizza. And yes one slice to stick in the fridge.

Also note that your comments about four fifths of 20 or the like are a higher level of problem yet. Since it is of 20. So dont jump to that immediately. And it only works, for neophytes, with common factors

Answer 4

We teach that there are two basic ways of understanding division. På norsk they are called delingsdivisjon and målingsdivisjon; sharing division and measurement division would be rough translations. The actual English terminology might be different.

Sharing division

You have three liters juice and seventeen guests as the birthday. How much is there for everyone to drink? Is it enough, too much, or too little? (To make for an open question that might be interesting in reality, too.)

Measurement division

You have three liters juice and drinking glasses where you can pour 5/3 deciliters juice to. How many glasses of juice can you fill? (And the same bonus question as above.)

I recommend doing these with concrete objects to anchor the mathematical understanding to the real world and operations there.

I am not quite answering the question you asked, but I hope this idea suggests how to move forward also in your case.