Many students think
$\sqrt{a} \sqrt{b}=\sqrt{a\ b}$
$\sqrt{a^2}=a$
$\frac{1}{\sqrt{a}}=\sqrt{\frac{1}{a}}$
but none of the above are true when (a) and (b) are negative.
To avoid such problems, students need to learn the properties below are true for any real* (a, b, c) that meet the conditions specified.
$\ a^b a^c = a^{b+c}$ when $a\neq0.$both sides are defined.
When $a^c b^c=(a\ b)^c$
$a^c b^c=(a\ b)^c$ when c is an integer and both sides are defined.
$a^c b^c=(a\ b)^c$ when (a0 < a < $\infty$) or (b0 < b < $\infty$) is positive.
When $\left(a^b\right)^c=a^{b c}$
$\left(a^b\right)^c=a^{b c}$$\left(a^b\right)^c=a^{b\ c}$ when c is an integer and $a\neq0.$both sides are defined.
$\left(a^b\right)^c=a^{b c}$$\left(a^b\right)^c=a^{b\ c}$ when (0 < a is positive< $\infty$).
$\left(a^b\right)^c=a^{b\ c}$ when (-1 < b $\leq$ 1).
$a^c b^{-c}=\frac{a^c}{b^c}$ for all $a, b, c.$$\ $ a, b, c.
$a^c \left(\frac{1}{b}\right)^c=\left(\frac{a}{b}\right)^c$ when$a^c b^{-c}=\frac{a^c}{b^c}=a^c \left(\frac{1}{b}\right)^c=\left(\frac{a}{b}\right)^c$ when $a$$c$ is positivean integer.
$a^c b^{-c}=\frac{a^c}{b^c}=\left(\frac{a}{b}\right)^c$ when$a^c b^{-c}=\frac{a^c}{b^c}=a^c \left(\frac{1}{b}\right)^c=\left(\frac{a}{b}\right)^c$ when $c$ is an integer and(0 < b < $b$ is positive$\infty$).
* Both -$\infty$ and $\infty$ are excluded because theyThe caveat "when both sides are not real numbers.
Two properties specify $a\neq0$ to exclude casesdefined", excludes couter examples such as
$\ 0^5\ 0^{-2} \neq 0^{5-2}$ and $\left(0^{-2}\right)^{-3}\neq0^6.$
Students also need to know a few things about when the above properties can be extended to complex numbers, but I didn't account for that above.
What books include most of the above properties?
-----Edit------
Actually, I only expect people to learn how some of the above identities extend to complex numbers when they are in certain fields of study. The above properties actually apply when the variables are complex numbers. An electrical engineering student should learn somemost of that before they study Discrete Fourier Transforms, andor Z-Transforms. However, the properties listed above are only slightly more complicated than what students typically learn in Algebra II and Pre-Calculus. Sadly, some books actually tell you the fallacies above are true. One example is the following from: Ron Larson et al, Algebra II, Maryland Edition, McDougal Little, 2008.
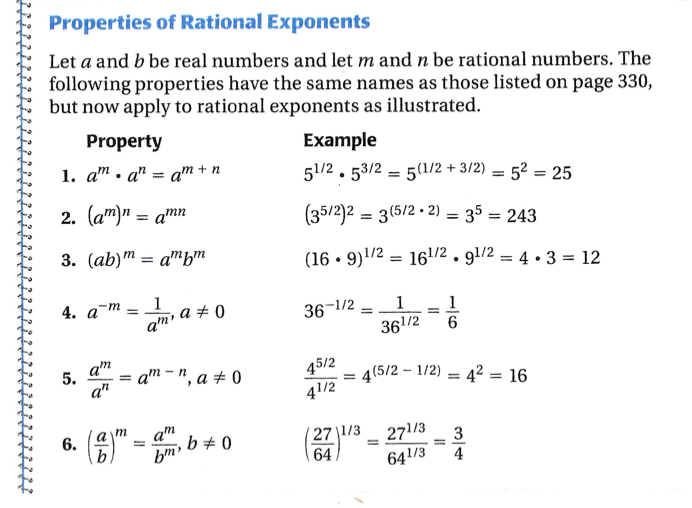 Consider the next example from Daniel Zwillinger, CRC Standard Mathematical Tables and Formulae, CRC Press 1996.
Consider the next example from Daniel Zwillinger, CRC Standard Mathematical Tables and Formulae, CRC Press 1996.
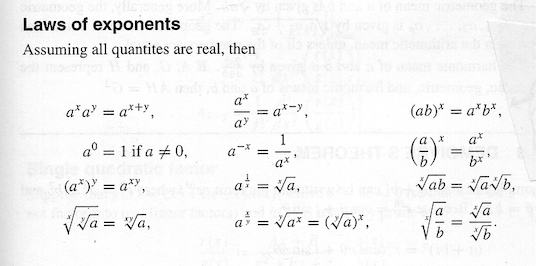
The second example is a math reference for engineers and scientists. It covers elliptical integrals and partial differential equations, but nothing is mentioned about the properties of $a^b$ that leads to complex numbers. Many books only mention how the properties work for integer and rational exponents. What do the authors think people will do when working with approximate exponents having 16 digits of precision? Do they think people will convert the approximate exponents to rational numbers and work the problem from there? Hopefully by the time students take Pre-Calculus they lean that that the properties work in the case of a positive number to a real power.
For those in math intensive fields, the subject should be revisited by the third year at a university. I don't remember what my professors mentioned about the properties of $a^b$, but I can't find a book that covers what I am looking for. I once took a course on functions of complex variables and the text book said nothing about the properties of $a^b$.