9
$\begingroup$

In every algebra (or basic analysis) book that I've seen, three properties of real numbers are taken as axiomatic: commutativity, association, and distribution of multiplication over addition [$a(b + c) = ab + ac$].

What's bothered me for a long time is that while combining like terms [$ax + bx = (a + b)x$] is equivalent to distribution, it seems more basic and fundamental. It's used as an addition process (instead of a multiplicative one); it seems commonsensical in terms of unit addition (3 feet + 5 feet = 8 feet), which was referenced by some as the "great principle of similitude"; and so forth.

So what is the rationale for taking distribution as axiomatic, and proving combination afterward? Why is it not better pedagogy to take combining terms as fundamental, and then prove distribution from it?

I've cross-posted this question on the Mathematics site: https://math.stackexchange.com/questions/1417856/why-is-distribution-prioritized-over-combining

Improve this question
$\endgroup$
10
  • 8
    $\begingroup$ I'm having a bit of trouble parsing your question. Are you asking about why the left-hand side of this equality is typically presented as $a(b+c)$ and the right-hand side as $ab + ac$, whereas presenting them in a switched order seems more natural, to you, pedagogically? As in, you would prefer it written as: $ab + ac = a(b+c)$? (In your examples, you have distribution occurring on different sides of the parenthetical sum, and you've also changed from $a, b, c$ to $a, b, x$...) $\endgroup$
    – Benjamin Dickman
    Commented Sep 5, 2015 at 8:09
  • 7
    $\begingroup$ I think it would be good to emphasize early on that equations work both ways. Some students who remember $(x+y)^2=x^2+2xy+y^2$ fluently don't recognize that $x^2+2xy+y^2$ could be written as a square, especially if $x$ and $y$ are more complicated than a single variable. Too often have I heard people say "it never occurred to me that you could use this identity in that direction". Making students see "$=$" as a symmetric binary relation should make distribution and combining obviously equivalent for the students. $\endgroup$
    – Joonas Ilmavirta
    Commented Sep 5, 2015 at 12:10
  • 2
    $\begingroup$ I think it is probably just a historical accident. As soon as you have the name "distributivity" for the law in question, people will want to write it in the form $a(b+c) = ab+ac$, to demonstrate the $a$ being distributed to the $b$ and $c$. We have a verb "distributing", but using the verb "combining" is less standard. Changing the language will probably be difficult, even if it does make a little more sense. I would also like to write function composition in the other order, but we do not live in a perfect world. But feel free to write the equality the other way when you teach it. $\endgroup$
    – Steven Gubkin
    Commented Sep 5, 2015 at 18:58
  • 2
    $\begingroup$ Steven Gubkin has my meaning; I'm talking about both the order of the presentation and the name itself. Granted that (a) we will take one as axiomatic, and (b) the initial emphasis will be on the left-to-right transformation (and so the name is inherently connected to the initial presentation and application), then it seems that we had two options. Option (1) "distribution" a(b+c) = ac+bc; option (2) "combining" (or collection) ac+bc = (a+b)c. It seems like option (2) is far more intuitive and commonsensical and so easier to digest as an axiom. $\endgroup$
    – Daniel R. Collins
    Commented Sep 5, 2015 at 20:15
  • 7
    $\begingroup$ Changing every instance of $a(b+c)$ to $ab+ac$ (in a polynomial) can be done "mechanically". However, changing instances of $ab+ac$ to $a(b+c)$ requires one to recognize when terms have common factors, and incompatible choices may be involved. (When you have $ab+bc+cd$, either of two combining operations, but not both, can be applied.) Hence the normal form for polynomials (in any number of variables) is the unfactored form. $\endgroup$
    – Alexander Woo
    Commented Sep 7, 2015 at 23:55

3 Answers 3

Reset to default
9
$\begingroup$

This grew a bit long for a comment.

(My first note is similar to Alexander Woo's remark about factoring polynomials; perhaps he intended "polynomials" to subsume the case here, in which we add constant functions...)

Given $413 + 91$, it may not be clear that this can be re-written as $7 \times 59 + 7 \times 13 = 7(59+13)$.

(Plenty of people seem to believe that $91$ is prime; an earlier comment cites J. Conway as observing as much, and I would guess it is related to memorizing the $10\times10$ or even $12\times12$ times tables.)

Meanwhile, $7(59+13)$ can have the $7$ distributed mechanically.

Still, the act of viewing an equation from both perspectives is certainly important, and I think what you observe here is not altogether different from the tendency to write $1 + 1 = 2$ significantly more often than $2 = 1 + 1$, which is known to cause problems with viewing the equal sign, $=$, as an operator, i.e., operationally rather than relationally, cf. MESE 7964 and the nice response of D. Hast.

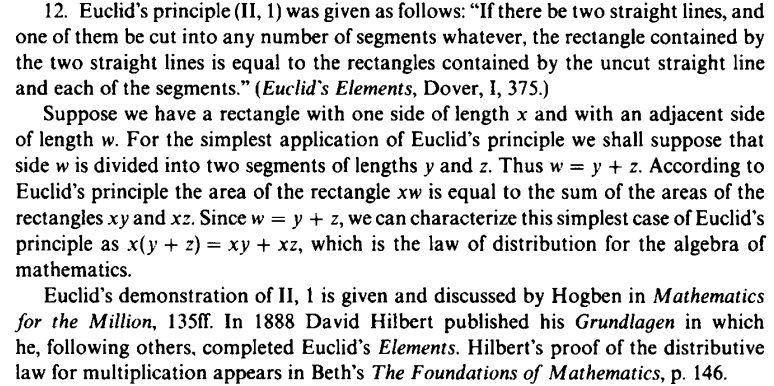
You did ask for some rationale; perhaps we can look historically to Euclid's Elements. If we are to believe the translation provided here, then we have Euclid remarking in the same order. A similar translation is found, e.g., in the following source:

Drucker, T. (Ed.). (2009). Perspectives on the history of mathematical logic. Springer Science & Business Media.

A note from the aforecited:

enter image description here

Finally, a separate but related comment: I observe a prioritizing in the presentation of the difference of squares and their factorization, i.e., more often than not I see: $a^2 - b^2 = (a+b)(a-b)$.

This phenomenon seems to prioritize "combining" over "distribution," and is often accompanied by the same for the sum and difference of cubes. Anyhow, there are consequences in this case, as well. Few students consider such a property in computing, e.g., $47 \times 53$.

(Ask students to compute that product in a few ways and see if it even comes up!)

I feel confident that most important will be for students to see that the same mathematical information is presented by an equality regardless of which expression is on which side, and to give students the opportunity to consider both presentations and their various ramifications.

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ Best response I've seen so far. I'm not sure it answers the "why this way first" question, but it's very thoughtful and the Drucker/Euclid reference is great. Thanks for that. +1 $\endgroup$
    – Daniel R. Collins
    Commented Sep 14, 2015 at 1:59
  • 1
    $\begingroup$ @DanielR.Collins You're welcome! For fascinating remarks on $a^2 - b^2 = (a+b)(a-b)$ and ways of extending this factorization-thinking beyond writing a natural as the difference of squares, see Pomerance's A Tale of Two Sieves (beginning with A Contest Problem on its second page). $\endgroup$
    – Benjamin Dickman
    Commented Oct 27, 2015 at 1:42
  • 1
    $\begingroup$ Certainly the best answer that was given here, so I'm accepting this. $\endgroup$
    – Daniel R. Collins
    Commented Dec 22, 2019 at 21:28
3
$\begingroup$

As the above comments explain, that it is called "distributive law" is just a name. Distributing and combining (factoring) are logically equivalent. The identity can be applied in both directions.

A practical difference is that distributing (multiplying out) can be done mechanically, factoring (combining) often has several potential common factors among different sets of terms, selecting "the right one" isn't always obvious.

That said, it is certainly worthwhile to emphasize that identities work both ways, and factoring complex expressions is obviously an important skill that has to be trained.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Yes, I stipulate in the OP that they are equivalent. The question is why historically "distribution" was picked as the name, and the initial direction, instead of the other option. $\endgroup$
    – Daniel R. Collins
    Commented Sep 12, 2015 at 20:10
2
$\begingroup$

Having an inquisitive, but impatient 3rd grader can help you to get the answer pretty quickly:

Distribution can be explained and applied directly out of the box, because a, b and c are all known when starting on the left. In order to work from the right hand side, the combination requires finding (isolating) the common part (i.e. denominator), which might not be obvious on the spot, especially when starting with numbers as commented by Alexander Woo.

This is unneccessary distraction while trying to explain this equivalence on its own, so more or less knowingly, the explanations prefer distribution over combination.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.