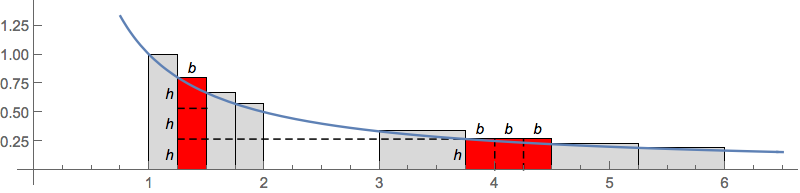
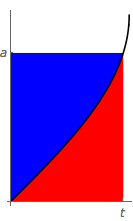
I'm designing a lesson for an Introduction to Integral Calculus class, and I want to encourage students to evaluate integrals without just going straight for the antiderivative and using the fundamental theorem of calculus. I want them to think geometrically about the situation before diving in with computations. Here are a few general topics I've thought to include so far:
Integration of even and odd functions.
Integration of functions with graphs that are familiar shapes. Like $\int_{-2}^2 \sqrt{4-x^2}\;\mathrm{d}x$.
Maybe integrating a function without an nice antiderivative on the domain of integration, like $\int_{-a}^a |x|\;\mathrm{d}x\,$? But I would want the function so be more interesting that just $|x|$.
What are good examples of integrals that illustrate the ideas above and would work well in a lesson? Or better, does anyone have other ideas for topics that I should (or shouldn't) include in this lesson?