7
$\begingroup$

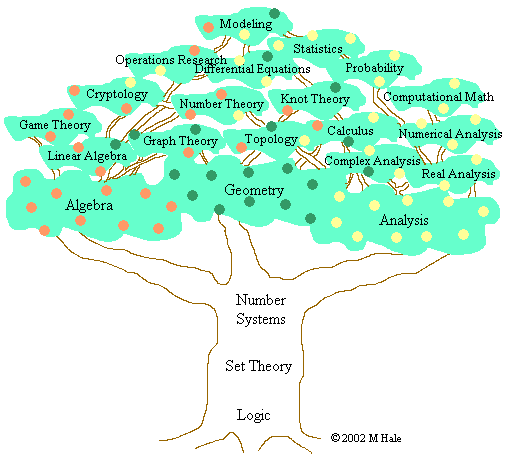
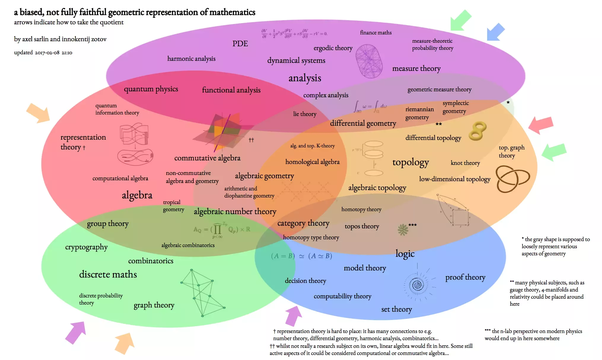
If you were to "map" mathematics onto a tree structure where the top is "Mathematics", and then below it the different branches, then sub-branches, etc.

What do you suggest is a good structure, for educational purposes? The idea is this - if I have a student learning a topic, then all I would have to do is look at all the sub-branches (and theirs) below that topic in the tree, to represent everything the student needs to know.

I'm certain there will be overlaps, but I'm okay with this - looking for some insight on this, or relevant articles.

If you think that such a hierarchy does not even exist, please let me know what you think the right structure is for educational purposes, and if you know where to find it.

Many thanks!

Improve this question
$\endgroup$
6
  • 15
    $\begingroup$ I would not map mathematics in such a hierarchical structure. $\endgroup$
    – Xander Henderson ♦
    Commented May 20, 2020 at 11:58
  • 5
    $\begingroup$ mathscinet.ams.org/msc/msc2010.html $\endgroup$
    – Henry Towsner
    Commented May 20, 2020 at 14:02
  • 5
    $\begingroup$ @AmirHardoof Because I do not believe that mathematics has a hierarchical structure. Different parts of mathematics overlap and communicate with each other in innumerable ways. If we imagine for a moment that mathematics can be discretized and given a graph structure, that graph would no doubt contain a tremendous number of loops. $\endgroup$
    – Xander Henderson ♦
    Commented May 20, 2020 at 17:27
  • 1
    $\begingroup$ If you pick a very small piece of mathematics, "commonly-taught school precalculus," then developers of online homework systems have actually tried to create such a hierarchy. The hierarchy is hard to determine, core to their business model, and not publicly released. Essentially even if you believe this exists for small areas of math, it is very difficult and expensive to find out the tree structure. $\endgroup$
    – Chris Cunningham
    Commented May 20, 2020 at 20:15
  • 1
    $\begingroup$ I believe something like what you’re suggesting is the most important aspect of course design: that someone designing a course should have in mind exactly what the prerequisite knowledge assumed is, and each lesson should build upon that with specific lesson objectives that build up to the course outcomes. Every assessment in the course should only require concepts that were prerequisites or lesson objectives. Unfortunately, I think many course designers don’t spend enough time thinking the prereqs for their course, let alone specific objectives for every lesson. $\endgroup$
    – Joe
    Commented May 21, 2020 at 16:10

3 Answers 3

Reset to default
7
$\begingroup$

(1) Here is Margie Hale's tree:

HaleTree

(2) And here is Gaspard Sagot's hierarchy:

SagotHier

Improve this answer
$\endgroup$
0
1
$\begingroup$

Here's an interesting attempt in both text and interactive images. Not a hierarchy but rather a "map."

The Map of Mathematics. A project by Quanta Magazine. Text by Kevin Hartnett. Design and visualizations by Kim Albrecht and Jonas Parnow. Feb. 2020. Quanta link.

         
          The Riemann zeta-function.

The top-level map is here. (Need to scroll a bit and click on $\fbox{The Map}$.) Hover over a topic to see connections between fields.

Improve this answer
$\endgroup$
-1
$\begingroup$

I'm not sure that a complete hierarchical categorization of math is possible even if we ignore the incompleteness therom. For example arithmetic can be derived from lambda calculus or geometry or incrementation, recursion and inversion; but I learned those subjects from teachers who assumed I new arithmetic and this is just a simple case.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.