Edit (May 2016): From The Atlantic is:
Boaler, J. & Chen, L. "Why Kids Should Use Their Fingers in Math Class." Apr 2016. Link.
"Evidence from brain science suggests that far from being “babyish,” the technique is essential for mathematical achievement."
(See the link for more!)
Counting is one of the most important activities to engage in with young children; it is also one of the key precursors for developing number sense later on. If you are looking to help young children with their mathematical development, then I would say that the single best choice is to count with them (another important activity is to practice using words meaningfully, e.g., identifying different shapes). If you are wondering about how to help them count, the standard approach is to begin with the number chant (it is pretty tough till around 13, and then gets a bit easier in the later teens, and then goes rapidly from 20 on); next, move on to skip counting (by 2s, by 5s, by 10s); and counting in reverse order.
As to the question here, there is a lot written about the subject. For example, Gelman writes here:

The real reason to post such an excerpt is to indicate how complex a task this is!
You can find some good work on counting by searching for AJ Baroody and HP Ginsburg.
If you are not so keen to read through the literature yourself and would just like a quick answer, here is the best that I can do (though, I reiterate, counting is not so simple!): The ultimate goal is for children to be able to count without needing to use their fingers; however, in building up to that goal, it is totally fine for them to count using their fingers. Keep practicing the chanting, the skip counting, and then try counting groups of objects! It is fine for children to tap each object when they are counting them; later on, they might just point; later still, they may be able to count without needing their fingers; ultimately, the goal is to count without even having the objects in front of themselves. But the time that this takes can vary.
(Note about multiplication and finger counting: Later on, when students move on from basic facts about addition to "basic facts" about multiplication, i.e., multiplying pairs of whole numbers from 1 to 10, they can still use finger counting to help them: especially with the skip counting numbers of 2, 5, and 10. For example, one way to compute 2x8 is to hold up 8 fingers, and then count on them by 2s (2, 4, 6, 8, 10, 12, 14, 16), putting down a finger each time it has been used. Again, the ultimate goal is to move towards abstraction and not needing a concrete representation, but using fingers to count - for addition or for multiplication, which can be thought of at that age as repeated addition - can be very helpful.)
Perhaps one of the stressful factors is not knowing what the norms are for a given age (or stage). The language that Jamie is using looks totally age-appropriate; my bigger concern would be that his tutors are not quite sure how to elicit his reasoning. For example, the response of because you said that looks very familiar if you have spoken with children about mathematics. One good way to try to understand their thinking is by using clinical interviews; a single particular technique is to say something like, what if a little kid asked you to explain your thinking? Rather than delve deeply into techniques for using the clinical interview, I will give the same recommendation I included in earlier answers here and here (see the very end of each):
Ginsburg, H. P. (2009). Early mathematics education and how to do it. Handbook of child development and early education: Research to practice, 423-428.
Finally, if you would like to know a bit more about norms for kids learning mathematics at an early age, I recommend the following book (or its 1989 reprinting):
Ginsburg, H. (1977). Children's arithmetic: The learning process. D. van Nostrand.
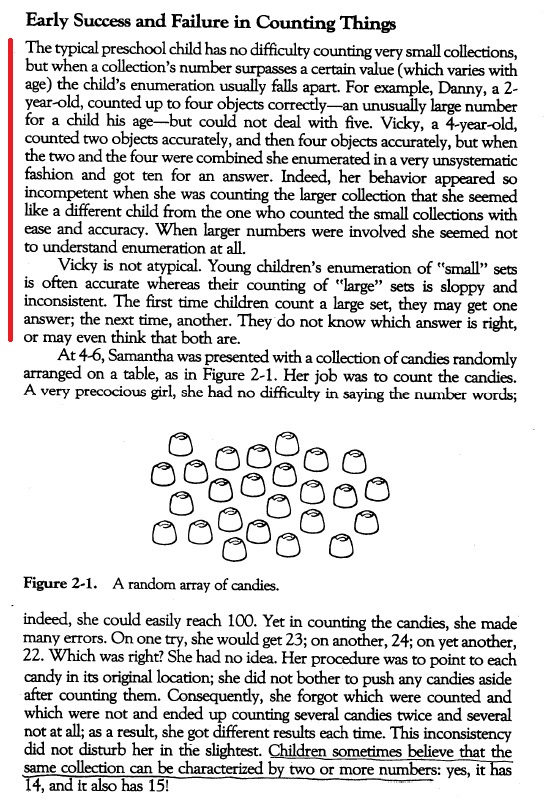
Here is an excerpt from Chapter 2, Learning to Count, to give you an idea around norms: