Explanation for cutting a Möbius strip at one-third its width

Question

Can anyone offer a concise, convincing explanation for why cutting a Möbius strip along a line, not midway but rather one-third of the width of the strip, and eventually joining back to itself, produces two linked, twisted loops, one long, one short:

---

^{(Image from this web site.)}

---

I am not seeking a proof, but rather an explanation that could convince essentially anyone paying sufficient attention. Especially the lack of symmetry in the result can be surprising.

Consider it a teaching challenge. :-)

Answer 1

The middle third is obtained by trimming the edges off the original möbius loop. It is therefore simply a thinner möbius loop (the short loop).

The outside thirds of the möbius loop are obtained by cutting the loop in half and trimming 1/3 off the edge that was not originally the outside edge. It is the same as cutting the strip in half: as the outside edges are linked by a twist, the edge is a single loop twice as long as the original.

Imagining that a wire runs along the centre of the original möbius loop, one can follow the path of the outside edge as you trace along the wire. The edge slowly twists around the inside loop, so that after following the wire for 2 rotations, the edge has made a complete loop around the centre of the Möbius strip, going through the middle of the wire loop. As the wire becomes the small Möbius strip, and edge becomes the long Möbius strip, the long strip loops itself once around the small strip.

Answer 2

Alternatively, a pictorial explanation can be used (perhaps more effectively)

Answer 3

The outer two-thirds are the same piece; the inner third is a different piece from the outer two-thirds.

There have to be two different pieces, because at all points along the cut line, the cut is separating a piece that "contains the centerline" from a piece that "contains the original edge".

Because both edges are the same edge, the outer two-thirds form a single piece.

Because the outer piece has twice the area as the inner piece, but has the same width, it must be twice as long.

Answer 4

A nice way to think about the result is to take a long strip of paper, cut it into three pieces, and then twist and join them. This results in the same configuration as cutting it after twisting and joining, but it lets you track each strand individually.

Answer 5

To explain the linking, consider three long parallel pieces of string attached to a long rectangular piece of paper. Joining the edges in a cylindrical fashion results in three disjoint loops, while using a Moebius twist joins two of the strings together, and does so in a linked fashion to the loop created by the middle string. From this image it is not too hard to switch to your ribbon model and count the number of twists in each ribbon. Perhaps someone will be kind enough to provide an image to illustrate.

Gerhard "Twist Your Brain To See" Paseman, 2015.02.12

Answer 6

A complete cut of a strip (= tape jointed to itself) is a cut which forms a loop in the strip interacting one, two, three… times with each ‘parallel’ of the strip. Namely a cut with one, two, three,... turns.

A complete cut of a strip individuates a geometrical object adding, at each turn, the number of edges of the initial strip.

Thus, for instance

• a complete cut with a turn of a strip with two edges generates an object with 2+2 edges, namely two strips with two edges,

• a complete cut with two turns of a strip with one edge (such as the Möbius strip) generates an object with 1+1+1 edges, namely a strip with two edges and a strip with one edge.

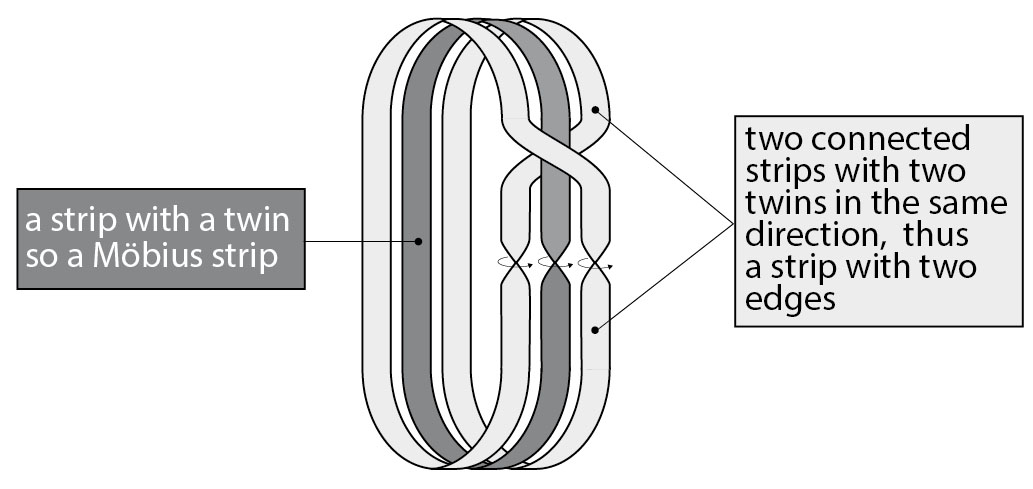
Since a ‘one third’ cut of a Möbius strip, to be complete, must turn twice around the strip, dividing the strip in two parts, this cut necessarily individuates a strip with two edges and a Möbius strip.

Then the two external parts individuated by the cut must be connected and must form a strip with two edges.

So, this cut separates the central part of the original Möbius strip from its external part, which is given by the conjunction of two strips as long as the central part.

Finally, since the edges of these strips are interlaced, the two resulting strips are linked.

NOTE. It is interesting that when the internal part disappears, the cut becomes a cut with a turn which individuates only the external part (with two edges).