Interesting Physical Implements for the Classroom

Question

I'm interested in gathering a list of physical objects of mathematical interest for occasional or permanent display in a classroom. Mostly I'm interested in things that are wall-mountable, but any physical objects will do.

Answer 1

Two things that I had in mind, which prompted the question in the first place, are:

A Pin Board

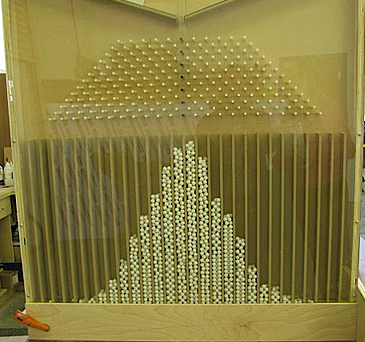
_{(source: ptrow.com)}

This device demonstrates the emergence of normally distributed data from a large number of independent, random events applied in series.

Image from http://ptrow.com/articles/Galton_June_07.htm

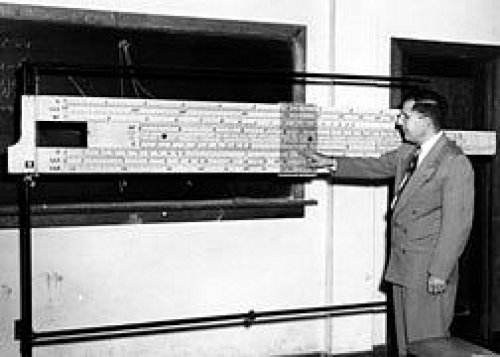
An Adding Machine

I'm currently building one of these beauties. This machine serves as an excellent starting point for binary representation of numbers, mechanized computation in general, etc. Students can gain an appreciation for the notion that computers working in binary is primarily an engineering decision (eg, it's easier to make a toggle that has 2 positions than any other number).

Image and machine plans from https://woodgears.ca/marbleadd/index.html

Both of these pieces are beautiful, loud, mathematically rich, and quite a bit of fun to watch. Their presence will stir curiosity and discussion.

Answer 2

I think polyhedra and polyhedra-like objects are, although not exactly wall-mountable, quite pleasant to have around.

Polyhedra are particularly easy to build with zometool materials (a professor of mine always had a complete set of zometool Platonic Solids laying around in his office; they were great), as this picture (from the zometools website) shows:

Of course, you don't need fancy struts and joints, as (preferably nice cardstock) paper will suffice, and opens up whole new realms of possibility, as George W. Hart shows:

The latter have the advantage of being light enough to be hung from the ceiling easily and don't need a file cabinet or anything to rest on. I've built a few of his 'slide-together' structures, and they're marvelous. (Note: The above image is the object obtained from rotating each face of an icosahedron by, I believe, 60 degrees, as demonstrated in this Wolfram Demonstration).

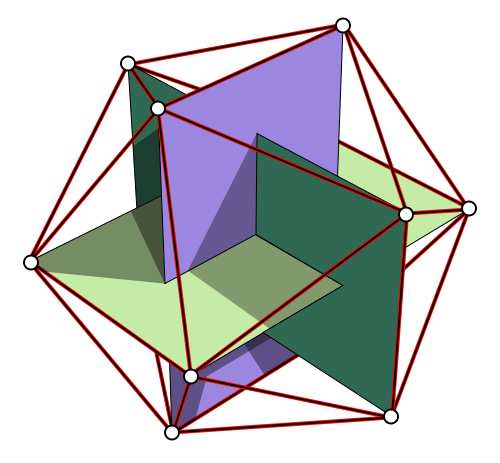
The icosahedron can also be obtained by fitting three golden rectangles together. This is also fairly easy to implement physically, though I haven't decided how to include the edges (and it's kind of more fun to hold the three interlocking planes and convince yourself that you're looking at an icosahedron).

And, of course, who doesn't want to have a dodecahedron, soccer/football, and golf ball around, as evidence that all polyhedral objects with only regular hexagonal and pentagonal faces, with three meeting at each vertex, must have exactly 12 pentagons?

Answer 3

You could hang posters of the 17 wallpaper groups, or the 230 space groups, hoping their presence will engender wonder and generate questions.

---

         
          ^{(17 Wallpaper Groups.)}

---

         
          ^{(230 Space Groups.)}

Answer 4

How about a Slide Rule:

Also some wolfram posters: or: