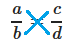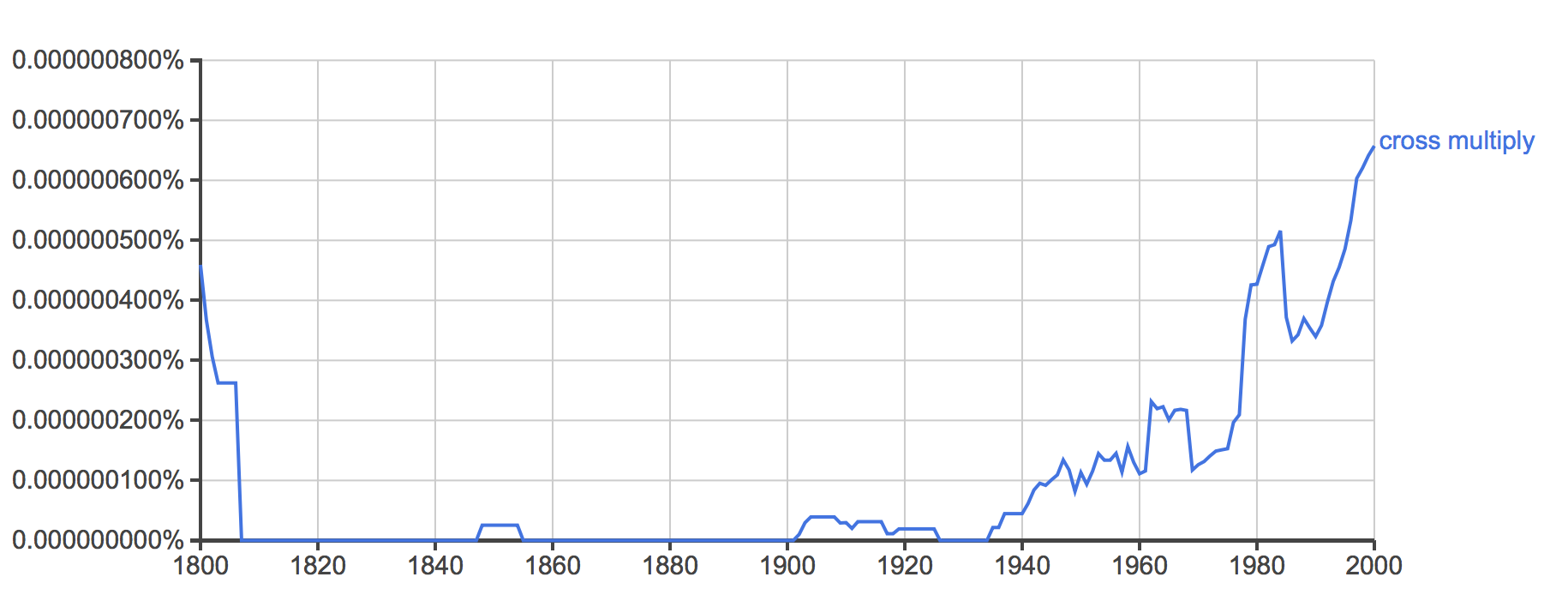9
$\begingroup$

The title says it all, I suppose. I'm interested to know when/where the term/technique cross multiply came into use. Sources would be nice.

In case it's unfamiliar to anyone, or in case the usage of the term varies, I'm referring to the compound arithmetic operation where an equation of two fractions is multiplied on both sides by its two denominators. It's named because it can be illustrated with a big cross:

$$\frac{a}{b} = \frac{c}{d}$$ The cross! $$ad = cb$$

Improve this question
$\endgroup$
5
  • 3
    $\begingroup$ According to link it was early 1950's $\endgroup$
    – Amy B
    Commented Nov 20, 2015 at 2:45
  • $\begingroup$ Ah, the old fashioned dictionary.com $\endgroup$
    – NiloCK
    Commented Nov 20, 2015 at 2:47
  • 3
    $\begingroup$ A quick check of google scholar suggests: <Short, R. L. (1939). Methods in Arithmetic and Algebra. School Science and Mathematics, 39 (3), 239-250.> as a possible source. ("To prove this, just cross multiply numerators and denominators") One issue is that the term cross multiplication had a different meaning before its use in solving proportions... $\endgroup$
    – Benjamin Dickman
    Commented Nov 20, 2015 at 3:03
  • $\begingroup$ Aren't methods for solving proportions found even in the Rhind Papyrus? Or is this question merely asking when the name "cross multiply" was used for it? $\endgroup$
    – Gerald Edgar
    Commented Nov 20, 2015 at 15:23
  • $\begingroup$ @GeraldEdgar Certainly people have been solving these problems for a very long time. I'm interested in the particular term, and more specifically in its emergence as a pedagogical tool using the mnemonic of the cross. $\endgroup$
    – NiloCK
    Commented Nov 20, 2015 at 19:06

1 Answer 1

Reset to default
8
$\begingroup$

The earliest example of the OP's use I can find on Google Books is 1908. It was also used to describe adding fractions about the same time (although there is a use in a professional mathematics article in 1833). It starts to be used often in the late 1930s and shoots up again in mid 1970s.

Some early references to school mathematics from Google Books:

Earlier uses of the term in professional articles. They seem merely descriptive, although the usage to describe multiplying number systems with place values seems common (ones-tens-hundreds, feet-inches-parts). The 1833 paper uses the term to describe an operation in simplifying an fairly complicated algebraic fraction.

Ngram viewer results:

enter image description here

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ This is excellent, thank you. I followed the link but am still unsure - do the percentages in that scale refer to the percentage of books which use the phrase? $\endgroup$
    – NiloCK
    Commented Nov 20, 2015 at 14:26
  • 1
    $\begingroup$ +1, the third example is very nice, though the pointer goes to p. 149, which refers back to the earlier definition on p. 138. There, one can find the method stated clearly in the context of solving proportions (jpg). $\endgroup$
    – Benjamin Dickman
    Commented Nov 20, 2015 at 23:43

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.