4
$\begingroup$

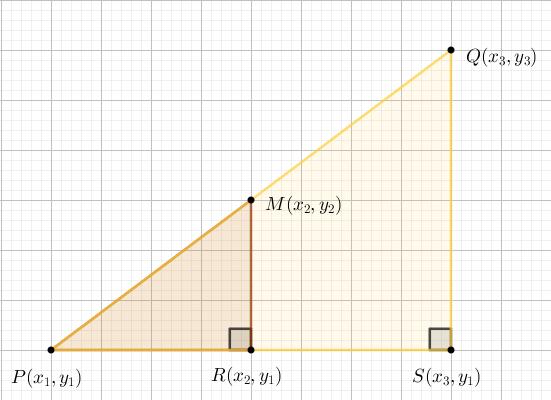
I've had to try to explain the following problem:

Let $PQ$ be a line segment, given $P(x_1, y_1)$ and the midpoint $M(x_2, y_2)$, find the coordinates of $Q$.

I always draw a diagram and draw $\Delta x$ and $\Delta y$ and explain why they are $x_2 - x_1$ and $y_2 - y_1$ respectively. I then explain that you have to add the differences to move from the midpoint to the other endpoint. This always confuses the kids. Should I introduce the idea of a vector? What would you suggest to help them understand this procedure?

Improve this question
$\endgroup$
1
  • 3
    $\begingroup$ I recommend at that level: follow the textbook very closely, and do not introduce anything extra (like vectors). $\endgroup$
    – Gerald Edgar
    Commented Sep 18, 2017 at 20:51

4 Answers 4

Reset to default
7
$\begingroup$

Putting aside the two-dimensional question for a moment, consider the corresponding one-dimensional question:

You are given two numbers $p$ and $q$. $p$ is $5$. The average of the two numbers is $8$. What is $q$?

If you ask the class (or yourself), you might get multiple different approaches. For example, you might go with

Well, it is $3$ units from $5$ to the middle. So it must be $3$ more units from the middle to the other side. So the answer is $q = 8 + (8-5) = 11$.

Or perhaps:

I don't know what $q$ is, but I know how to find the average of $p$ and $q$. The average is $8 = \frac{p + q}{2}$. Plugging in $5$ for $p$ lets me solve for $q$, and $q$ comes out to 11.

Then you can decide between:

  • Choose the first method as the official method and explain its 2D analog to the class.
  • Choose the second method as the official method and explain its 2D analog to the class.
  • Express the idea that both methods are fine, there is no such thing as an official method, and show both 2D analogs, and let everyone do what they think is easiest as long as they show what they are doing.

I don't know which of the three will work best in your classroom environment, but whatever your approach is to the easier 1D problem, you should go with the same thing in 2D.

Improve this answer
$\endgroup$
1
  • $\begingroup$ I have noticed that different students use similar approaches to "Find the midpoint". Some will find the average (mean) others add half the difference to the smaller. (I don't recall seeing one subtract half the difference from the greater number.) $\endgroup$
    – Jim H
    Commented Sep 18, 2017 at 16:13
4
$\begingroup$

Let $Q(x_3,y_3)$. Using the midpoint formulas $x_2=\frac{x_1+x_3}{2}$ find $x_3$.

Improve this answer
$\endgroup$
1
  • $\begingroup$ That could definitely help students who are comfortable with basic algebra but have a hard time thinking geometrically. $\endgroup$
    – John Coleman
    Commented Sep 18, 2017 at 10:54
3
$\begingroup$

Assuming the students know the principle of similar triangles, one might use the following approach:

Midpoint & similar triangles

$$ \triangle PMR\sim\triangle PQS$$

We know that $\dfrac{PQ}{PM}=2$.

Therefore by the property of similiar triangles we know that

$$ \frac{PS}{PR}=2 \text{ and }\frac{QS}{MR}=2$$

Therefore

$$ \frac{x_3-x_1}{x_2-x_1}=2 \text{ and }\frac{y_3-y_1}{y_2-y_1}=2$$

Solving these two equation for $x_3$ and $y_3$ gives

$$ x_3=2x_2-x_1 \text{ and }y_3=2y_2-y_1$$

This can be checked by verifying that

$$ \frac{x_1+x_3}{2}=x_2 \text{ and }\frac{y_1+y_3}{2}=y_2$$

Improve this answer
$\endgroup$
2
  • $\begingroup$ My first reaction was that this was hilariously overcomplicated, but it uses some very generalizable skills that students in early math and science need to practice anyway (creating right triangles on their own, labeling points on a diagram with their coordinates as a habit), so I do not think it is so unreasonable after all. $\endgroup$
    – Chris Cunningham
    Commented Sep 18, 2017 at 20:33
  • 1
    $\begingroup$ @ChrisCunningham Usually, the midpoint formula is justified by an argument using similar triangles. Assuming the students had been convinced by that argument but not convinced by the algebraic argument for finding the endpoint based on the derived mid-point formula, going back to an argument based on similar triangles might persuade them. Some students are better convinced by geometrical arguments. $\endgroup$
    – John Wayland Bales
    Commented Sep 18, 2017 at 20:38
2
$\begingroup$

Vectors are a natural way to think about the problem, but if the students are struggling with this problem then explicitly introducing a whole new abstraction might confuse them even more.

You could perhaps sneak in a vector intuition without explicitly defining vectors: instead of adding the differences to the midpoint, add twice the differences to the starting point, describing it as going twice as far from the starting point in the same direction as before. Even without a formal notion of vectors, students should have some understanding that slope determines direction. You could even draw arrows in the diagram to illustrate the situation. As an added benefit, this might prime the pump for subsequent work with vectors.

Improve this answer
$\endgroup$
1
  • $\begingroup$ I taught 9th grade Algebra and utilized both method mentioned already (by whatever and by you). I would say that this geometric approach is much more intuitive since students can easily count squares while others had trouble solving for x or understanding what the x that they solved for represented. The geometric approach was much nicer since it segued into "rise-over-run" for the slope. Just count the rise over run from start to midpoint, then do it again to get to the endpoint. $\endgroup$
    – ruferd
    Commented Sep 18, 2017 at 12:31

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.