Could this visual explanation of horizontal shift be helpful ? ...( if not beautiful...)

Question

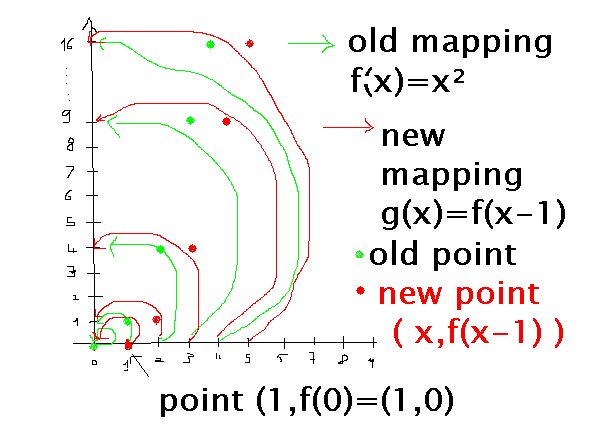
With the image below I try to explain in which way substituting (x-a) ( with a> 0) for x in the expression defining a function results in a shift to the right, although " intuition" tells us it should result in a shift to the left. To do this I use the auxiliary idea of "shifting" as "copying" or "imitating".

I would be interested in knowing whether this explanation could be efficient in the classroom at the high school level.

Suppose we have $y=f(x)=x^2$ and want to graph $y=g(x)=f(x-1).$

The function $g$ maps each $x$ to the image of $(x-1)$ under the function $f$.

In other words, each $x$ value has an "$ x-1$ " ( his own "$x-1$" ) , and copies his (x-1) 's image under the "old" mapping $f$ .

Since each "imitator" ( each $x$ value) is to the RIGHT of it's "model", that is it's " $x-1 $" , the change from the function $f(x)=x^2$ to the function $ g(x)=f(x-1)$ results , for each "old" point of the graph of f, in a translation of $1$ unit to the RIGHT.

Remark. - I first used the idea of " stealing" , for which I substitute the idea of " copying" or "imitating". I've just seen a post of Hyperpallium using the idea of " sampling" , even better.

Link to Hyperpallium's post : https://math.stackexchange.com/questions/2813164/seeing-why-horizontal-shifts-are-reversed

Answer 1

I tried two other ways along the graphical representation you are proposing.

1. A lookup table:

In the first line note some values for $x$: -2, -1, 0, 1, 2

In the second line note the corresponding values of $f(x)=x^2$: 4, 1, 0, 1, 4

In the third line note the corresponding values of $g(x)=f(x-1)=(x-1)^2$: 9, 4, 1, 0, 1

Fill them out together with the pupils and let them observe that all the values of $g(x)$ are shifted by one to the right. Let them think about why this is the case and how they can get the values of the third line from those of the second line.

2. A function with some particular "feature" like the extremum of a parabola or the first extremum or zero of a sine. Say, in the parabola case, to get the extremum you need to plug in $x=0$ into $f(x)=x^2$. So which value of $x$ needs to be plugged in into the shifted parabola $g(x)=(x-1)^2$? Obviously, a larger one to compensate for the 1 which is being subtracted before the squaring comes. Therefore, we will get the extremum at $x=1$, shifted to the right.

Both methods can (and probably should) be used together with the graphical representation as in the original question.