15
$\begingroup$

My first grader is very advanced in math. Rather than doing more and more math and making school math even more boring for him, I recently decided to start going "deeper" rather than "faster."

Some of the questions we've explored recently are:

  • Why do we use a numbering system? (Because otherwise we'd need an infinite number of names)
  • Why is multiplication commutative? (Because you could switch the number of items and the number of people and you'd still need the same number of items in the 'rectangle')
  • Why are 2, 5, and 10 the only numbers whose divisibility tests only need the last digit? (because they're the factors of 10)
  • Why is a number divisible by 9 if its digit sum is divisible by 9? (becuase every power of 10 has remainder 1 when divided by 9)

Are there any resources I can use to find more questions/explorations like this?

Improve this question
$\endgroup$
12
  • 2
    $\begingroup$ How about polygons, polyhedra, etc.? My algebra professor brought in an icosahedron, and I managed to make my own out of magnetiles. You can build up from tetrahedron, to cube, octahedron,... Specifically, the question to pose is: what regular polyhedra can be made? $\endgroup$
    – David Raveh
    Commented Oct 29, 2023 at 10:21
  • 2
    $\begingroup$ School systems vary a lot over the world, can you indicate how old a "first grader" is? $\endgroup$
    – user132647
    Commented Oct 30, 2023 at 6:52
  • $\begingroup$ There are social, psychological & historical "reasons" "why" things in those realms have come about but a proof is not "why" something is a consequence, there is no such "why", only "demonstrations that" something is so. The only reasonable "why" a theorem is true is always the same--it is a consequence of assumptions/givens. $\endgroup$
    – philipxy
    Commented Oct 30, 2023 at 8:52
  • 1
    $\begingroup$ "Why do we use a numbering system? (Because otherwise we'd need an infinite number of names)" That's not a good reason for using a numbering system. In fact, I'm not sure your reason has any explanatory power. The same goes for your second question: your reasoning for why it is the case is poor/lacking. $\endgroup$
    – Adam Rubinson
    Commented Oct 30, 2023 at 11:33
  • 2
    $\begingroup$ @AdamRubinson It's not reasoning: it's the summary of the conclusion of what was probably a several-minute-long discussion. $\endgroup$
    – wizzwizz4
    Commented Oct 30, 2023 at 14:20

18 Answers 18

Reset to default
17
$\begingroup$

Here is a game I stole from Marty Weissman:

You start at zero on the number line. You are allowed "hop" forward or backwards by $6$ and "jump" forwards or backwards by $10$. Which numbers can you reach? Are there any numbers you cannot reach?

Variations:

  1. Change $6$ and $10$ to other numbers.
  2. Use "hop", "skip", and "jump" (3 numbers) instead of just two.
  3. Now you are only allowed to hop or jump forwards, never backwards. How does that change the numbers are you able to reach?
Improve this answer
$\endgroup$
7
  • $\begingroup$ I wonder if #3 would be a simpler starting point to build off of. $\endgroup$
    – hodale
    Commented Oct 30, 2023 at 12:49
  • 1
    $\begingroup$ What is the difference between "hop" and "jump"? $\endgroup$
    – d-b
    Commented Oct 30, 2023 at 13:31
  • 2
    $\begingroup$ @d-b to me it's obvious.. a hop is 6 and a jump is 10 :) $\endgroup$
    – CGCampbell
    Commented Oct 30, 2023 at 13:47
  • 3
    $\begingroup$ @hodale The solution to #3 is harder than the solution to #1. $\endgroup$
    – Steven Gubkin
    Commented Oct 30, 2023 at 14:44
  • $\begingroup$ @CGCampbell So you can reach odd numbers but you can reach all even numbers? $\endgroup$
    – d-b
    Commented Oct 30, 2023 at 19:22
8
$\begingroup$

Following up on David Raveh's comment, geometric manipulatives would offer a wealth of interesting questions.

(1) Polydrons.

PolydronsPlatonic

(2) Snap / Linking cubes.

SnapCubes

(3) Tangram puzzles

Tanagrams

Improve this answer
$\endgroup$
7
$\begingroup$

When I was around this age (I dont remember exactly when, but I could read but only slowly) my parents introduced me to a book "What Is the Name of This Book?" by Raymond M. Smullyan. It is a colection of progressivly more complex logic riddles in the likes of: A says "all of us are lying". B says "exactly one of us is truthful". C doesn't say anything.

I remember finding it really fun at that time. Formal logic is usually taught much later in math curriculum, but I find it not necessary to postpone, since it felt to be very natural and intuitive. Looking back the very early exposure to evaluating statements as true and false and learning to explicitly think about contradictions and implications has helped me in seeing underlying logical structure in many math problems later on.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Starting to read happens at the age of six. Are you saying that you solved "Who is lying" riddles at the age of six? (I would say that even for fourteen-year olds, this might already be advanced) $\endgroup$
    – Dominique
    Commented Oct 30, 2023 at 10:48
  • 3
    $\begingroup$ I was slow reader for several years. It was one of the first books I was wiling to i read, because it provided a lot of information/fun per number of words. I am saying these riddles are not as advanced as they seem. Only prerequisite is understanding language and concept of lying. $\endgroup$
    – BagiM
    Commented Oct 30, 2023 at 13:32
  • 1
    $\begingroup$ I agree that formal logic should be introduced way earlier in the curriculum. Actually all the math that doesn't have a ton of pre-reqs could be introduced way earlier. I suppose this is in some sense AOPS's business model with their books $\endgroup$
    – Sidharth Ghoshal
    Commented Oct 30, 2023 at 15:25
6
$\begingroup$
  • Matte-LIST is one resource we suggest to our students. In Norwegian. https://www.mattelist.no/
  • I think some of the tasks there are from Youcubed, but I have not looked deeper into it. In English. https://www.youcubed.org/tasks/
  • Tall og tanke aktivitetsbok has exercises for teacher students, but many of them are also suitable for children, or imply such. In Norwegian.
  • Relevant books by Jo Boaler have nice, open exercises, that might be suitable. In English.
  • In general, any good books on mathematics didactics or mathematics education in general are worth a look. They should have useful principles and also often activites.
Improve this answer
$\endgroup$
6
$\begingroup$

As a child I was fascinated with numbers. As part of our fourth-grade curriculum we were familiarized with conversion to and from, as well as performing basic arithmetic in, different bases. I remember having fun with that and working on some additional problems on my own beyond assigned homework.

I clearly recall that the bases covered were both smaller and larger than ten. I would claim that this does improve mental flexibility and provides a bit deeper insight into the nature of positional number systems. It even has some practical relevance in the world of computers, but that part I was not aware of at the time. Obviously computations base-60 are common when dealing with time, and here one might add a bit of mathematical history by mentioning the use of the sexagesimal system by Sumerians and Babylonians.

Improve this answer
$\endgroup$
5
$\begingroup$

NRICH has a lot of resources. I usually go through adding 1 to the number in the URL until I find an interesting-looking problem, like Geoboards or Cereal Packets.

Here's an extract from the end of Clock Hands:

If you are the sort of person who prefers to work with a clock that the hour hand moves properly with the minutes hand then you might like to look at these:-

The hands on the clocks are replaced with squares (the squares' diagonals as the clock's radius), equilateral triangles (the altitude as the radius) and circles (the diameters are radiuses). The clocks show 10 past 12, 20 past 12, half past 12, 20 to 1 and 10 to 1 for each shape, for a total of 15 clocks.

Good luck.

Improve this answer
$\endgroup$
3
$\begingroup$

Some more:

  • Why does division by zero not work?

  • Why is the order of operations important in math?
    (Necessity for a standardized order to get consistent results)

  • Why are there infinitely many prime numbers?
    (The proof is actually simple enough for a first grader to understand)

  • What does it mean for a number to be "irrational"?
    (Discuss how some numbers can't be neatly expressed as a fraction. You don't have to use the vocabulary of "irrational.")

  • What is a negative number?
    (Discuss the concept of "less than zero" and where it’s useful. For instance, instead of asking "What is the sum of 10 plus 10?", you could ask "The sum is 20. What could the added numbers be? Could they be negative?")

Improve this answer
$\endgroup$
6
  • 2
    $\begingroup$ "The proof is actually simple enough for a first grader to understand" uhhhh... what? Proofs? For a first grader? That uses contradiction? I have a hard time believing first graders can understand it. $\endgroup$
    – Passer By
    Commented Oct 30, 2023 at 7:26
  • $\begingroup$ @PasserBy Agreed. Without understanding divisibility, it's hard to imagine understanding primes. There may be some dozens of first graders who can understand the proof, but that statement does not support OP's assertion. I suspect that OP just used "first grade" as a stand-in for "surprisingly young". $\endgroup$
    – Ben I.
    Commented Oct 30, 2023 at 14:25
  • $\begingroup$ @PasserBy @ BenI. It certainly depends on the individual child, but it is possible to explain without notation / terminology. But yes, it may be a bit challenging depending on the person's background. OP said the 1st grader is "very advanced in math." $\endgroup$
    – BigMistake
    Commented Oct 30, 2023 at 14:30
  • $\begingroup$ @PasserBy The proof that there are infinitely-many prime numbers isn't by contradiction. "Suppose we have some prime numbers. Multiply them all together, and add 1, to get our new number. What's the remainder, compared to all the primes in our set? 1: so none of them divide it! None of its prime factors are in our original set. Therefore, no matter how many prime numbers we have, we can always find more prime numbers." $\endgroup$
    – wizzwizz4
    Commented Nov 8 at 18:52
  • 1
    $\begingroup$ @PasserBy Euclid didn't think it was more complicated than what you call the "standard one". In fact, his direct proof as described in Elements (book 9, proposition 20) describes a concrete instance of a general algorithm, which is how I remember most mathematics being taught to me at that age. It might take a couple of days to understand the proof (trying lots of examples, with blocks), but I see no reason to believe it's beyond a child capable of understanding multiplication and division with remainder. $\endgroup$
    – wizzwizz4
    Commented Nov 9 at 16:29
3
$\begingroup$

Take whatever questions the child has found interesting to study, and expand upon them. Some directions will be more fun than others: pay attention to that.

For the questions you've mentioned:

  • What happens to divisibility tests in other numerical bases? (That's where we use a different number of digits: we count up to a different number before deciding we've reached one-zero.)
    • Can you find one? (That digit sum insight might help!)
    • Why does it work? Can you prove to yourself that you won't ever find any counterexamples?
      • Can you convince somebody else? (Even somebody who's being totally unreasonable about what they'll count as proof?)
    • (If it doesn't work:) Why did it look like it worked? What's actually going on here?
    • Can we find base-10 divisibility tests for the hard numbers, like 7?
    • Why the similarity between base-9's 4-test and base-10's 3-test?
  • What happens if we only use the last digit of calculations (like addition, multiplication, subtraction)?
    • This is the same as using remainder-10: what if we use remainder other things? (5, 7 and 8 are good numbers to look at.)
    • If division is the opposite of multiplication, can we do remainder-5 division?
    • What about remainder-10 division? Which numbers don't work?
    • For which remainder is 0 the only number you can't divide by?

Using techniques you'll already have studied, like “fill in the blank equations” (algebra), can really help with these problems! (No need to use letters, if squares and circles and different colours do the trick – but equally, no need to use those if letters work fine.)

If you want to make sure you're not making future school-maths boring, try university-level mathematics. (No, I'm not joking: the only reason half that stuff is “university-level” is because it's currently not in the curriculum for younger people, often because it's hard to write exam questions for.) Those questions you asked about operations lead nicely on to the study of binars of various kinds. Yes, this is incredibly dull – but the maths isn't! Clock addition, flipping shapes, and rules for transforming strings can all be used as examples to make Cayley tables from, for asking questions like:

  • What if we know that $a+b=b+a$, but nothing else?
    • Apparently rock-paper-scissors looks like that – can you make rock-paper-scissors bigger?
      • What makes rock-paper-scissors, or its variants, fair? (Yes, this is no longer abstract algebra: sue me, it's interesting.)
    • What if we also have a value that behaves like $0$: $0+a=a+0=a$?
  • What's the fewest different values where we can have a $0$, but we can define $+$ such that $a+b$ doesn't always equal $b+a$?

Alternatively, you could go for number theory along those lines:

  • We have $+$ and $\times$ – can we go bigger? Do those operations have any surprising properties?

Don't invest too much in any one idea, though: it's no use putting together a six month curriculum if the child isn't actually interested in this area of mathematics right now. (You wouldn't want to just re-create school at home.)

Improve this answer
$\endgroup$
2
  • $\begingroup$ "0+a=a+0=0". Do you mean "0+a=a+0=a"? $\endgroup$
    – panofsteel
    Commented Oct 30, 2023 at 13:59
  • $\begingroup$ @panofsteel I do. (And I made that same mistake repeatedly, all of last week.) $\endgroup$
    – wizzwizz4
    Commented Oct 30, 2023 at 14:00
2
$\begingroup$

These are great questions that find patterns within concept. They are also all closed questions with a set answer. To challenge your young mathematician further, make concepts applicable in daily life, with open-ended questions to exercise fluid thinking and reasoning. The grocery store is an easy one. There are always incongruous unit rate prices for the same items. "Hmmm...I wonder which sandwich bread I should buy. What do you think?" By not identifying any values to the various options, your child gets to identify relationships and reason their decision based on cheapest, or best value, or best unit price, etc. They are playing with the math. My credentials: I taught math for ten years, wrote math curriculum for seven years, wrote a math book, and currently train educators on how to teach reasoning from a pedagogical perspective.

Improve this answer
$\endgroup$
2
  • $\begingroup$ This looks like an introduction to "If you want to know more, you need to buy my book.". $\endgroup$
    – Dominique
    Commented Oct 30, 2023 at 8:57
  • $\begingroup$ That was far from my intention. I only wanted to point out the pedagogy to reasoning, which is an important aspect for a mathematician. I have done a lot of research on how to teach it and was sharing. I hope the person who posted can see my intention and finds fun ways to apply playing with the math in everyday situations that can have multidimensional reasoning/ answers. $\endgroup$
    – Diane
    Commented Oct 30, 2023 at 11:20
2
$\begingroup$

One of the classic responses to this question that I like is to ask the student to invent a new math concept (perhaps an operation, a new way of doing their curriculum problems, or a way of writing a number, etc.). See what they can explain about their new idea.

Also, counting problems make interesting activities. For example, "Can you count how many ways you can rearrange these four blocks?"

Improve this answer
$\endgroup$
2
$\begingroup$

Not a professional teacher but around math competitions for a long time. I love exploring combinatorics with children since the concepts are easy to imagine/visualize but problems are very deep (e.g. pigeonhole principle). Here's a sample problem from a 4-5 grade competition: "15 guests at a party sat down around a round table where each chair had a guest name tag. They noticed that no one sits on their assigned chair, so each tried shifting to the chair on their right. Show that if they continue shifting, at some point at least two guests will be sitting on their assigned chairs."

Improve this answer
$\endgroup$
2
$\begingroup$

There are some really hands on "basic" topology things you could do. The most basic is the Mobius strip: watch this Numberphile video https://www.youtube.com/watch?v=wKV0GYvR2X8&ab_channel=Numberphile for some fun hands-on demonstrations you can do.

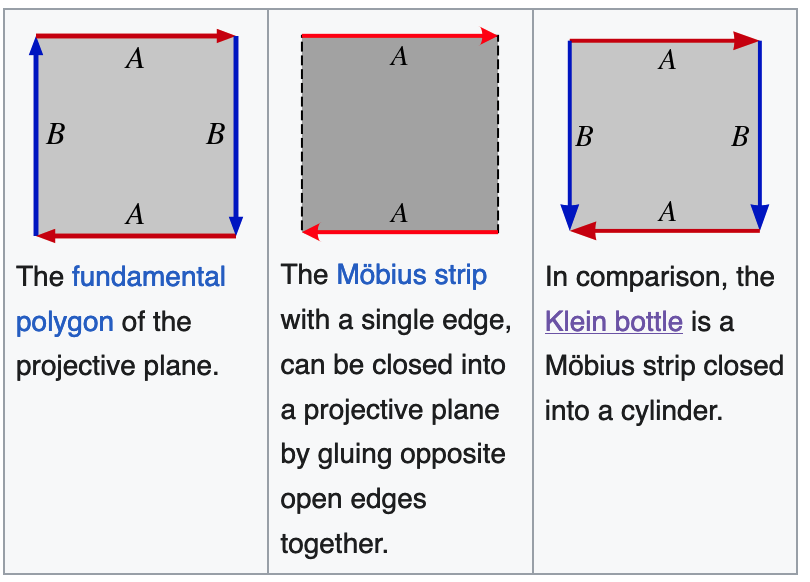
In general, one can draw some arrows on a polygon, and try to connect it up into a surface. For the square, we have the following 3: Mobius strips are the easiest to make, and then Klein bottles, and then the real projective plane. fundamental polygons

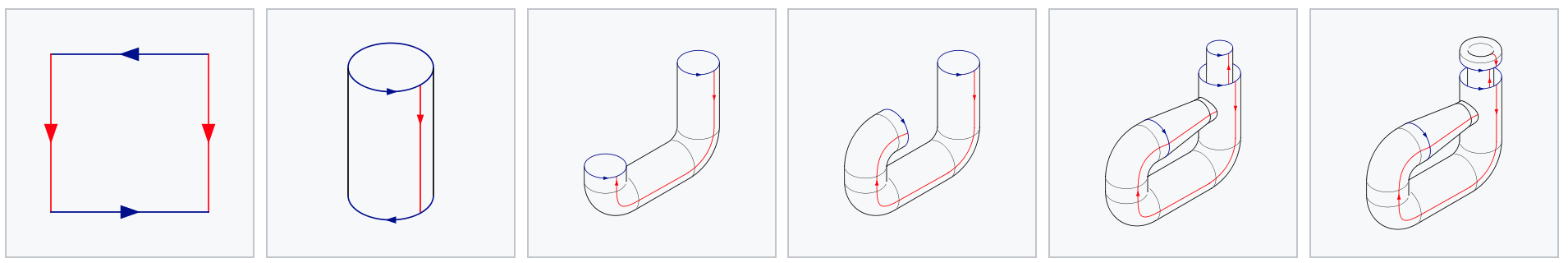
The Klein bottle can be understood via this diagram https://en.wikipedia.org/wiki/Klein_bottle Klein bottle step by step

The real projective plane is the hardest of the three, but one can make one hands-on via a paper foldable: https://divisbyzero.com/2020/04/08/make-a-real-projective-plane-boys-surface-out-of-paper/. I made one today! Smoothing this construction out, one gets a Boy's surface. They look very nice http://wordpress.discretization.de/ddg2019/2019/05/06/tutorial-4-boys-surface/.

For polygons more complicated than a square, Jos Leys https://www.youtube.com/@josleys/videos has a lot of videos connecting up various polygons. Mesmerizing to watch! Here are a couple:

Another medium of hands-on activity: you can crochet hyperbolic surfaces https://www.theiff.org/oexhibits/oe1e.html! See this TedTalk https://www.youtube.com/watch?v=D-AHvZqbMT4&t=0s&ab_channel=TEDxTalks ( Crocheting Hyperbolic Planes: Daina Taimiņa) and this tutorial video https://www.youtube.com/watch?v=xtlDND7NVp8&ab_channel=CodeParade.

If 2 dimensions gets too boring, one can go to the third https://www.youtube.com/watch?v=yqUv2JO2BCs&ab_channel=ZenoRogue. There are now lots of games that have the player play in non-Euclidean (hyperbolic) 3-space.

Hyperbolic geometry in 3-space has no shortage of difficulty: https://www.youtube.com/watch?v=IrlaVaATiOY&ab_channel=LastGinger ([HD Upscale] Not Knot - A guide to mathematical knots in hyperbolic space).

There's also this super classic and super awesome video: https://www.youtube.com/watch?v=OI-To1eUtuU&ab_channel=LastGinger ([HD Upscale] Outside In - How to turn a sphere inside out). Mathologer also has a video on sphere eversion that I find a bit easier to visualize https://www.youtube.com/watch?v=ixduANVe0gg&ab_channel=Mathologer.

Also one can play around with visualizing the 4th dimension https://www.youtube.com/watch?v=0t4aKJuKP0Q&ab_channel=%5Bmtbdesignworks%7BMiegakure%2C4DToys%7D%5D.

EDIT: there's also Henry Segerman's Youtube channel https://www.youtube.com/@henryseg. There are several videos

Another video I found very fun was this one https://www.youtube.com/watch?v=Cyhqc8l03GE (Using topology to close a rubber band bracelet), which spawned from a discussion on Math Overflow (a Q&A site for professional mathematicians; this video's construction of a rubber band bracelet is cutting edge research!)

EDIT 2/17/24: I came across a beautiful beautiful set of videos https://www.youtube.com/watch?v=6-Z0qgYjVjU&list=PLJHszsWbB6hq40r_aSVlCXDvTT0VcrgcT (also found here https://archive.org/details/M435Ep8Of8FlowsTopology), where people show lots and lots of models of surfaces, and prove some wonderful things. I feel like working with children to make these models, and playing around with deforming them (making many models to illustrate a deformation), would be really cool, and also introduce them to really deep ideas!

One idea that appears over and over again is the Euler characteristic, which is also a great theorem to study in a "hands on" manner with children, making different polyhedron and so forth.

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ (+1) I learned some years ago that there is a mathematical knitting community. Knitting Klein bottles is apparently quite popular. I learned crocheting in the third grade and would think it is likely too difficult for children younger than nine. I assume knitting is a skill that is even more advanced. $\endgroup$
    – njuffa
    Commented Oct 30, 2023 at 8:48
1
$\begingroup$

He can play with blocks. Kapla/Keva/Citi blocks are great, as are pattern blocks.

You can read him mathy stories. I love The Cat in Numberland (may be hard to find); Quack and Count is most likely too young for him, but he might like to write his own book like it (especially if he has any younger siblings); Two of Everything by Lily Toy Hong; How Hungry Are You? by Donna Jo Napoli; One Grain of Rice by Demi; You Can Count on Monsters by Richard Evan Schwartz.

You can play mathy games together. Nim is a good one, with tons of variations. I play it with stones, using 3 uneven piles. You can take as many stones from one pile as you want. The one who picks up the last stone wins (or loses, for one variation). Check out Blockus, Katamino, Rush Hour, Chocolate Fix, and Set.

Have fun!

Improve this answer
$\endgroup$
1
$\begingroup$

one possible thing to look into is knot theory. most of the modern bits are horribly complex, but you can do a lot of the basics by playing around with some string and permanent markers

Improve this answer
$\endgroup$
1
$\begingroup$

There are a lot of accessible ideas from number theory in 'the housekeeper and the professor'. It's also a lovely story in its own right and beautifully written.

I read it to my daughter when she was about 10 and we had some good conversations about maths. It complemented well what she was doing at school at the time.

She is not hugely interested in maths but it still held her interest both as a story and for the maths ideas.

I'll update this answer with some specifics if I can dig out my copy.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Based on the summary of the book provided by Wikipedia completing this answer with specific examples from the book seems worthwhile. $\endgroup$
    – njuffa
    Commented Oct 30, 2023 at 11:48
  • $\begingroup$ thanks will do. $\endgroup$
    – George Savva
    Commented Oct 30, 2023 at 12:31
1
$\begingroup$

There are a number of games based around Venn diagrams. I once saw a guessing game made up of three loops of yarn and several colored blocks. The blocks could be any of four colors, any of three shapes, and either of two sizes. The loops are laid out so as to form a venn diagram of three sets.
The teacher has a secret rule that governs each of the loops. One loop might contain all the green blocks, while a second loop contains all the square blocks, and the third loop contains all the small blocks. Each kid, in turn, gets to pick out a block and guess where it goes. If the kid guesses right, the block stays, and it scores a point. Sooner or later, the kids figure out the rule.
It might be good to play a practice round with just one loop, and then another practice round with just two loops.
Every time I've seen this played, there was at least one kid who was faster than the teacher at figuring out the three loop case.
I've included a diagram from an unrelated web page just to show how three overlapping loops form a venn diagram with eight zones in it, if you include the "outside zode". With last zone is where blocks go if they don't belong in any of the loops.

https://3.bp.blogspot.com/_r94Ze5inyTo/TKSGchMom9I/AAAAAAAAAfo/hXQHKb9AAjo/s1600/kids+venn.jpg

Here's another image from a product that facilitates Venn games. I've never used the product, so I have no opinion of it's value.

https://i.pinimg.com/originals/36/18/42/36184276d7f286cb44858ac994ac4609.jpg

Improve this answer
$\endgroup$
1
$\begingroup$

A classic one I heard from Janos Komlos to keep his kids busy was "can you find a square number whose remainder when divided by 3 is 2?"

Once you have exposure to modular arithmetic this is trivial but without it the result can seem very mysterious. Also right around the corner from this result is the starting point for things like Algebraic Number Theory and yet this is a very very elementary question to ask.

Another related one is can you find a fraction that squares to two? The proof of this is understandable by a grade schooler but requires a lot of creativity to come up with, and hints towards a lot of interesting math. Also the history around it (people died for this proof!) would be very engaging to also discuss.

For high schoolers. After having shown the Geometric Series, discussing $1-1+1 ... = \frac{1}{2}$ and the notion of Cessaro Summation can be very interesting. It challenges our intuitions of "what it means to sum" if there are procedures that sum all convergent sums correctly and yet also can assign values to divergent sums. To me that felt insanely profound the first time I was exposed to it.

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ Do you mean "whose remainder is $2$"? Otherwise, after coming up with $1^2$ or $2^2$ or $4^2$, they'll say "Next question please." With remainder $2$ they'll try examples for a while and then begin to wonder why it never works. This, however, seems a little bit advanced, even for bright six year olds. $\endgroup$
    – Will Orrick
    Commented Oct 30, 2023 at 15:30
  • $\begingroup$ ah lol good catch! I should have thought about what I was writing as I wrote it. $\endgroup$
    – Sidharth Ghoshal
    Commented Oct 30, 2023 at 15:34
  • 1
    $\begingroup$ I also think theres value in mysteries. Like imagine being exposed to a mystery like this in 1st grade and then in 7th grade getting an answer. It's kind of nice for Math to seem like a mysterious subject with patterns that are easy to see but hard to explain $\endgroup$
    – Sidharth Ghoshal
    Commented Oct 30, 2023 at 15:36
0
$\begingroup$

This kind of approach may be helpful to improve exploring skills in mathematics and if you can start by giving hints for elementary students later on they can buildup lateral thinking which is the key point in creating new conjectures and solving global challenges. Here I share my suggestions,

  1. How to illustrate geometrically that, sum of two consecutive triangle numbers is a square number and what are the other cases relevant to triangle numbers which you can be proved geometrically?
  2. Many of the students know how to obtain the product when single digit number is multiplied by 9 using the pattern. How can you describe the pattern when two digit or three digit numbers are multiplied by 9 ?
  3. In 9 th century Al-Khuwarizmi discovered completing square method by geometrical approach. Since negative numbers were not used at that time he described his approach for six different types of quadratic equations. How can you apply his geometrical approach for negative coefficients without using negative values as side lengths?
Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.