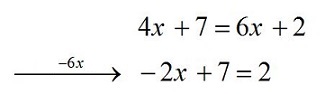18
$\begingroup$

This is a common way to write the steps during solving equations: enter image description here

But in GeoGebra the steps are shown this way (the highlighted part):

enter image description here

I'm going to use GeoGebra to teach equations. Is it OK to let the students write the steps just like GeoGebra (I mean, with paranthesis)?

Improve this question
$\endgroup$
1
  • 6
    $\begingroup$ I have never seen the notation you say is "a common way", where is it used? $\endgroup$
    – JiK
    Commented Feb 27, 2018 at 13:08

10 Answers 10

Reset to default
13
$\begingroup$

I wouldn't do that. The parenthesis in use are also used for legal expressions within equations. So you can end with one line containing the same parenthesis meaning different things, what looks like a seed of confusion to me.

If you prefer, you can replace the GeoGebra parenthesis with some others i.e. <>, or [] etc. to keep the notation similar to Geogebra but to remove (reduce) the potential for the confusion.

Or what about writing steps like this:

4x + 7 = 6x + 2

 -6x = -6x

-2x + 7 = 2

This way you also get a bit of sensibility for systems of equations later.

Improve this answer
$\endgroup$
3
  • 3
    $\begingroup$ I think you intended for there to be no equal sign between the two appearances of $-6x$, correct? With the equal sign, it's pretty confusing. Without it, it would look just like how I write such operations all the time. $\endgroup$
    – Todd Wilcox
    Commented Feb 26, 2018 at 19:56
  • 6
    $\begingroup$ @ToddWilcox I don't know... I put equals sign sometimes. It shows that we can subtract that from both sides, since they are equal. (In this case, it's reflexive and pretty obvious, but it might be helpful in other cases) $\endgroup$
    – Quintec
    Commented Feb 27, 2018 at 0:55
  • 3
    $\begingroup$ I think the point about this being relevant for multivariable linear systems is an important one. With that in mind, the equals sign lays the groundwork for a fairly universal principle, that you can add and subtract equations (in the sense of adding/subtracting the things on the left side and also the things on the right side, and you wind up with another valid equation). $\endgroup$
    – David Z
    Commented Feb 27, 2018 at 6:33
10
$\begingroup$

The problem with

$(4x+7=6x+2)-6x$

is that there is no subtraction operation that involves subtracting a term from an equation. Subtraction involves subtracting a term from a term. So the correct notation is

$(4x+7)-6x=(6x+2)-6x$

Improve this answer
$\endgroup$
6
  • 4
    $\begingroup$ It's just a notation, not an operation. It an abbreviation for "subtract $6x$ from both sides". $\endgroup$
    – Behzad
    Commented Feb 26, 2018 at 8:55
  • 9
    $\begingroup$ @Behzad The point is that it is confusing, misleading, and inconsistent notation. $\endgroup$
    – Todd Wilcox
    Commented Feb 26, 2018 at 19:55
  • $\begingroup$ @ToddWilcox Don't you suggest an abbreviation for "Subtract 6x from both sides"? If you do, what's it? $\endgroup$
    – Behzad
    Commented Feb 26, 2018 at 22:40
  • 3
    $\begingroup$ @Behzad I don't think it's valuable to try to abbreviate mathematical notation, in general. Mathematicians are prone to brevity already, and the way things are written mathematically usually are already close to the shortest possible way without sacrificing clarity. $\endgroup$
    – Todd Wilcox
    Commented Feb 26, 2018 at 23:29
  • $\begingroup$ Technically (from a programming point of view) you could (canonically) "overload" the binary operator "-" to take an equation on the left and a term on the right and return an equation as result where the LHS and RHS are obtained by subtracting the term from the LHS and RHS of the given equation... When I saw that notation for the first time this is what I presumed to be happening, although I admit it can't be claimed to be an obvious interpretation... $\endgroup$
    – Pedro A
    Commented Feb 27, 2018 at 1:57
10
$\begingroup$

Speaking as someone who has taught college precalculus several times, I have an intense dislike for the way that Geogebra writes this step.

In my opinion, it is very important to emphasize to students that we are subtracting 6x from both sides of the equation, which means of course that we are subtracting 6x from two different expressions.

If we write "-6x" only once, it may cause students to have the unfortunately common misconception that subtracting 6x is something you can "just do". (You can, of course, do it to both sides of a statement that says that two expressions are equal.)

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ Don't you suggest an abbreviation for "Subtract $6x$ from both sides"? If you do, what's it? $\endgroup$
    – Behzad
    Commented Feb 26, 2018 at 22:33
7
$\begingroup$

I'm going to use GeoGebra to teach equations. Is it OK to let the students write the steps just like GeoGebra (I mean, with parenthesis)?

I would not allow this in my class, but it would depend what you think is good/clear/consistent notation for your students.

The developers at GeoGebra made a choice for showing algebra steps, but that doesn't mean we have to accept it as a norm. If the developers decided to allow something like $$5x+3=15x$$ $$sub/div (5x+3=15x)$$ $$x=\frac{3}{10}$$ to indicate a "subtract first, then divide" procedure, would you allow students to write this? I doubt it.

The problem is that I am fighting all the time to get my students to write in a clear and consistent manner. They'll write something like $$4x+3=2x+11$$ $$3-4x+3 = 2x+11-3$$ $$4x=2x+8$$ explaining that they've "subtracted 3 from both sides". They feel that because they knew what they were doing, that I should be okay with how they wrote. Of course, I am not okay with it, and part of my job is to correct their use of notation.

In short, I would not let GeoGebra's shorthand become part of your students' practice. If you do, their future teachers will be justifiably confused, and your students will have to spend valuable time relearning how to show algebraic steps.

Improve this answer
$\endgroup$
5
$\begingroup$

Thinkeye's answer is good in that it easily extends to dividing two related equations and similar, more advanced operations. On the other hand, for the sake of brevity, I would suggest the way I have been taught to explicitly specify operations:

$$ 6x + 14y = 4x + 12y \quad | -4x $$

A vertical line to separate the equation from the intended operation. Such vertical lines are frequently used to indicate "a divides b" as in $a|b$, but I think that there is little risk of confusion here. Same is true for absolute value delimiters.

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ I use two such lines "||", since that is what I was taught in school or gymnasium. I justify it by saying that two lines are like the two sides of an equation. $\endgroup$
    – Tommi
    Commented Feb 27, 2018 at 9:06
5
$\begingroup$

Since I recently had a math teacher from another institution observe me during an Algebra 2 lesson and comment positively on my notation ("I'm going to steal that!") here is a worked example:

Find all values of $x$ that satisfy $3(x-4)^2 + 8 = 23$.

 

$-8: \hspace{20 mm}3(x-4)^2 = 15$

$\div3: \hspace{20 mm} (x-4)^2 = 5$

$\sqrt {}: \hspace{20 mm} x-4 = \pm \sqrt{5}$

$+4: \hspace{20 mm} x = 4 \pm \sqrt{5}$

Sometimes I will use other symbols on the left hand side such as $\square$ for "square both sides" or individual letters such as $c$ for "collect like terms" or $f$ for "factor [at least one side of the equation]."

The general approach can be modified to one's liking, but the key component is indicating clearly how to get from each line of one's work to the next; I had found that students were carrying out some operations in ways that made it tough for me to decipher what they had done because various steps were mixed together along with cancellations or crossed out coefficients/variables. It was also more difficult for me to point with specificity to where an approach had gone awry.

I find that the above-notation suitably merges mathematical rigor with pedagogical clarity (especially around following student work) with its main drawback being speed, which I am happy to sacrifice (to some extent) as students cement basic arithmetic/algebraic fluency.

Improve this answer
$\endgroup$
3
$\begingroup$

If I had to communicate the solution to this problem to someone else in writing, I'd probably write

...gives $$4x+7=6x+2\text{.}$$ Subtracting $6x$ from both sides of this equation gives $$-2x+7 = 2\text{.}$$

If the equality were one in a long chain, I'd write

$$\begin{split}&\ldots \\ 4x+7&=6x+2 \\ -2x+7&\stackrel{\text{(a)}}{=}2 \\ &\ldots \end{split}$$ We arrive at (a) by subtracting $6x$ from both sides...

If I were just typing up notes for myself, I'd write

$$\begin{split}&\ldots \\ 4x+7&=6x+2 && \\ -2x+7&=2 & &\text{subtract }6x\text{ from both sides} \\ &\ldots \end{split}$$

and in my calculation notebook I'd probably write

$$\begin{split}&\ldots \\ 4x&+7&=\,\,\, 6x+&2\\ \underline{-6x}& &\quad\underline{-6x} \\ -2x&+7&=&2 \\ &\ldots \end{split}$$

It seems that, in practice, I use words, not notation, to convey the idea of applying the same function to both sides of an equality to get a new equality. Flipping through a few textbooks suggests that most other mathematics users are largely the same in this respect. The preference for rhetorical instead of symbolic description and the lack of consensus on a notation suggest that having a notation for this idea is not that useful for most people.

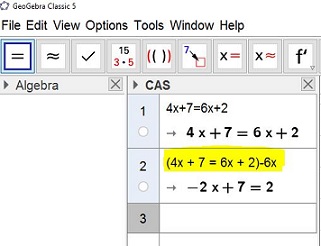
On the other hand, people who work with proof assistants do need such an idiom, because doing the same thing to both sides of an equality to get a new equality is an important proof technique that needs to be formalized. For instance, Idris and Agda have cong:

cong : {f : t -> u} -> (a = b) -> f a = f b
cong Refl = Refl

cong : ∀ {a b} {A : Set a} {B : Set b}
   (f : A → B) {x y} → x ≡ y → f x ≡ f y
cong f refl = refl

I expect that this necessity is the motivation that GeoGebra's designers had in mind.

Improve this answer
$\endgroup$
2
$\begingroup$

I encoded my preferred notation in this web-app for practicing equation solving:

http://thewessens.net/ClassroomApps/Main/equations.html?topic=algebra&path=Main&id=2

enter image description here

It has served me quite well. The picture shows a completed solution - at each step the student enters an operation on each side (unless it is expansion which is one side only) and the result is shown.

Note the use of colour, the clarity in choice of an "opposite" operator, and the emphasis on "doing the same thing to both sides".

Improve this answer
$\endgroup$
2
  • $\begingroup$ How well does this notation work for paper-and-pencil calculations, which only have a single color? $\endgroup$
    – Jasper
    Commented Mar 4, 2018 at 20:42
  • $\begingroup$ I use colour for clarity in the app and on a whiteboard, but do not require it for pen and paper since location and size does much the same job. Often a student is happy to switch pen colours to make it look nicer though. $\endgroup$
    – kallikak
    Commented Mar 5, 2018 at 1:09
1
$\begingroup$

I think it‘s important to represent steps as happening between equations, linking one to the next. I do this similarly to kallikak‘s notation, only with arrows on either side. This shows that steps can be reversed, and also that some operations split one equation into two, such as taking a root or the zero-product rule. Arrow notation also mirrors functional notation and thinking.

Improve this answer
$\endgroup$
-1
$\begingroup$

Geogebera is bad here. Stop trying to make something bad work. Do it the old fashioned way.

Kids have a hard tie with this stuff. Show every step and emphasize that it is an equality and you perform same operation to each side (NOT move stuff from one side to other). Once kids have been drilled hard they don't need to show every step. But when they are new, drill it in detail.

Something like this:

ax + b = cx - d

ax + b - cx = cx - d - cx

ax -cx + b = -d

(a-c)x = -d - b

(a-c)x = -(d + b)

x = -(d+b)/(a-c)

x = (d+b)/(c-a)

x = [evaluate fraction numerically]

And don't just have them solve it generally. Have them solve numerical examples over and over.

Drill, drill, drill. If you think that is too tedious for our young computer generation, don't complain to me (or have their higher course math and science teaches complain) when they mess up algebra, get signs reversed or ratios upside down.

[Pedant protection, making a point here. If I messed up my algebra, it's kharma.]

Improve this answer
$\endgroup$
0

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.