32
$\begingroup$

(I was inspired by the comments in this answer to ask this question.)

I have some multiplication table cards from Kumon that have a list of commonly mistaken multiplications: $7\times 8, 4\times 8, 11\times 12, 7\times 9, 6\times 7, 12\times 8, 4\times 7, 6\times 8, 9\times 12, 8\times 9, 11\times 11$, and $6\times 9$ (in this order).

Kumon multiplication table cards

I assume that this is based on data they obtained from the numerous children who have answered their worksheets.

Is there some other source (a study, perhaps) that lists the products of single digits that children usually get wrong?

Improve this question
$\endgroup$
13
  • 6
    $\begingroup$ My adult students would agree with that. 7*8. $\endgroup$
    – Sue VanHattum ♦
    Commented Mar 3, 2021 at 15:34
  • 3
    $\begingroup$ For $7 \times 8$, try doubling $7 \times 4$ as a way of remembering it. Or thanks to @Adam try $8 \times 8 - 8$. $\endgroup$
    – J W
    Commented Mar 3, 2021 at 16:14
  • 2
    $\begingroup$ @ruferd: 7x8 is easy to recast as 8x8 - 8, and power-of-2 stuff is hard-wired into the brains of many computer geeks so that makes it very easy for me. (Especially since multiples of 8 come up often when dealing with bit shift counts for whole numbers of bytes, and other low level SIMD / bithack stuff that I spend a lot of time on. So I fully realize that I'm not a typical person learning their times tables :P I tend to have to think harder about multiples of 7 than most others, probably because it's the largest single-digit prime so resists many simple tricks.) $\endgroup$
    – Peter Cordes
    Commented Mar 4, 2021 at 10:36
  • 27
    $\begingroup$ @ruferd Just remember it like this: $56 = 7 \times 8$ (i.e. 5,6,7,8) $\endgroup$
    – Jann Poppinga
    Commented Mar 4, 2021 at 13:14
  • 5
    $\begingroup$ And if comparing $7 \times 8$ and $9 \times 6$, just remember that (for a fixed sum) the product will become larger if the numbers are closer together. That's often more useful to remember than a specific product, anyway. $\endgroup$
    – Misha Lavrov
    Commented Mar 4, 2021 at 20:15

2 Answers 2

Reset to default
48
$\begingroup$

https://www.theguardian.com/news/datablog/2013/may/31/times-tables-hardest-easiest-children

There are links to a dataset in the article. As far as I can tell, this isn't a formal study:

But some new data generated by pupils at Caddington Village School in Bedford sheds light on which multiplications are actually the hardest – and how kids do overall.

The data is generated by an app produced by an app developed by education tech firm Flurrish, and in total the 232 children who participated produced more than 60,000 answers. Here's how they did

So the data is of unknown quality, but the graph is both pretty and pretty believable. (Except that I'd probably label the graph below inaccuracy rather than accuracy.)

It's notable that the data is slightly asymmetric but I'm unsure if that is statistically significant. i.e. Do kids use commutativity?

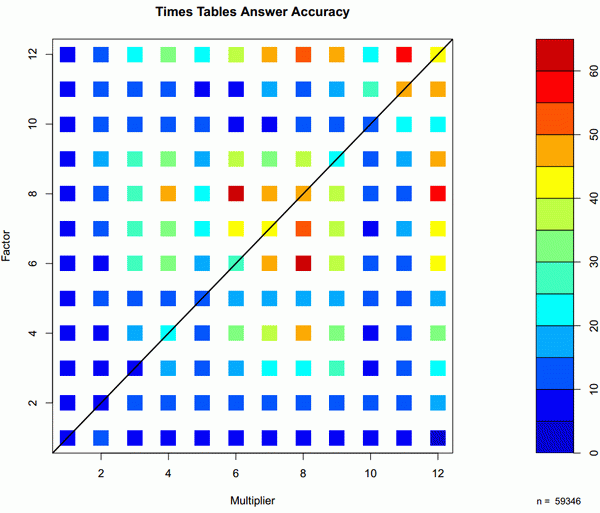
enter image description here

Improve this answer
$\endgroup$
28
  • 10
    $\begingroup$ Also the row/column of 10 that drops accuracy for 10*11 and 10*12. They've memorized a rule, but seem slightly uncertain if the rule applies to numbers higher than 10. $\endgroup$
    – ruferd
    Commented Mar 3, 2021 at 18:26
  • 13
    $\begingroup$ Is it common to teach multiplication tables up to 12 in english-speaking countries? Or is this maybe specific to the UK (due to the historical 12 pence in a shilling) and the US (due to the still current 12 inches to a foot)? In the French system we only learn them up to 10. Or at least we used to, though I doubt this has changed. $\endgroup$
    – jcaron
    Commented Mar 4, 2021 at 11:24
  • 4
    $\begingroup$ What surprises me about this chart is the asymmetry. 7 × 6 is wrong considerably more often than 6 × 7. Likewise for 12 × 11 vs. 11 × 12, 12 × 7 vs 7 × 12, etc. Not sure if this is just noise in the data or kids confused about commutativity? $\endgroup$
    – Darrel Hoffman
    Commented Mar 4, 2021 at 14:00
  • 13
    $\begingroup$ @DarrelHoffman: Looks mostly like noise to me. I played with the raw data a bit, and none of the results seem to be more than 2 standard deviations away from what would be expected under the null hypothesis of commutativity. Furthermore, the biggest deviations are for "easy" pairs like 6×3 vs. 3×6 (74% vs. 82% correct, 1.96 σ), 12×1 vs. 1×12 (90% vs. 95% correct, 1.66 σ) and 10×3 vs. 3×10 (88% vs. 92% correct, 1.56 σ). For 7×6 vs. 6×7 the deviation is less than 0.6 σ, easily explained by chance. $\endgroup$
    – Ilmari Karonen
    Commented Mar 4, 2021 at 17:55
  • 3
    $\begingroup$ The asymmetry is significant and expected. If you had presented a symmetric plot I would have downvoted because I know it's asymmetric. $\endgroup$
    – Joshua
    Commented Mar 4, 2021 at 19:11
6
$\begingroup$

In the comments, it seems some people are surprised that $4 \times 8$ and $6 \times 8$ have such low accuracy (as shown in the table in the accepted answer).

There's actually a cognitive principle behind this: associative interference, the phenomenon that conceptually related pieces of knowledge can interfere with each other's recall.

For instance, when recalling $4 \times 8,$ related facts like $\mathbf{4} \times 6 = \mathbf{24}$ and $3 \times \mathbf{8} = \mathbf{24}$ interfere with the spreading activation during the recall process and increase the likelihood of the error $4 \times 8 = 24.$

(Spreading activation is a method by which connections between information can be used to recall information in response to a stimulus. The stimulus activates some piece(s) of information, and the activity flows through connections to other pieces of information.)

Here's a diagram that I made to illustrate:

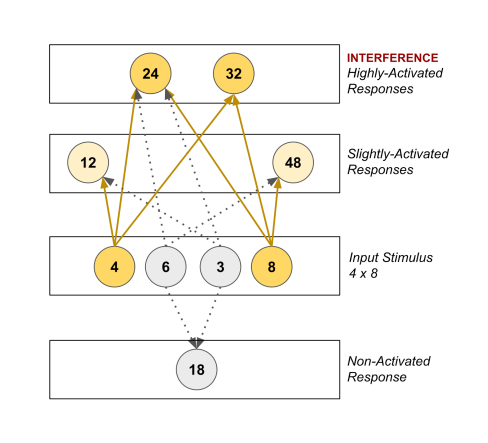
spreading activation when recalling 4x8

Multiple studies have shown that well over half, and potentially as high as 90%, of multiplication mistakes are caused by interference. See the following reference for a summary:

Campbell, J. I. (1987). The role of associative interference in learning and retrieving arithmetic facts. Cognitive processes in mathematics, 107-122.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.