Some teachers teach multiplication between integers with the following rules:
plus with plus gives plus
plus with minus gives minus
etc.
So for example in order to deal with the multiplication $2\cdot(-3)=-6$, they uses the second rule above.
I've never liked this approach, for me it's like a memorization which the students don't know the really meaning of this multiplication.
So my question is how to teach students about multiplication between integers for the first time?
My preferred model of multiplication with integers involves motion.
Imagine you are recording a video of a car driving at a certain velocity. If the car is going forward, the velocity is positive; if the car is going backward, the velocity is backward.
Now when you play the video, you can choose whether to play it at normal speed, or at 2x, 3x or 4x normal; you can also choose whether to play the video backward at any of those speeds, and backward playback speeds are treated as negative numbers (-2x, -3x, -4x...)
When you watch the video, the car appears to be driving at a speed that is the product of its actual velocity and the playback speed. That is, if the car is driving at 10 miles per hour and you play the recording at 3x speed, it appears to be driving at 30 miles per hour. If the car is driving at 10 miles per hour and you play the recording at a -2x speed, the car will appear to be driving at -20 miles per hour (i.e. it will seem to be going backward).
Now it should be clear that a negative times a negative is a positive: a car driving backward, watched in reverse, will appear to be going forward. All of the other multiplication rules for integers also make sense in this context.
I always liked fill in the following pattern:
$$(3)(4)=12$$
$$(3)(3)=9$$
$$(3)(2)=6$$
$$(3)(1)=3$$
$$(3)(0)=0$$
$$(3)(-1)=$$
$$(3)(-2)=$$
The students should see that when the second multiplication number decreases by 1, then the product decreases by 3. Then when they hear "negative times a positive = negative" they can actually see it isn't a random rule, but a logical rule that was decided in order to make this pattern consistent. Once they know a negative times a positive equals a negative, then you can use
$$(-3)(3)=-9$$
$$(-3)(2)=-6$$
$$(-3)(1)=-3$$
$$(-3)(0)=0$$
$$(-3)(-1)=$$
$$(-3)(-2)=$$
And then they see here that when the second number is decreased by 1, now the answer increases by 3. So the "negative times a negative = positive" rule isn't a random rule for no reason, again it was decided in order to make this pattern consistent.
Perhaps using this approach, you can get the students to create the rules themselves without you telling them, and that way they will more likely be able to recall those rules since they "invented" the rule themselves instead of it being something they were told to memorize.
There are two useful practical situations where the interaction of signs appears. I recall using the temperature analogy when in school.
Zero degrees (Celsius) is the temperature where water freezes. Around where I used to live, winter temperatures were often much colder than that. This approach is physically suspect, though.
Both money and temperature and familiar concepts to school children and can be used to illustrate the double negative.
The best way to teach multiplication of integers is to use two sided counters. This is clearly explained in this YouTube Video I call this the best way because it gives a clear conceptual picture of what's going on.
Here are some clips from the video with my comments. Integers are represented by the yellow side for positive, the red side for negative and equal numbers of red and yellow for zero.
When multiplying a positive times a negative such as 4 times -2, start with zero and add 4 sets of -2.
When multiplying a negative times a negative you will want to take away sets. You can take away sets by starting with pairs of positive and negative that add up to zero. Here is an example where we are multiplying -4 times -2. We know we will have to take away 4 sets of -2. So we start with 0 made up pairs of -2 and + 2.
In this second step of -4 times -2, we circle the 4 sets of -2 that we are taking away.
We then take the four sets away.
We can count what's left or remove the extra zero pairs and then count. Either way -4 times -2 leave +8.
The video continues with additional examples including -4 times 2 in which you take 4 copies of 2 away and leave -8.
In summary. a) When multiplying by a +, start with zero and add that many sets of yellow (+), or red (-) b) When multiplying by a -, start with zero (in this case pairs of chips that are equal to zero) and take away that many set of yellow (+), or red (-)
Acting this out helps make sense of the rules in the beginning of your question and students will soon discover the rules and be able to use the rules without the chips.
Many of these rules are the way they are for the sake of consistency. When negative numbers were created (or discovered, if you like), we had to work out the rules such that multiplication would still be consistent with arithmetic, otherwise it would lead to contradictions.
While intuitive analogies have their place in teaching, and certainly modeling and applications as well, the only way I see it to get past the memorization stage in learning new topics in mathematics, is to know enough of the connected pieces such that the rule makes sense as it is, for the sake of consistency. Obviously, in the beginning, that isn't possible because the student is learning, so naturally, the students must rely on memorization until more of that connected web builds.
In any event, you might want to start showing students some of the inconsistencies that occur IF we apply the wrong rule. For example, if (-25) times 1 was (25), then 25 divided 1 would be (-25), but we know that 25 divided by 1 is 25. Thus, (-25) times 1 must be -25 and -25 divided by -25 must 1, and so on.
Unfortunately, memorization gets a bad rap, but it is a natural part of the process early on. You want to pay attention to the progression of sophistication in your students' thought and see that they progress from the rote stages to the knowing stages.
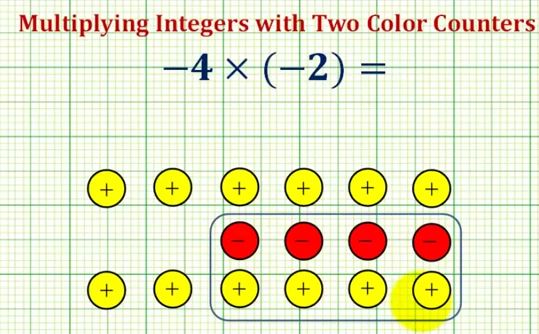
One way uses a number line.
|----|----|----|----|----|----|----|----|----|----|----|----|----|----| -6 -5 -4 -3 -2 -1 0 1 2 3 4 5 6 7 8
Taking a concrete geometrical point of view, short joinable rods made of straws could be used.
A number of rods each of length 1, 2, 3, ... can be made and the following done on a flat page.
From the origin place rods on the number line, either right or left. Given a placed rod we may apply the negative sign to it. The negative sign rotates a rod a half-turn around the origin.
Note: The negative sign "$-$" itself has half-turn rotational symmetry.
Examples:
2 x 3 = 6 : start at the origin with a rod of length 3 and join a second end-to-end.
2 x -3 = -6 : first place the rod to the left so it is length -3 and join a second.
-2 x 3 = -6 means first place the rod of length 3 to the right,
then join a second of these making length 6 to the right,
then apply the negative sign which is a half-turn.
It may be better if eventually this rotation and scaling could be done in one operation.
-2 x -3 = 6 means place the rod to the left so it is length -3,
join a second of these making length -6 to the left,
then apply the negative sign which is a half-turn, ending up 6 to the right!
This would useful later if introducing complex numbers.
By this stage they should be familiar with angles and multiplying pairs of now abstract numbers where there is no difference in type between the first and second number (which doesn't have to represent a quantity from physics like a rod of length 3cm). Studying examples like above, they may see that multiplying pairs of numbers means summing their angles and scaling their lengths.
(They could begin this using 1 and -1, remembering +1 is one to the right and -1 is one to the left.)
It is then quite a puzzle to see where a unit length must be placed from the origin, so that when multiplying it by itself (two equal rotations), it ends up as -1. The number which satisfies this no longer lies on the number line but, is somewhat between -1 and 1 in resemblance, and is called i.
