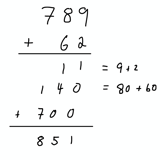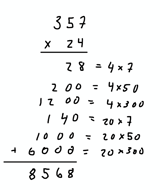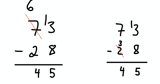Alternative approaches in basic mathematical operations

Question

Sometimes awareness of alternative approaches of different origins may be helpful for students to improve their creative skills in mathematics. What would you suggest as alternative methods in basic mathematical operations such as addition, subtraction, multiplication, division, squaring, and finding square roots?

As an example when it comes to the multiplication of numbers, there are alternative ways such as the Japanese method using straight lines and the Chinese method using lattice. There should be much more to explain to our students and it would be great if you could share your experience and knowledge related to this issue.
P.S.
I thought it would be better to add further information to give a much clearer idea about this issue.
1)There can be some special benefits when applying a particular approach, as example when you consider the products 11×11, 111×111,..... it is much easier to understand the pattern using a straight-line approach rather than the so-called standard method.
2) As I stated in my original question, what I'm interested in here is not just alternative approaches but approaches from different origins.
3)If talking about alternative approaches is not good in classroom for all students as a common topic, what about discussing these as activities in other platforms like maths clubs , considering as an option for students who are interested.

Answer 1

I would be very cautious when introducing any algorithms other than the standard methods. The standard algorithms are standard for a reason: they're easy to set up and hard to mess up. (The standard algorithm for multiplication by hand is long multiplication, and, as Xander mentions in the comments, it has been standard for the past century or so in the USA.)

The lattice method, for instance, is hard to set up (it takes a lot of time and effort to draw the entire grid with diagonals) and easy to mess up (if I had a dollar for every time a student drew a sloppy grid with misaligned diagonals and screwed up the calculation as a result, I'd be filthy rich).

Kids will often latch onto whatever method they "like" best, as though it were a flavor of ice cream, regardless of its practicality -- and their incentives are often misaligned. For instance, I've tutored students who straight-up told me they preferred the lattice method because they liked being able to take a break from math to draw the grid (and believe me, they took their sweet time drawing the grid and making it perfect). Of course, it took these students forever to complete their problems because they were working with incredibly low efficiency, and that frustrated them, but another factor leading them to resist switching to the more efficient standard method was they had completely forgotten it (as a result of using the lattice method for so long) and relearning it would require some additional up-front time and effort on top of what already felt like an overwhelming workload.

I'm not against alternatives, but as a teacher you have to run through a simulation in your mind: "what will happen to students who latch onto this method indefinitely and resist using other methods?" If the alternative method is just as efficient and just as general, then sure, introduce it. But if not, then I wouldn't introduce it, because students who latch onto it and resist letting go are going to be in for a world of hurt. (Even if you introduce an alternative method as a fun, temporary vacation away from standard techniques, some students will try to stay on that vacation forever.)

Note that while I be focused on the lattice method in this answer, the same argument applies to the "straight lines" method, which seems even harder to set up and even easier to mess up.

Answer 2

It is absolutely a good idea to give students different ways of thinking about things, but I do not think that the examples in the question are good examples of what this should look like.

What is wrong with the given examples?

The examples in the question are all examples of algorithms which students might use to compute a product, not examples of how students might think about what a product is. Generally speaking, having more algorithms available doesn't really help students to understand what they are doing any better—it just gives them more ways of doing computations (which, honestly, might cause confusion).

Teaching more algorithms also leads students into the trap of thinking that mathematics is nothing more than a bunch of computational "tricks", which must be applied in certain circumstances. Students get into the mindset of looking at a problem, trying to decide what "type" of problem it is, and then implementing whichever tool or algorithm they determine is the "correct" tool for the job.

Personally, I would avoid teaching too many algorithms—keep it to the minimal set of techniques which will be needed to get the job done.

What could one try instead?

Thinking about multiplication, there are a number of ways of describing what multiplication "is" which might give students different approaches for solving problems. For example:

• Multiplication is Area: To the ancient Greek way of thinking, number was a length and a product was an area. To multiply two numbers $a$ and $b$, lay one length horizontally and the other perpendicular in order to build a rectangle with width $a$ and height $b$. The area of this rectangle is the product.

  This isn't a great model for computation, since it can be hard to figure out what the area of a rectangle is, but multiplication over the natural numbers can be done with physical manipulatives. To multiply $a \times b$,

  1. lay down a horizontal line of $a$ stones (or coins, or poker chips, or whatever),
  2. starting at the far left, lay down a vertical line of $b$ stones, arranged so that the left-most stone of the horizontal line is the bottom-most stone of the vertical line, and then
  3. continue laying down stones until a rectangle is formed.

  The product $a\times b$ is equal to the area of the rectangle, which can be computed by counting the number of stones. I have put together a small Desmos model for this: https://www.desmos.com/calculator/n2e06dhgdq .

  I like this model because it reverses the usual approach: what often happens in elementary school is that students are first taught algorithms for multiplying numbers, and then taught to use these algorithms to find areas. Here, area is a product, from the start. A student who understands this model doesn't have to ask if they should multiply or add (or whatever) to find an area—it should fairly quickly be internalized as part of the definition.

• Multiplication is Scaling: Imagine a real number line sitting in a plane, made out of some rigid material (e.g. imagine a wooden ruler). Attach the ray $[0,\infty)$ to this rigid number line at zero, and imagine that this half-ray is made out of some elastic material which can be stretched or compressed.

  The product $a \times b$ can be thought of as scaling $b$ by a factor of $a$. To visualize this, imagine the entire ray $[0,\infty)$ being scaled by this factor—stretch the elastic ray so that the $1$ on the ray aligns with $a$ on the rigid number line; the product $a\times b$ can be found by locating the $b$ on the elastic number line and determining where it lines up.

  To give an idea of what this looks like, I've whipped up a very basic GeoGeobra model: https://www.geogebra.org/calculator/pk3d65ax . To multiply $a \times b$,

  1. Set $a=1$ (this aligns the blue ray with the real number line on the $x$-axis).
  2. Set $b$. Note that the purple point labeled $b$ will align with the value of $b$ on the real number line.
  3. Set $a$ to the actual value of $a$.
  4. The purple point on the ray will now align with the product $a \times b$.

  One of the advantages of this model is that it very quickly justifies the rules for multiplication by negative numbers (e.g. "negative times positive is negative"): if $b$ is negative, then the ray initially points along the negative $x$-axis; scaling by a negative $a$ reverses the orientation of the ray when $1$ on the ray aligns with the negative value of $a$.

• Multiplication is Repeated Addition: First off, I side very much with Keith Devlin's assertion that Multiplication ain't no repeated addition, and I think that it is important to teach multiplication as being a distinct operation. However, there are versions of multiplication-as-repeated-addition which pop up in other places^[1], and treating it as repeated multiplication does give rise to an algorithm for computation. And this model can be justified for natural numbers by looking at the area model (add together $b$ rows, each of which contains $a$ stones—this is really just $a+a+\dotsb+a$ (with $b$ copies of $a$).

  I am not a huge fan of this model, but I am not sure that it hurts to introduce it to students alongside other models.

Summary

I think that it is very important to give students different ways of thinking about the tools, definitions, and results which they being introduced to. But this different ways of thinking need to be about the underlying logical model, and not just distinct algorithmic or computational tricks.

The idea is that students should have multiple internal models, which gives them multiple lines of attack when trying to solve a problem. This kind of mental flexibility should be supported and encouraged.

---

[1] For example, in the study of groups, one might write $$ ng = \underbrace{g+g+g+\dotsb+g}_{\text{$n$ times}} $$ in order to denote adding the group element $g$ to itself $n$ times, where $n$ is a natural number. However, that is way beyond the scope of an elementary school math curriculum.

Answer 3

I think that introducing new algorithms just for the sake of diversity is not such a great idea especially if you have the intention of getting students to be proficient in multiple algorithms. Achieving proficiency in one algorithm is hard enough.

I do think that the "standard algorithms" are suboptimal for understanding and for checking your work. Here are "alternative" algorithms which I would actually make the new standard if I had the power. These are not my own inventions: they are known in the education world as "partial sum", "partial product", or "scaffold" algorithms.

Addition

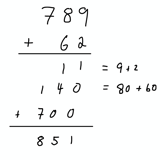
This algorithm reinforces place-value concepts. Instead of placing a random "one" above the 8 (not understanding that this one actually represents ten!), we explicitly show the partial sums.

We can verbalize our reasoning: "I need to combine 789 units with 62 units. First I combine the 9 singles with the 2 singles to get 11 singles. Then I combine the 8 tens with the 6 tens to get 14 tens (which is 140)..."

We can also check our work. The standard algorithm is hard to "debug": you can get single digit sum wrong, forget to carry, carry to the wrong place value, etc. Looking back at your work it is very hard to tell where an error happened.

With this algorithm, if the student made an error like 80 + 60 = 120 they can see exactly where the mistake was made and how to fix it.

The only disadvantage is a few extra lines of writing.

Multiplication

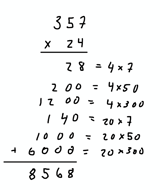
Again we reinforce place value. We also make the use of the distributive property explicit here.

We can verbalize our reasoning coherently: "I want 24 groups of 357 units. I start with 4 groups of 357 units. So I need 4 groups of 7, 4 groups of 50, and 4 groups of 300. Then ..."

It is also nice to pair this with an area model to see where the partial products come from geometrically.

Again we can check our work: instead of crossing things out and carrying we explicitly show each partial product. If we make a mistake on a single digit multiplication fact (like $4 \times 7 = 24$), or make a place value error (like $20 \times 50 = 100$), we can pinpoint exactly where the error was made and correct it.

Division

We can verbalize our reasoning coherently: "I have 937 units which I want to share equally with 4 groups. First I put 200 units in each group which uses 800 units, leaving me with 137 units remaining. Then I put 30 units in each group..."

Note that this reasoning continues to make sense even with decimal quotients! "I have 1 unit left. I break that unit into tenths, so I have ten tenths. I put 2 tenths of a unit in each group, leaving me with 2 tenths of a unit remaining..."

Again we can check our work and see if the mistake was with a division fact, a multiplication fact, or subtracting incorrectly.

Any use for multiple algorithms?

I do think that, if you have the time, it could be nice to very occasionaly give students the challenge of figuring out why an alternative algorithm works. The goal is not to teach them to use a new algorithm: it is to show that many methods are possible and that they are all based on reasoning.

One I like is the following alternative algorithm for subtraction:

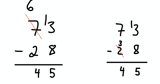
The standard algorithm is on the left, while the "novel" algorithm is on the right.

In the standard algorithm we think about breaking down one ten into ten ones so that we have "enough ones". This is an important idea!

You could challenge your students to explain the reasoning behind the second algorithm. Can you do some more subtraction problems this funny way? Do you think it will always work? Why? Is this method easier or harder for you to use? Are there any situations where choosing this algorithm might make it easier?

This algorithm relies on the principle that $A - B = (A + C) - (B + C)$ which is also an important idea: the difference is invariant under shifts.

For an interesting situation, consider $1000 - 1$ using the standard algorithm vs. this new algorithm. Of course, if you really understand our number system you should be able to compute the difference without an algorithm. However, it is instructive to compare the "multiple borrowing" in the standard algorithm to the relatively clean treatment with this alternative algorithm.

Answer 4

I am not a professional educator. I have, however, tutored students in math that needed to improve their grades. My personal observation in that context is that presenting alternative explanations or algorithms is confusing to students if they have not yet mastered one particular algorithm based on one particular set of explanations. For this reason I always consulted a students' textbook first to make sure I would be in line with it.

Assuming you are working with gifted and / or highly motivated students that are not overwhelmed or confused by the additional information, I think covering a wider array of algorithm options is possible within the realm of Euro-centric mathematics, which is the only mathematical tradition I am familiar with. I do not have a good overview of secondary literature that covers this well. It may or may not exist. Students might want to take note that having knowledge of French, German, Italian, and Latin is useful if one wants to consult historical mathematical literature.

When European countries switched from abacus-like computation (by manipulating tokens on lines drawn on a desk) to pen-and-paper calculation during the 16^th century, there was initially great diversity in the arithmetic algorithms used. This is covered in much detail in

P. Treutlein, Das Rechnen im 16. Jahrhundert. Leizpig: B.G. Teubner 1877

The following book for example recommended a subtraction methods that proceeds from most significant to least significant digit on p. 9, employing a one digit look-ahead:

Peter Ramus, Arithmetices Libri Duo, et Algebræ totidem, Frankfurt 1586

As another example, in the first printed work on algebra, the author presented a total of eight different methods of performing multiplication, many of which originated in India from what I understand.

Luca Pacioli, Summa de Arithmetica geometria, proportioni : et proportionalita, Venice 1494

The description of the multiplication algorithms is at pp. 26ff and includes the gelosia method as the sixth method, which is a lattice method named after the grids installed in front of the windows of high-ranking ladies so they could look out but not be seen from the street. The first method covered is the distributive method which is the standard method used in long-hand multiplication today. The crocetta method, which is based on the column-wise addition of partial products is one that is relevant to performing multiplications with computers.

The following influential French work starts with the current standard long-hand multiplication method but also provides multiple alternative algorithms, however there are fewer than in Pacioli's earlier work, and the gelosia method is not among them:

Larismetique & Geometrie de maistre Estienne de la Roche dict Ville Franche, Nouuellemente Imprimee & des faultes corrigee, Lyon 1538

If one looks at further developments during the 16^th century, it is obvious that there was a rapid convergence of arithmetic algorithms towards the standard long-hand methods taught today. This seems to be a clear indication that the alternative methods were considered impractical, cumbersome, or overly difficult. If one looks at a standard introduction to basic arithmetic from the late 1600s, the algorithms are as I learned them in elementary school in the early 1970s in a non-English speaking country, representing multiple centuries of continuity:

William Leybourn, Arithmetick, Vulgar, Decimal, Instrumental, Algebraical, 7th ed., London 1700

As for square roots, the standard long-hand method in use today (if it is taught at all, that is) was already the dominant method in the 16^th century, as for example in this book:

Die Coss Christoffs Rudolffs, Mit schönen Exempeln der Cosz. Durch Michael Stifel Gebessert vnd sehr gemehrt, Königsberg 1571

Treutlein notes, however, that in addition to this algorithm some authors also provided methods for the approximate computation of square roots.

Answer 5

I would like to challenge the view that knowing and using more than one method is necessarily harmful. I shall take subtraction as an example.

According to the Wikipedia page on subtraction there are two main methods here, the American and the Austrian. I remember confusion at secondary school when boys had been taught different methods at their primary school. While trying to clear this up the maths master remarked to me "There is a third way, Dewey, isn't there? The right way.". I answered yes as tactically the best answer. I was not challenged on what it was but there is indeed another way which I suspect I learned from my father and which I still use now for mental arithmetic alongside the other method for bigger numbers. For small numbers I use counting up. So to take 37 from 95 I would go 3 (to make 37 up to 40) 53 (to make 37 up to 90) 58. I suspect many people in fact use multiple methods depending on circumstances and I can see no real disadvantage as long as they have different areas of application.

If our schoolmasters had been unkind enough to make us do subtraction in the mixed radix systems we used for distance and weight at the time I suspect my method would have been better there. Of course it was used in a process for currency called giving change but that is obsolete now we have almost abandoned cash

Answer 6

My favorite alternate method is what one of my students called "adding by subtracting".

Taking a problem from above: 789 + 62

First, subtract 1 from 62 and add it to 789 to turn the problem into 790 + 61

Then, subtract 10 from 61 and add it to 790 to turn the problem into 800 + 51

Then it is hopefully easy to get 851.

You can also "subtract by adding":

789 - 62 = 789+8 - 62+8 = 797 - 70 = 727

Or:

789 - 62 = 789+1 - 62+1 = 790 - 63 = 730 - 3 = 727

Many of you might be thinking "neither of those is easier". Of course not, for you. A very small portion of my tutoring students have serious lightbulb moments when I mention other ways to think about adding and subtracting. Some brains are just different.

Another thing about these methods is they are more catered to doing the math in your head without paper. Again, some students find the writing of math to be the most onerous part and giving them some mental math techniques can really help make them hate math class a lot less.

---

This is a little more esoteric, but I like to divide through prime factorization. So... how about 489/48:

489 = 163 * 3 and 48 = 12 * 4 = 3 * 2^4 (not sure if 163 is prime but it's coprime with 2^4 of course)

So 489/48 = 163/16 = 10 3/16

Answer 7

In basic mathematical operations, alternative approaches can include mental math shortcuts, the use of different number bases, or applying algebraic manipulations. Strategies like estimation, breaking down problems into simpler steps, or leveraging patterns and properties offer alternative ways to approach addition, subtraction, multiplication, and division.