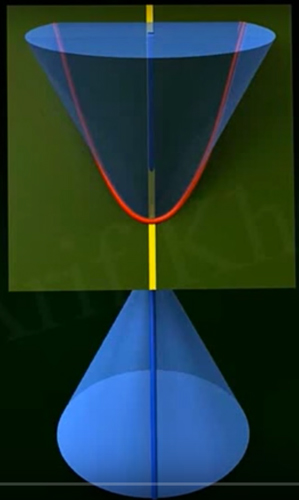
I want to present these three descriptions of a parabolic curve to my precalculus class:
The graph of a quadratic function $f(x) = ax^2+bx+c$.
Given a line called the directrix and a point called the focus, the set of all points in the plane that are equidistant to the directrix and focus.
The intersection of a cone with a plane that is parallel to a tangent-plane to the cone.
Showing students that the first and second descriptions actually give us the same curve isn't too bad: just draw a focus and directrix in the plane, take an arbitrary point that is equidistant to them, and show that the $y$-coordinate is quadratic in the $x$-coordinate.
But showing that these first two descriptions are the same as the third seems quite a bit harder. Does anyone know an intuitive way to show that the conic section description of a parabola gives us the same curve as the other two descriptions? I really want something brief but satisfying (so not necessarily thorough) so that my students don't just tune out. But every method I've seen so far has been a bit too involved to present to a precalculus class (like here), and I don't see an easy way to prune out some details of these methods.