Is evaluating a Real Polynomial at a Complex Value a suitable task for Precalculus students?

Question

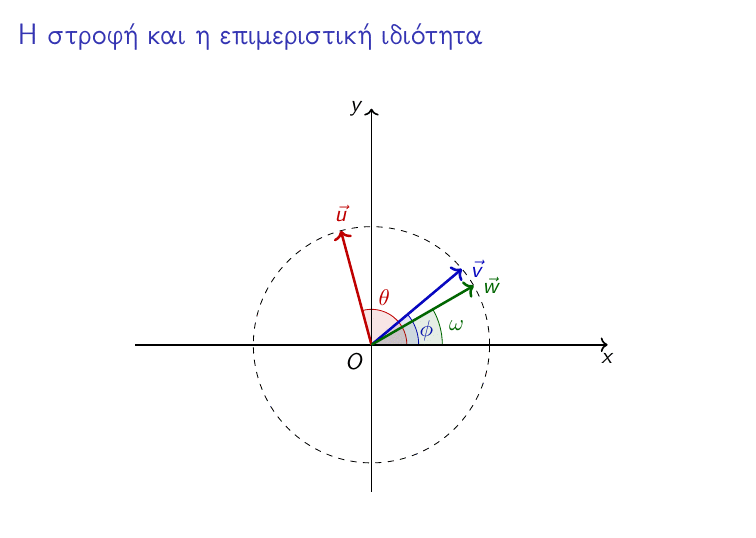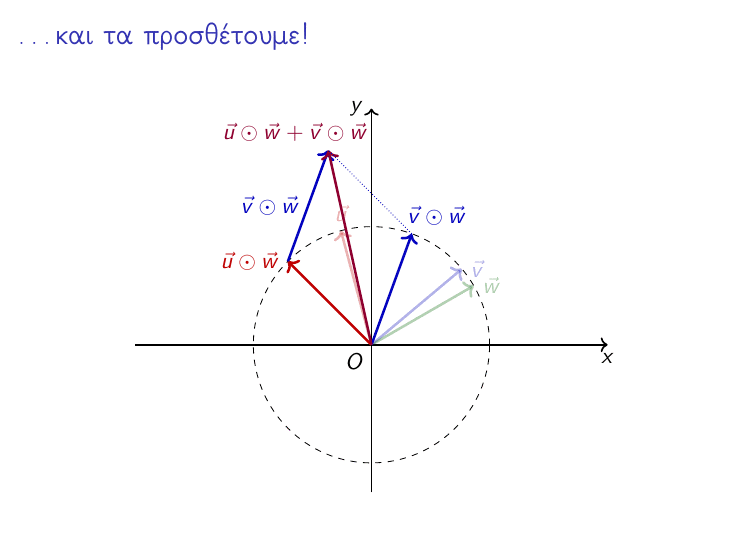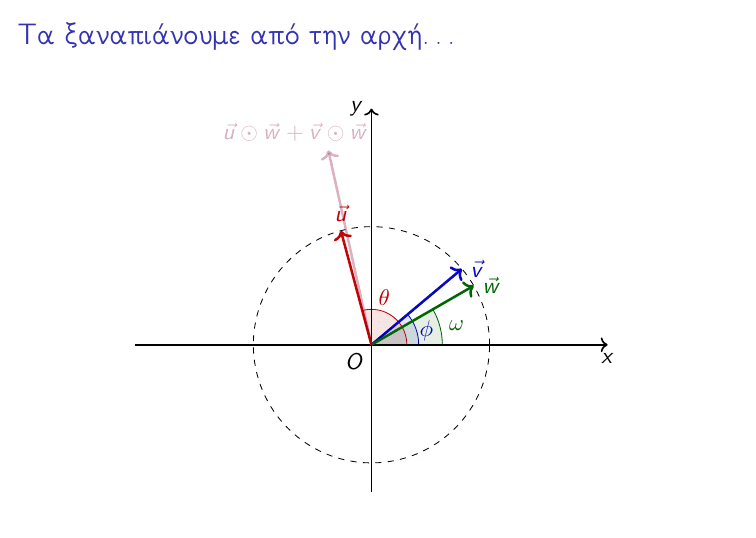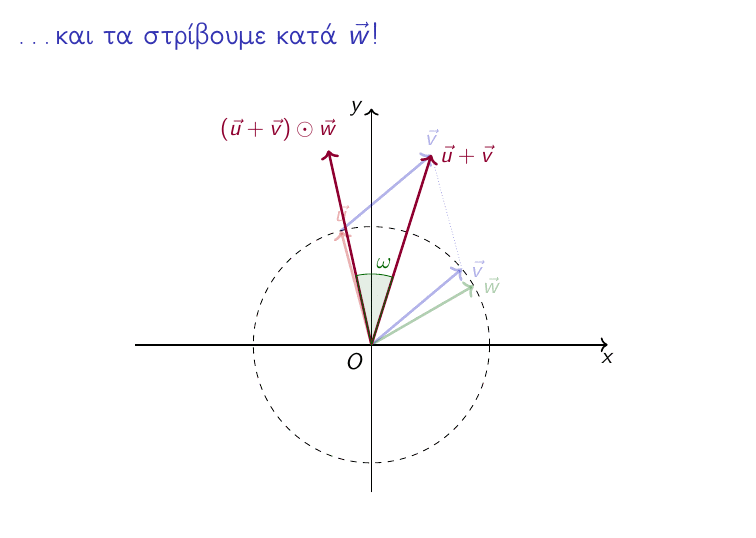
In Korea, basically every teaching material for 10th grade math(about the level of precalculus) contains this kind of exercises in their first treatment of complex numbers:

Evaluate $f(x)=4x^4-8x^3+3x^2+10$ at $x= \frac{3+\sqrt2 i}{2}$.

The standard answer goes like this: $x$ is a zero of the real polynomial $g(x)=4x^2-12x+1$, and dividing $f(x)$ by $g(x)$ we get $f(x)=g(x)(x^2+x+1)+x-1$. Plug in $x= \frac{3+\sqrt2 i}{2}$ and we get $\frac{1+\sqrt2 i}{2}$.

I have three reasons against having students at the level solve this type of problem. I would like to see what the community has to say about it.

1. It is more like an algebraic gymnastics than a useful tool for future learning. I don't see the students making use of this in any setting of math learning before they get into undergraduate level courses like Complex Analysis, and even then it won't be too late to pick up this kind of trick.
2. Going back and forth between (mostly rational) real coefficient polynomials and their complex values can blur students' vision of functional domains and codomains when they have absolutely no idea about complex functions.
3. Appropriating polynomial division to that end can mislead students to think of polynomials as something to break down to get to numerical 'answer', rather than mathematical objects on their own with proper operations.

I would like to add something about field extension, about how arbitrarily plugging in complex numbers to real(rational) polynomials could deter students' understanding later on, but that's probably too much when thinking about school math curriculum.

So, what do you think? Is it OK to keep this type of exercise in Precalculus level textbooks?

Answer 1

Personally, I agree with your viewpoints on the topic. Apart from what you mention, such excercises do not unveil the reasons behind the emergence of complex numbers - which, at first glance, are a quite counter-intuitive entity.

Personally, I would prefer an introduction based on a more historical context such as some cubic equations that need Tartaglia's formulae - i.e. "depressed" cubic equations - and then some equations that need Cardano's general formula. Thus, students have to manipulate complex numbers so as to arrive to even real solutions of the initial equation.

Another approach that I have followed in classes that have been taught about vector plane geometry is initiating a discussion about how one could, alongside the typical vector addition on the Cartesian plane, define a multiplication. After some discussion, we end up that multiplication seen as rotation is a suitable choice for such an extension. Then, roation is written, using some trigonometry, analytically in terms of the vector's coordinates. At this point, the notion of $i=\sqrt{-1}$ appears as a very useful convention so as to extend the already known properties of the reals to the new "complex" plane.

The above may sound too theoretical, but I do not invoke that much "hard-coding". Instead of verifying multiplication properties using trigonometry etc I prefer using some images like the ones below - which show how rotation satisfies the distributive law etc.

Answer 2

I think some algebraic gymnastics can be a good thing. They will need to keep track of such things when they do series (moderately in 10th grade and then in more detail for calculus and ODEs). Some "muscles" for this sort of thing are not bad.

In addition, I think the "concept lovers" make a mistake in not allowing for some approach to learning a topic first, more mechanically. Or at least alternately more mechanically. There is a saying about quantum mechanics, that you just get used to it over time, rather than thinking about it intuitively immediately. Certainly just lots of manipulation of i (sort of a strange quantity, philosophically) is a way of getting used to it. Just as very significant practice/exposure was needed in elementary school with negative numbers.

I do think if this is the very first problem, than that's not appropriate. (Start with definition of i and simple quadratics. But I doubt it is very first problem, since the students know what i is.) But within an overall chapter? It's OK.

In addition, nothing prevents later learning/thinking about i in different ways: phase angle, impedance versus resistance, etc. But it is nice to already be sort of familiar with our old friend i as just that thing that makes solutions of otherwise impossible quadratics (and other polynomials).