How to explain Monty Hall problem when they just don't get it

Question

Talking to some friends, I was asked to explain the answer to the Monty Hall problem (see also here;) .... they were having some trouble because whoever explained it to them didn't do a very good job.

_#Humblebrag I was able to explain it in under 30 seconds to most people (winning on staying is 1/3; winning on switching is 2/3 because it's based on losing the first pick, which is a 2/3); but some people just didn't understand....is there any sure-fire method of teaching the answer to people?

---

Note: This question is not being asked about a math class per se, but is probably equally applicable to the classroom setting as it is to a group of friends.

---

A lot of the new answers don't understand what this question is asking. Pay attention to the bold text above, and realize that I understand the solution to the problem, but had some trouble explaining it to some friends....the focus of this question is how to explain the answer, not what is the answer.
A question focusing just on the answer would probably fit in a lot better on Mathematics or MathOverflow; possibly Cross Validated (statistics).
Thanks! :)

After posting where to find other questions regarding directly to the problem itself, I found this question on Cross Validated -- it appears to be very, very related to my question.
(The letter copied in this answer to that question is hilarious, but very telling....)

Answer 1

Your explanation, by the way, is very elegant. As an experienced mathematician, I see immediately that it cuts right to the heart of the matter and admits no ambiguity. Unfortunately, this is precisely the quality that makes it unconvincing to others; the main confounding aspect of Monty Hall is that it ruthlessly exploits an intuitive misunderstanding of probability, and that misunderstanding remains even when one hears the correct explanation. People don't learn by being told what's true if that truth is in conflict with a previously more strongly believed falsehood; you have to directly confront and discredit their preconceptions before they will be able to appreciate the slick answer.

One possible misconception is that, after one door is eliminated and two remain, many people will feel that as an "either/or" choice, each door has probability of success 1/2, whereas in fact the distribution is not uniform. You might test the possibility of this being the problem by posing other, simpler questions about non-uniform probabilities, for instance: play a game where I flip a coin and give it to you if it comes up heads. What's the probability after two flips that you have 0, 1, or 2 coins? If they know the right answer, ask them to explain why, and if not, work it out with them.

(This can become Monty-Hall-like if you change the game once they understand. I flip both in secret and announce that there were "more than zero" heads, without saying exactly how many. Now what are the probabilities? This is very close to one of the branches of the actual Monty Hall problem)

Because of the coins setup where the "doors" are dynamically generated rather than static and the implication that the game is repeatable is more obvious, it may conform better to the intuition. The language a problem is stated in is very important for it being intuitive: people have different opinions about different but isomorphic versions of a single problem. I don't claim that everyone will like coins either.

I would avoid an explanation that rests upon the other person successfully adopting a mathematical mindset. For instance, the "100 doors" version immediately displays the problem but the concept of specializing from a generalization, or solving a related problem, especially one with extreme behavior, is not always natural to people.

Answer 2

For some reason, the 'extend it to 100 doors and eliminate 98' explanation doesn't make it any clearer for me.

Rather than talk about probabilities as fractions, I explain it this way:

"If you picked the car (without knowing it) on the first choice, you'll lose it by switching, whereas if you didn't pick the car, you'll gain it by switching."

(stop here and make sure they get that. Then...)

"Suppose you do the experiment 100 times*, and always stick with your initial choice. About 33 of those times, you picked the car on the first choice. So if you always stick, you'll end up with the car 33 times out of 100.

However, about 66 of the times, you didn't pick the car on the first choice, but if you switch, you'll switch to the car.

Therefore if you always switch, you'll get the car about 66 times out of 100, whereas if you always stick, you'll get the car about 33 times.

So it's twice as likely to get the car if you switch, as if you stick.

---

*or 99 if they're picky

Answer 3

There isn't any sure-fire method of explaining anything, and especially in math. But specifically in the case of the Monty Hall problem it has been proven by extensive experience that many individuals with otherwise above average intellectual capacities exhibit an exceptional tenacity in refusing to accept the (otherwise) widely agreed upon solution; don't waste your time on repeating this experience, unless you should find pleasure in doing so.

It should also be noted that for experts in probability theory there is a lot of nitpicking to be done about the exact hypotheses that need to be formulated in order to get a completely well defined probabilistic problem with the purported solution as correct answer (the Wikipedia page on the subject illustrates this). So if the resistance you get is of the type: "it is not so clear cut, it might depend", then very possibly they could actually be right, unless you took all the required precautions in explaining the problem.

Supposing you are trying to convince one person (otherwise it may become complicated) who has honest misunderstanding (as opposed to for instance a problem of pride in admitting to have been wrong) I could suggest the following approach. First make sure you have the same understanding of the problem formulation; otherwise any reasoning is pointless. Then if the other person agrees this should be a well defined probability problem with an unambiguous answer (again pointless if not) let him/her propose a method that should be able to determine that answer; it does not have to be efficient, but it should be acceptable independent of your beliefs about the answer. Since you are (convinced to be) right, you can afford to be generous; of course you should take care to refuse obviously incorrect models (like anything where the winning location is determined/changes after the game starts). Some people would agree to be convinced by an experiment/simulation, but not everybody; you cannot impose this method. (Also if you do agree to this, be sure to fix beforehand a number of trials sufficient for you to accept the risk of being accidentally "shown" wrong; asking for more trials after such an eventuality would make your credibility zero.) Another option would be to make a tree of all possibilities, with agreed upon probabilities for descending into each branch. (Many arguments start "without loss of generality let the first door be chosen". Don't do such a thing; while it can be justified, you cannot expect your adversary to accept the WLOG). Then compute the probabilities for each leaf, and add up. Or some other method still that could be proposed. Just make sure you agree about the method and its validity before applying it. Then just work it out, and accept the result.

Answer 4

Perhaps it's not the explanation that's the problem. I suggest you have them explain to you their understanding of the problem. Listening to their justification might reveal why your explanation is not gaining traction.

Even in the cases where people are saying they now agree with you, you don't necessarily know that they understand the problem. It's possible that all you did was get them to agree with you. People often just agree with another person who seems to have a better grasp of math.

However, if they explain it to you, you'll have a much better idea of their understanding. No matter whether they agreed or disagreed with your conclusions.

Answer 5

I've had best luck by simplifying the problem to asking whether you want to pick one door or two doors. Everyone understands they'd be better off picking two doors. At that point I tell them to just ignore the fact that Monty showed them what was behind one of the doors they didn't pick. They're still picking either one door or two doors.

Those more sophisticated, but still confused after listening, will object that this ignores the additional information gained from Monty opening one of the doors. But that's a red herring. There's always a losing door in the two you didn't pick, so Monty is not revealing any new information.

Answer 6

I found the most helpful way to think about the problem is to expand it to a larger number of doors. For example, if you have them select from 100 doors, where 99 are losers and 1 is a winner. Then after the initial selection is made, eliminate 98 doors.

The crux of this explanation is from one of Polya's heuristics, as explained below.

The initial answer remains above, as I feel it is a quick answer to the question, thought it might not satisfy everyone. I answered almost immediately after the question was posed, and in the time since this edit, many more thorough answers exist on the board that should satisfy such readers.

I do wish to provide an answer to the comment by @Tutor who asked "what Polya's heuristics are". George Polya is a well-renowned 20th century mathematician whose seminal work is How to Solve it. In it, he discusses his heuristics (techniques) for solving (perhaps difficult) problems. Under the principle of "Devise a Plan" he discusses the idea of solving a different problem if you are having difficulty solving the one posed. Sub-heuristics of this are: (1) solve a simpler problem, (2) solve a special problem, (3) solve a more general problem. The statement of a new problem with 100 doors could fall under any one of these categories.

Answer 7

I never understood it until my Maths lecturer explained it to me. Unfortunately I can't remember the exact way he explained it, but I'll try my best to remember. I really like your own explanation, so there is a chance that the following won't work. In that case, you may have to resort to empirical experiment. Another observation from my own experience is that sometimes I get too "mathsy" too quickly, and people don't follow my reasoning. Perhaps you need just need to slow down a bit!

Try this explanation. Get three cards to represent the doors. Use an ace as the winning door. Now, take two of the cards, and give one to your friend. There is a 2:3 chance that you have the ace, because you have two of the three cards, and there is a 1:3 chance that your friend has the ace, because he has one of the three cards. Now, peek under your cards, and discard one. Ask your friend if they want to swap for the card that you kept.

This is essentially identical to what happens in the problem, but it feels different because you are choosing two of the cards. You can highlight that you had a 2:3 chance of having the ace, because you had 2 of the cards. This may appeal to their intuition.

Answer 8

The best sure-fire method for teaching this to people who don't want to learn it is to set up a Monty Hall style game for small stakes of real money. When they start losing 2/3 of the time, they will become more receptive to your explanation.

Answer 9

While your explanation is correct, and easily understood by people like us, it's a bit too terse (a quality we like) for a lay person to understand. You can simply expand on it. And most importantly ask the audience questions along the way. Every explanatory sentence should have a question that goes a long with it. The key is for you to identify where the hangup is. I like to think about the problem in terms of the chosen door rather than the switching door. So this is how I might go about asking the questions.

1. Ask them before removing a door "what is the probability that the door they chose has the car?"

2. Then say, "I remove a door. Since I am not evil, I am not going to remove the door with the car." Then ask "Why does the probability that the car is behind the door you initially chose not change?" If they don't get this right, work out the cases, as others have mentioned (continuing to ask questions along the way).

3. Then say, "Since there is 1/3 probability that the car is behind your door, what must be the probability the car is behind the other door?"

Explanation at each stage may be required, but this socratic method seems to work well. I deployed something similar to a bunch of small children at the NY county fair. By making the argument composed only of leading questions, its a way of making sure they are following at every step. Of course you can ask them different questions. The key is to make it socratic.

Answer 10

I find that most people who THINK they understand the Monty Hall Problem, actually don't. For about 5 years I was one of them, until a further insight made me understand it better. More of that at the end.

However, I came up with a super-exaggerated version that seems to give people pause for thought at least.

In the UK, our lottery has a probability of 14,000,00:1 for the jackpot. Suppose I buy all 14,000,000 tickets one Saturday and let you choose 1 ticket with your favourite numbers, the rest I put in my shed. I lock you in a room until the draw with no access to the lottery result and I sit in my shed and watch the lottery draw. After the draw, I walk out of my shed with 1 ticket in my hand and set light to the entire shed and the rest of the tickets. I unlock the door of your room and announce, “Good news! One of us has the winning ticket! Would you like to swap with me?” Why would you think that your ticket has suddenly become a 50/50 chance of being the winner? If you believe that, imagine we did the very same thing NEXT week and the week after. Why has your lottery ticket suddenly acquired a 50/50 chance of winning every week – yet never wins it?

The crucial thing I alluded to at the top is that Monty HAS TO KNOW where the car is before any of the normal interpretations become valid. The “100 doors” scenario illustrates the point very well here. You pick door one and Monty opens door 2, then door 3; all the way to door 36. He bypasses door 37 and moves to 38, 39; all the way to 100. He then asks if you want to swap door one for door 37. Clearly the car is behind door 37 (well, 99 times out of a 100).

However, imagine you watch the program for 3 months before you are a participant and every week Monty keeps opening doors to find that the car is behind: door 17, door 81, door 53 – each week he keeps stumbling upon the car BEFORE the point he would give the contestant the chance to swap. When you go on the show, you choose door 1, then Monty proceeds to open each door from 2 to 99 and does not find the car. When he offers to swap door 100 with you, there really is a 50/50 chance that you have the car already and there is no advantage in swapping.

Answer 11

Speaking from my own experience, a stage I had to go through before understanding any explanations of the logic and math involved was indignation at being baffled by a cheap fairground trick that probably goes back centuries. Also the eventual recognition that what put me, the punter, at a disadvantage to the operator was the fact that s/he is working to a strategy and is therefore prepared to lose a game every so often (1 out of 3 in this case), whereas I'm limiting myself to only thinking I've got to win the present game each time.

Another motivational prepping step I'd recommend is a highly entertaining presentation (available on YouTube) by the great tele-mathematician Marcus du Sautoy playing the game with someone who sportingly agrees to be the fall guy who never switches and so loses heavily. This brings out the compelling practicalities.

A couple of points about the explanation stage. A clear diagram with your three doors and a list of the three possibilities of what's behind them: Car Goat Goat; Goat Car Goat; Goat Goat Car. If my first choice of door happens to be the correct one, and I switch, then sure as hell I'm going to lose on that occasion. Don't mince words about that, but use it to show how it means that if instead my first choice happens to be the wrong one, which is going to be 2 times in 3, then switching will reverse those odds into my favour.

(I agree with others that I've never understood how considering lots and lots of doors is helpful)

Answer 12

Here's my take on explaining it. (And it usually involves a drawing for me :-) )

Assuming the host opens a door the odds of making the right choice without switching the door is 1/3 because it was made before having the knowledge the host provided.

Once you decide to make the switch here is a breakdown of the probability of losing VS winning:

Answer 13

The big problem with the "Monty Hall" problem is that there are many problems that sound superficially the same, but have different solutions. The terms of the game have to be stated very precisely.

As an example, Marily vos Savant's statement of the problem as it is quoted in the Wikipedia article is imprecise. It doesn't state whether the game host must or may open another door, whether he must open a door with a goat or whether he is allowed to open a door with the car, and if he has some freedom of choice, whether he is trying to help you or not. Or whether he is even allowed to "cheat" and swap the contents of the two unopened doors sometimes.

So you listened to the explanation why changing doors is so good, but they just changed the rules - after you choose the first door, if you chose a goat the game host will open the door and say "tough luck, you lost". If you chose the door with a car, the game host will open another door with a goat, so if you swap, you lose. So you see that without stating the rules precisely, you can't give the correct answer.

The "100 doors" explanation doesn't help. So you are the lucky guy that by a one in hundred chance picked the car, and you are shown 98 doors with goats. If you swap, you lose. On the other hand, people who picked a goat will be shown fewer than 98 doors (once in a while, someone will be shown 98 doors, or the game would be given away).

Answer 14

The best way I came up with is to draw all 9 possible outcomes.
Prize is in A, you pick A;
Prize is in B, you pick A;
Prize is in C, you pick A;
Prize is in A, you pick B;
and so on.
Each outcome is equally probable and 6 of those will make you win.
I don't think it get's any easier than this.

Answer 15

The problem is most of the time something in the line of that a switch isn't believed to be helpful, because nothing has changed in the eyes of the person you are explaining the problem to: they consider the chances to be the same as before.

Explaining that something has changed can be the best step to take first: make sure they understand that they are getting more information about the system.

Ofcourse, then you go on, that because of this information, the chances have been changed. Then you can explain that the information is different based on the case you were right (1/3, no real new information) and the case you were wrong (2/3, new information is he tells you were the winner is).

Answer 16

Switching turns a winning guess into a losing guess, and a losing guess into a winning one. Since your guess is twice as likely to be losing as it is to be winning, you do better to switch.

Answer 17

Please warn me if my rewrite of this /r/eli5 comment doesn't answer your bolded question.

---

It helps to get rid of the standard trappings of the problem. The problem beneath is mathematically the same, but makes it clearer why you ought switch.

You are a superhero patrolling a crowded train station. A male stranger comes up to you, and asks you to pick a person at random, out of a crowd of thousands. We'll call your pick person A.

This stranger then tells you that he's part of a math-themed supervillain militant movement. He explains that one of the people in the crowd is their agent, who has a bomb that will blow up the city.

Seeing the worry in your eyes---and a total lack of mathematical thinking given the crisis—he says that they'll even up the odds a bit: he'll narrow all but two of the people in the crowd who may be carrying the bomb to person: A (the person you picked at random without even knowing what you were doing), and B (their agent). He guarantees that A or B has the bomb, which'll detonate in a few seconds.

In this case, who would you think has a better chance of carrying the bomb: your random pick (A) or his pick (B)? If you only had time to disarm one of them, would you go for A or B?

This ought clarify why you “switch” rather than just, say, flip a coin. The odds that the bomb is on A, are a truly random chance from the original cast of thousands. The odds that B has the bomb are obviously higher, since B MUST have the bomb if your original choice was wrong, and your original choice only had a $\dfrac{1}{thousands}$ chance of being correct.

Answer 18

Short and sweet summation of the method in the question:

It's more likely that your first choice will be wrong than it will be right. Therefore, if you are given the opportunity to switch, it's more likely that switching will be right than it will be wrong.

Answer 19

How about encapsulating the entirety of the explanation in a tree diagram, which is visual, accessible, and relevant to any Intro Probability classroom?

We drew this today while going over the Monty Hall problem to cap off the topic (Probability) by considering interesting / counter-intuitive problems like the Birthday Problem, medical tests and the Sally Clark case and using tree diagrams to tackle them.

Here, without loss of generality, the contestant has chosen Door $1.$

Extending the traditional probability tree by framing the contestant's winning decision as a third trial makes this a self-contained visual summary of the game (probability experiment).

P.S. On less mathy fronts, I find these two videos (Brady's, Lisa Goldberg's) both cogent, and the OP's own explanation cleverly succinct!

Answer 20

Once I set up a bet, using a bunch of (future) lotto drawing. Lotto in Italy uses 90 numbers, and traditionally those from 1 to 30 are marked 1, those from 31 to 60 are marked 2, nd those from 61 to 90 are marked X. I would have taken the role of Monty Hall, and I explained in advance the algorithm which I will use to show a result (among 1, 2, X) which did not appear.

Exposing the algorithm makes people think that Monty Hall cannot usually choose a random door (or a random value, in my example). At least for me, in this way is easier to understand that for the opponent too the choices are not casual.

Answer 21

Judea Pearl & Dana Mackenzie, in their new book The Book of Why (p.190ff), explain the paradox in a way I hadn't seen before.

Pearl imagines changing the rules to "Let's Fake a Deal," where "Monty opens one of the two doors you didn't choose, but his choice is completely at random." Of course he could open the door containing the car/prize, ruining the game. But his point is that, with this random choice, there is no longer any advantage to switching. If Monty opens a door containing a goat, there is a $1$-in-$2$ probability of winning either by sticking or by switching.

That Monty's door-opening is not random, but rather constrained by Monty's need to avoid revealing the car, now makes it more clear that significant information is conveyed by his goat door-opening.

He assumes you open Door $1$, and then:

---

         
          ^{Let's Make a Deal, vs. Let's Fake a Deal.}

---

Answer 22

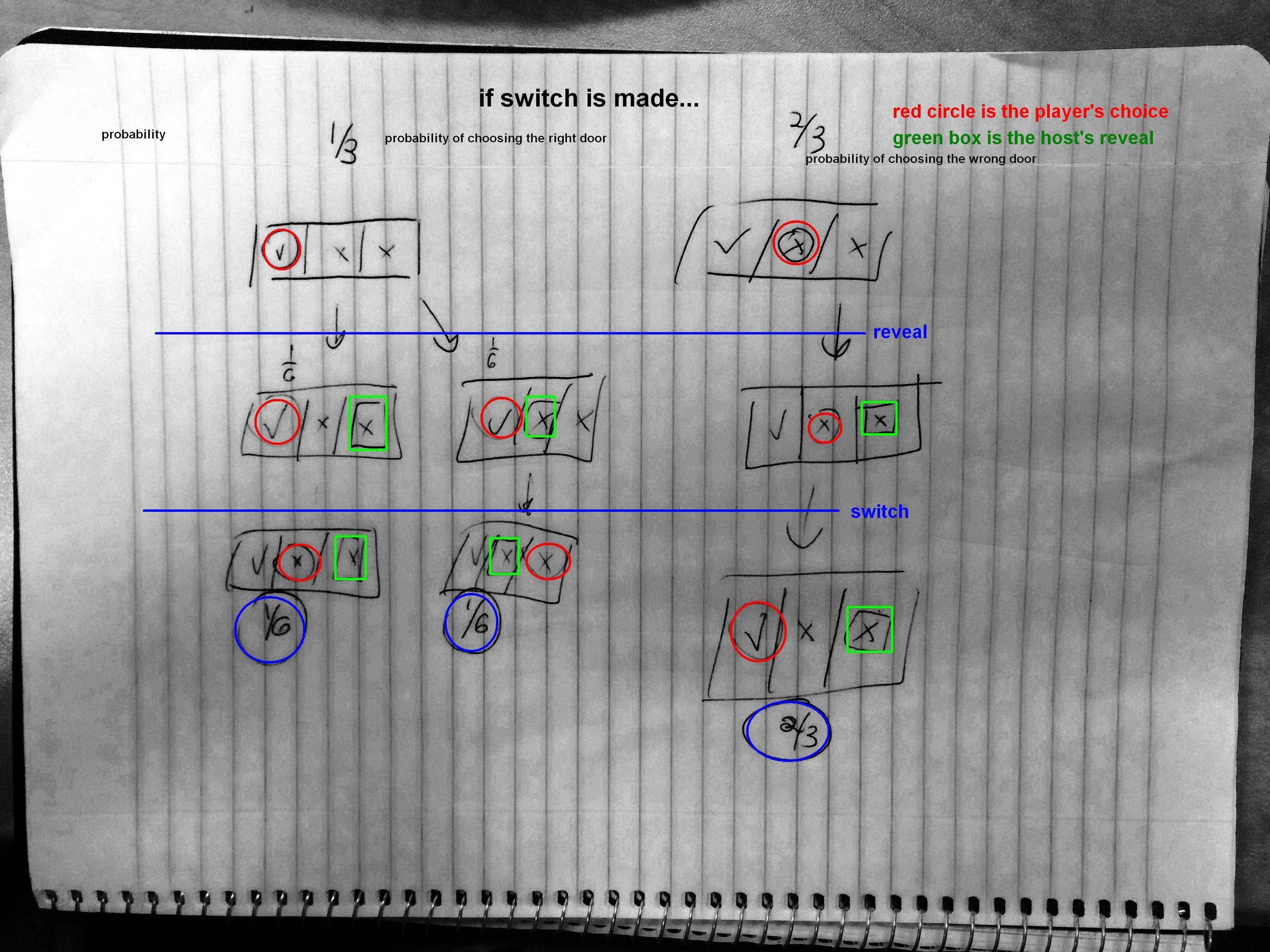
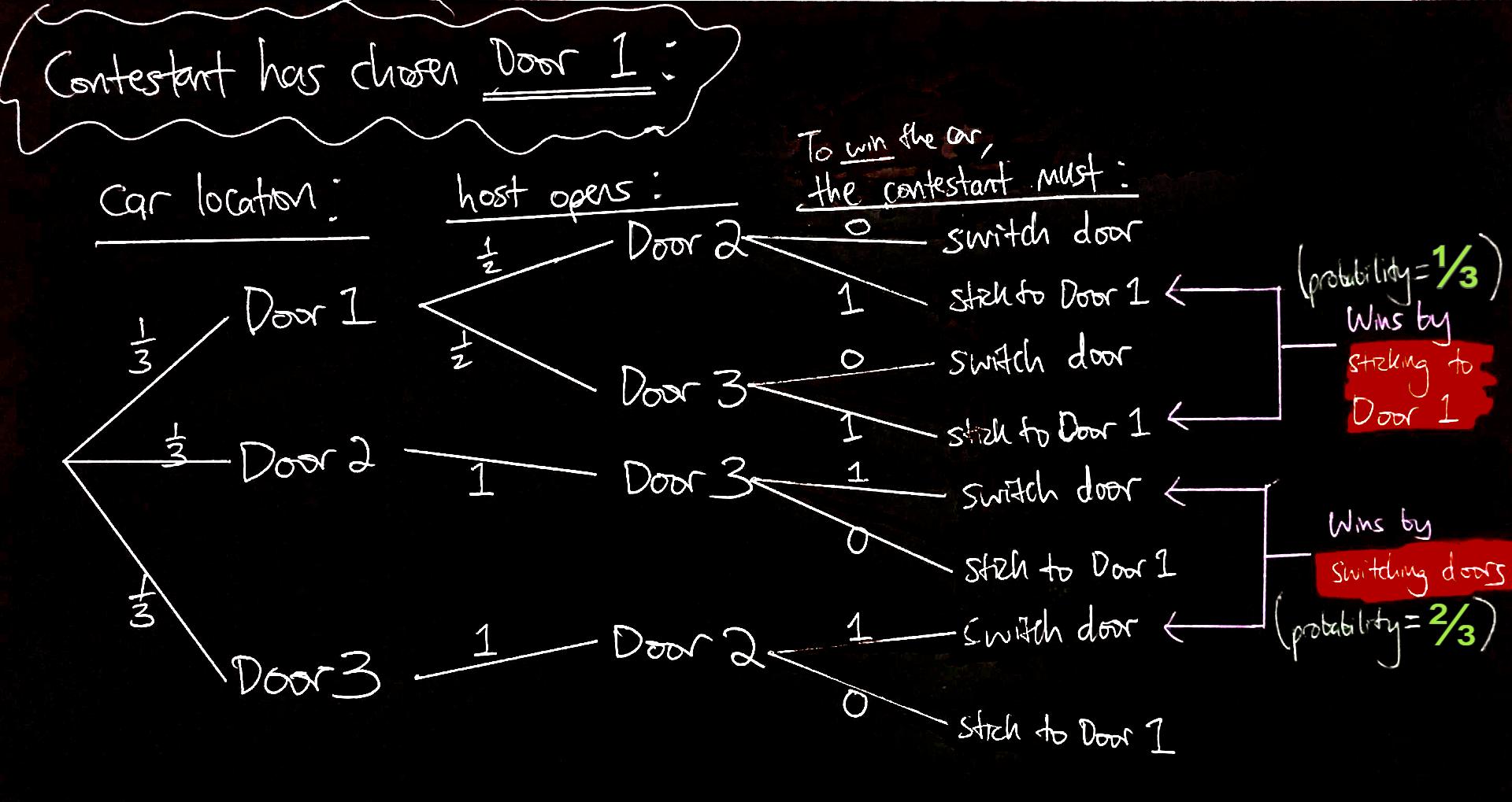
I usually explain this by simulating, and then defusing, the mis-understanding using a simple table. You can set up any of the three cases of the problem, as they are obviously all the same, I'll use [1:Goat] [2:Goat] [3:Car] here:

My choice        Monty Shows       Reward for Stick     Reward for Switch
 1 (goat)          2 (goat)               0                     1 
 2 (goat)          1 (goat)               0                     1 
 3 (car)           1 (goat)               1                     0 
 3 (car)           2 (goat)               1                     0 

Point out to the student that that in the case where I chose 3, Monty can open either door number 1 or number 2, but in the other case, he has only one choice. However, so far all that we've done is to replicate the confusion because if you count up the stick vs switch rewards, it's 50/50 (2/2).

The student will be, like, "See!" (and this is, indeed, the confusion that even many mathematicians who wrote into MVS's original column had!)

But let's look at the probabilities of each row. Recall that the total has to be 1. In the first column, start by assigning 1/3rd to each row. But that's not right, because it adds to 4/3rds. Okay, maybe 1/4 each? But that makes no sense because where does the 4 come from; there are only 3 doors!? The only obvious remaining assignment is 1/3rd, 1/3rd, and then there's 1/3rd that the personal will choose the car -- that works out, so obviously we need to split this, so it's 1/6th for each of the last two rows.

Aha! So there's an actual traceable imbalance in the rewards! Each of the +1 for switching rewards has 1/3rd, so you get 2/3rds for switching, but the +1s for sticking together only add up to 1/3rd (1/6th each)!

There are two advantages to this approach. First, the table is obvious (more obvious to most people than a tree). Second, you start by replicating the learner's understanding, then point out the mis-understanding, and lead them, using the same representation, to understanding. I find that I generally don't have to go father than pointing out the 1/6th for the latter two rows, and they figure it out themselves.

(I want to make a comment about another common approach to explaining this, which I actually think is not a great way to do it. Specifically,, sometimes explainers will say something like: imagine that there were 100 boxes, 99 with goats, and when you choose one, Monty reveals 98 of them. There's several reasons why, although correct, isn't a great way to explain the problem. The main issue is that it doesn't map uniquely to the 3-door case; it's not clear, when Monty is revealing the 98 other goats, that he couldn't, at that point, have just revealed them of them, or one-at-a-time, which is an equally natural analog to the 3-door case, and is very confusing because you'd have to mentally run the simulation backwards to the 3-door case before being able to answer! That is, although the explanation is correct, it's not obvious that the correct analog of the 3-door case is to reveal 98, as opposed to 1, wrong doors.)

Answer 23

Originally, the chances are 1/3 that the car is behind Door 1, 1/3 that the car is behind Door 2, and 1/3 that the car is behind Door three. If you choose Door 1, your chances of winning are now 1/3. More to the point, the chances are 2/3 that the car is behind Doors 2-and-3 together. This fact will hold true for the rest of the problem.

Monte Hall opens Door 3 and shows a goat, meaning that there are ZERO chances of the car behind Door 3. But the chances are still 2/3 that the car is behind Doors 2-and-3 together. What's left of this "together?" Door 2. ALL of these chances are now behind Door 2, which "inherited" Door 3's chances. So the increase in your chances of winning by "switching," to Door 2, are 2/3 to 1/3, or 2 to 1.

Another example: Suppose there was an election with Candidates A, B, and C, each with 10 votes. Suppose candidate C (Door 3) drops out and says, don't vote for me, vote for B over A (and his supporters follow him faithfully so he gets zero votes). What happens next? Candidate B gets candidate C's ten votes plus his own ten for 20, versus ten votes for candidate A.

Answer 24

TL;DR There are still 3 doors even though one of them has been opened.

Fact 1. After your initial choice there is a 2/3 chance that the prize is behind one of the other two doors. Your chosen door has a 1/3 chance.

Fact 2. When the empty door is shown to you, there is still a 2/3 chance that the prize is behind one of those two doors.

Fact 3. Except now you know that one of those two doors is empty, so you choose the door that was not shown. That one door has a 2/3 chance that the prize is behind it.

Conclusion: The unknown door has a 2/3 chance of having the prize, but your chosen door only has a 1/3 chance. So always switch.

The mistake that most people make is thinking that it is a now a two door problem and therefore has a 50-50 chance. It is still a 3 door problem and can be easily solved with basic logic.